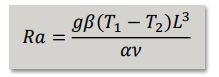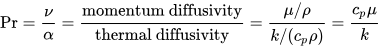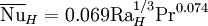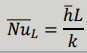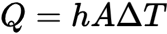How to calculate internal convection loss in a sealed container?

Question

Say I have a box with a 1m x 1m x 1m interior wall dimension.

The side walls and the floor are 10cm of styrofoam, with a thermal conductivity of 0.03 W/m K.

The top is 1cm thick opaque plastic with thermal conductivity 2 W/m K.

The box is fully sealed; no air can get in or out.

Across the entire floor of the inside of the box, there's a thin heating element at a constant 100ºC. The box is placed outside, where the air outside is a constant 20ºC.

Considering the bottom interior of the box, we have the following losses:

• conduction through the styrofoam downwards
• conduction through the air upwards
• conduction towards the sides

These are easy to calculate and I estimate will be <100W altogether. For example, the gradient of 80ºC between inside and outside bottom give 0.03/.1*80 = 24W conductive loss downwards.

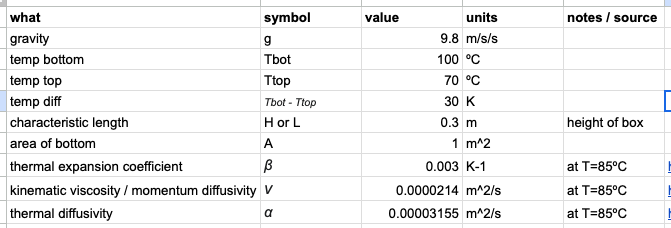
But, we also have convective heat loss: the warm air at the bottom will rise to the top of the box. It will be stopped by the plastic lid, but the higher thermal conduction means the heat loss will be faster there. The air will cool, and warm air from below will rise to replace it.

How would one calculate this additional convective loss within the box and compute the steady-state temperature of the lid?

Answer 1

This is a problem of free or natural convection in a horizontally-heated rectangular cavity.

If we know the temperature of the top, say 70ºC, the procedure is:

Calculate the Rayleigh number

(source)

With the following values:

We get: 3.527e7 .

Calculate the Prandtl number

(source)

We get: 0.678 .

Estimate the Nusselt number

This equation is valid for Rayleigh numbers between 3e5 and 7e9 (source):

We get: 21.99 .

Calculate the convective transfer coefficient

(source)

Therefore $h = Nu \frac{k}{L}$.

The specific answer here is: 1.91 .

Calculate the convective heat loss

This is now trivial:

Answer: 57 W.

You can repeat for any similar scenario.

Deriving the Lid Temperature

Without knowing the lid temperature, you have to set up the heat transfer equations and solve for the steady-state, re-calculating the relevant numbers at each step. This exercise is left to the reader.