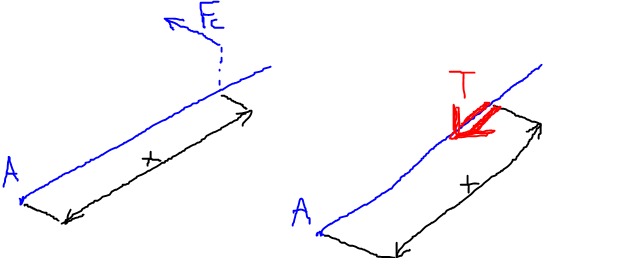
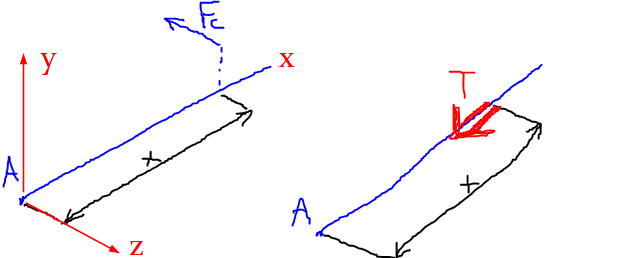
In both cases in the picture above torque is applied to the shaft, however in the first case the force $F_{c}$ also produces bending across the shaft. So my question is, how do I know if a torque should be shown with a force like in the first example, or with a torque vector like in the second example?
My best guess is that it depends on what produces the torque. Some ways do produce bending (like conic friction plates for example), while some don't.
Axial torque will never produce bending, just twisting. To get bending you need moments about the directions perpendicular to the shaft/beam.
In your first figure the force $Fc$ results in a moment along the vertical direction $y$ because the force is offset in the $x$ direction, as well as a moment along the $z$ direction because the force is offset in the $y$ direction.
If you apply a force or several forces with the resultant vector offset from axis of a beam or shaft as in you first case it causes moment and torque and transversal shear and axial stress if it is not perpendicular to the axis of shaft.
But if the forces resultant are such that they purely resolve to torque such as 4 equal forces acting around a circle perpendicular to the shaft equally at each 90 degree quadrant it will be just torque.
