Wind load of panel

Question

I am planning a prop for a marching band show and I want to determine how to best address the challenge of the wind blowing it over. This is definitely not my area of study and I am hoping for some guidance here.

The panel is 10' high and 4' wide. My working assumption is that we won't be dealing with winds any stronger than 15 mph. That may be wishful thinking but I needed to throw out a number.

The panel will be mounted on a small platform (5' x 3.5') and angled at roughly 80 degrees. Something like this:

The panel will be mounted top and bottom to the support and base.

Trying to get an idea about how much weight it would take to keep it from flipping over, I found a generic formula for calculating wind load:

F=AxPxCd

Using this formula and plugging in 0.00256xV^2 for P 0.00256 x 15^2 = 0.576

And based on some very cursory reading, I am guessing at the Cd value for a rectangular panel, setting it to 2.0.

I arrive at F = 40ft^2 x 0.576 psf x 2.0 = 46.08 pounds

But I'm not sure what this is telling me or if it is even correct for my situation. I'm primarily concerned with the wind coming from that support side and leading to it lifting or flipping the panel. I'm limited on the footprint but could add weights to the base and/or behind the base attached to the support poles and/or the base.

Thoughts?

Answer 1

In US there are zones of maximum wind velocity and its pressure.

Majority of the zones assume (15 to 35) lbs/sqr ft pressure for heights of up to 60 feet, depending on combination of factors such as zone, exposure, utility of structure. Lets use just for illustration pick wind pressure of 20 #/sf.

We will have an overturning momentum of $$ m_{overturning} = (20\times 4 \times 10)_{total\space p} \times (10/2)_{arm} = 4000 \space lbsft \space moment$$

We need to counter this by adding enough width and weight to the base to resist this.

As a first guess say we pick the base to be 3 feet deep. then 4000/3= 1660lbs so you need a ballast of 2\times 1660 =3300 lbs at the center of base, which is not practical.

We add the depth to 5 feet and get the $ballast= 4000/2.5 = 2 \times 800 lbs.$

I think there are reduction factores for temporary uses such as yours but this is just to get a feeling of the magnitude and order of wind pressure.

Answer 2

So, I am not a professional engineer, but: that 46 lbs. is a horizontal, equivalent force at the center of the panel. To figure out how much weight you need to keep it from tipping over, you will need to use statics (think levers). Put in a factor of safety of, say 2 -> you need to be able to balance a 100lb force acting on the middle of the panel (5' from the ground).

5' x 100 lb = 500 ft-lb of torque. Looking at your base, it looks like you have a lever of about 3 ft in length. Assuming you have the weight evenly distributed on the base (this will provide the same resistance to tipping in both directions), your weight needs to act on a 1 1/2 ft lever arm and provide that 500 ft-lb of torque. This translates to about 340 lbs.

You will also need to make sure that the method you use to secure the panel to the base can withstand transmitting that force: if the sign just rips off, it doesn't matter how much the base weighs.

Also make sure that the sign doesn't deflect too much when the wind is blowing: imagine some suitable person sitting in the middle of the sign when it is laid flat on some sawhorses. If werf ends up on the ground, you need to improve your design.

Moving the brace to connect to the sign 2/3rds of the way up could help.

Answer 3

I am not a qualified civil engineer. This advice is theoretical and not to be used for any actual design in the real world. It is for informational purposes only.

In civil engineering we always design things for worst case scenarios for good reason. Were that panel to fall over and injure a child as happened with a Hugo Boss store in Bicester (pronounced Bister) Village, England in 2015, there may be a criminal case to answer and also legal issues that cost vast sums of money. No design of any structure is taken on without a full assessment of its possible environmental impact which means how that structure is going to perform in its position and the effects it might have in failure situations.

To formuate any coherent design we must make educated assumptions that take into account safety factors, environmental factors, mitigation of loading extremeties, alternative design issues to overcome any unforseen issues.

• In this case we need to assume that the maximum wind velocity is not only possible, but is likely and then use something like the Eurocodes safety factor margins to improve the safety of the structure.
• The panel must be able to withstand a unifomly distributed load from wind at a far higher load than 15 mph for good reason: Wind has a tendency to NOT be uniform and GUSTS may easily be up to 80% times higher than median velocity. This means that 15 mph is possibly gusted up to 27 mph.
• A safety factor must be incorporated to withstand the moment of lateral forces and usually it is an additional 5 to 30% additional force tolerance.
• Altitude also plays a major factor in designing for wind load. Although wind is a dynamic load, all building structures are usually designed for a uniform load on account of safety and for simplified material strength calculations. The higher the board above ground level, the greater will be the unrestricted wind verlocity. There is often a vast difference in wind velocity between 4' and 40' depending on surrounding environmental fators.
• Depending on the location of the panel, the force of the wind may be substantially greater in certain sitings such as between buildings 2' to 12' above the ground compared to open area of a playing field.
• Aerodynamics also must be accounted for since it is flat and to be used specifically as an aerofoil or fro want of a better description, a wing.
• There is no indication as to whether this wing is going to be fixed or in motion on a float in a parade.
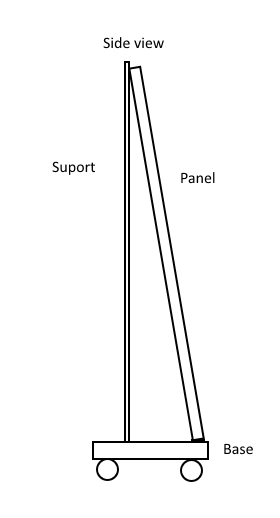
• According to the diagram provided, it is not going to be pinned (fixed) at all. It has two rollers meaning it can be moved mechanically. If this is true, then we need to consider using cable stays for a fixed device an mass-moment reaction balances for motion designs.
• There is no indication as to what the material is to be used because we do not know the magnitude of forces that may be exerted upon the wing for now.
• Self-weight of the entire system must be accounted for to calculate accurately what is a safe working design.

---

Calculations

Imperial (Metric) vertical Panel Dimensions $$ 10'(3.048m) \times 4'(1.2192m) =40'^2(3.7161216m^2) $$

Apparent inclined panel dimensions: $$ 10'\sin 80^\circ \times 4' \approx 39.39231012 \text{ ft}^2 \text{ or } 3.659665363 \text{ m}^2 $$

Wind Velocity:

Client estimated maximum velocity: 15 mph

Feasible velocity: $30 \text{mph}$ with gust velocity estimate: $\approx <60\%$ $$ 30 \text{mph}+(30 \times 6)=48 \text{mph} $$

Safety factor: 25% $$ 48 \text{mph}\times 1.25 = 60 mph $$

So now we have a design velocity for the wind force of 48 mph for which another website has calculations we can use to determine pressure and therfore a lateral force measure that may be exerted on the panel with a safety margin for error of 60 mph.

The problem is that air density differs according to its thermal state which means we also need to account for the temperature of the air as more dense air has greater inertia and hence a greater pressure against the panel. Wind pressure is proportional to air density multiplied by the square of the velocity:

$$ \rho = \frac{p}{R_{specific} \times K} $$

$ R_{specific}=287.058 J\cdot(kg·K)^{-1} \text { or } 53.35 ft·lbf(lb·°R)^{-1} $

When taking account of density, it is unlikely that anyone will sensibly march their band in temperatures below $5^\circ$C ($41^\circ$F) for which the density of air at sea level is going to be $ \rho=1.269 kg.m^3@100$KPa.

We know that our surface area is $3.659665363 m $^2$ and therefore the wind force will calculates as 1671N according to Engineering Toolbox

You can see from the result that that board would have to withstand a dead load of 170.4 Kg.f or 375.66 lb.f at the top vertical edge of the panel.

---

Design Brief and Recommendations

We can use a few different ways to prevent the board being blown over as enumerated below:

1. Deadweight welded to frame attached platform base;
2. Cable type stays like tent rope & peg systems;
3. Ground spike systems used to hold down groundsheets;
4. Equilateral triangle systems with internal deadweight.

Looking at your design, a single sheet of wood would theoretically be strong enough as long as it was able to withstand a minimum weight of 9.536 lb.ft$^2$

Considering that it is only a wood sheet, you might want to consider using voile over a framed support as an alternative or canvas with eyes. These options are to save on the weight of the device and work as a windbreak also. This is an important considerations since your wooden support's bending moments are going to require a great mass in a small area to prevent the system from toppling over.

If you consider that the total lateral force is effectively applied as a combined moment of force at the top edge of the panel in a lateral direction, you will require an equal but opposing force to counter that force in two opposing directions. This is a major concern because wind arrives from 540$^\circ$ of direction at ground level. To understand this, you need to consider a circular direction in plan and add 180$^\circ$ in elevation which is effectively an hemisphere of wind action towards the centroid mass of the force system which needs to have a very low centre of gravity.

A centroid is where the centre of mass combined with gravitation and wind inertia is situated. An example is that the entire construction is going to be in motion so we cannot use cable stays that are not also mobile in tandem with the panel and supporting structure.

Ideally, if the platform is going to be transported, use of ratchet straps that pull the panel downwards against the base of the support would be a good idea. Four straps of one inch width that wrap around the panel should hold it in position as long as the base and the apex are securely fixed to each other. In reference to previous ideas:

1. Deadweight welded frame and base While this is probably not a bad idea if transporting heavy loads above human carriage is not an issue, it requires financial expenditure if you are not a welder and do not have an encapsulating frame. On cost efficients grounds this may have to be a forgone conclusion and obsolete.

2. Cable type stays Feasible if using a vehicle like a pickup but will require some lateral moment opposition of a wider base.

3. Ground spike system This is clearly not going to help at all. Dropped into File 13 for trash.

4. Equilateral triangle system with deadweight. This is a modification of option 1 but with a design change to your initial proposal in the next section because frankly, my recomendation.

---

Design considerations

Moments of force

The basic formula for calculating moments around a point is $M_O = Fd$ where F is the total force multiplied by the combined distance from the point of rotation. Since the greatest force is accumulated at the top of the system, we combine the uniformly distributed load into a single point of force at the maximum point of rotation to calculate the rotation forces that need to be opposed.

$ M_O = 1671 \times 3.048 $

$ M_O = 5093.208 N.m \text { (1144 lbf)} $

The platform base should be cut into 4 lengths of equal width. Your proposed vertical panel is going to become the new platfrom base. Your vertical support will be supported by the 4 platform pieces as diagonals attached to the new base and upright support at angles of $60^\circ$ to the horizontal and $90^\circ$ to each other being pinned to the vertical support edge.

What we need to ensure is that the wind pressure does not blow the system over and the height is the issue because The force at the top edge multiplied by the height of the system will explain what magnitude of downward force we need to use to compensate for the turning moment. Fortunately, this design is quite simple and we can compensate for lifting forces whilst remembering that the design load is for 48 mph winds as a maximum and the extra 14 mph safety is to prevent anyone being hurt by the construction.