1
$\begingroup$

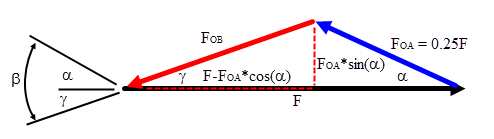
If the force in member OA is 0.25F and inclined at $\alpha$ degree from the horizontal. Calculate the angle $\beta$ to keep the system in equilibrium and calculate the angle $\omega$. $\alpha$ = 20°. F = 100 N.

enter image description here

Attempt:

By alternate interior angles:

poorly drawn alterior angles

$\beta - \alpha$ is the angle between OB line and horizontal axis x.

Sum of forces in X direction should be equal to 0:

$$\sum F_{x} = -0.25F \cdot \cos(\alpha) -F_{OB} \cdot \cos(\beta - \alpha) + 100N$$

$$\sum F_{y} = 0.25F \cdot \sin(\alpha) - F_{OB} \cdot \sin(\beta - \alpha)= 0 $$

is this correct? I'm not sure what $F_{OB}$, it's not given. So I have two equations, and two unknowns: $F_{OB}$ and $\beta$. I tried to solve via wolframalpha but it didn't find any solutions. What do?

Improve this question
$\endgroup$
4
  • $\begingroup$ I can remember that $\sin (A+B) = \sin A \cos B + \cos A \sin B$. and $\cos (A+B) = \cos a \cos B - \sin A \sin B$ $\endgroup$
    – Jem Eripol
    Commented Oct 9, 2017 at 0:38
  • $\begingroup$ This looks more like a trigonometric problem than mechanics problem. LOL. Identities are needed. I already forgot identities but here's my equations: $$0.25\cos{\beta}=\cos (\beta - \alpha)$$ $$\frac{0.25 \sin \alpha \cos \beta}{\sin (\beta - \alpha)}=\cos {\alpha}$$ Now, you only need to solve for $\beta$ and $\alpha$. The above equations can be simplified further into: $$\tan (\beta - \alpha) = \cot \alpha$$ $\endgroup$
    – Jem Eripol
    Commented Oct 9, 2017 at 0:42
  • $\begingroup$ I found out that $\beta = 2(\alpha)$ $\endgroup$
    – Jem Eripol
    Commented Oct 10, 2017 at 1:10
  • $\begingroup$ Seems something is wrong in the question because cos(20) is 0.93969 not 0.25. or maybe you can correct me? $\endgroup$
    – kamran
    Commented Dec 2, 2018 at 23:04

3 Answers 3

Reset to default
1
$\begingroup$

What do you do? You either use different software to solve the two equations, two unknowns (because there is definitely an answer), or you use a different technique to solve the problem. In this case, a different technique looks to be easier.

Your equations for the sum of forces in X and Y at point O are equivalent to this force diagram (which is not to scale):

enter image description here

The two components of the force $F_{OB}$ can be determined from this diagram:

enter image description here

from which you can determine these answers after substituting the value of $F_{OA}$: $$ F_{OB}=0.7698F=77.0N$$ $$ \gamma=6.78^o$$ $$ \beta=\gamma+\alpha = 26.78^o$$ $$ \omega=180-(90-\alpha)-\beta = 43.22^o$$

Improve this answer
$\endgroup$
0
$\begingroup$

Since the combined force (F) is 100 and 25% is in member OA, the balance must therefore be 75% in member OB.

In both member you need to convert the diagonal forces into vertical and horizontal components that provide the resultant sigma of forces. Since you have at last one angle and the final force (f), it is therefore possible to find all the other angles and forces, too.

Since alpha is 20°, the internal angle at A should be 90°-20° since we created a right angle triangle by separating the members into 2 force diagrams

Angle A=70°

Now you need to find the distances for right angled triangle AO $\alpha$

Improve this answer
$\endgroup$
0
$\begingroup$

$ 100- 25cos (20) = 76.5076 $

Force in member ob.

We divide the tributory of 75 N by this to get the cos(b-a) 75/ 76.5076 = 0.98029

Arccos (0.98029)= 11.3934

This added to 20 is angle beta.

Beta = 20 + 11.3934 = 31.3934

And Omega = 180- 31.3934= 148.606

Sum of angle in a triangle =180° Omega=180-(70+31.394)

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.