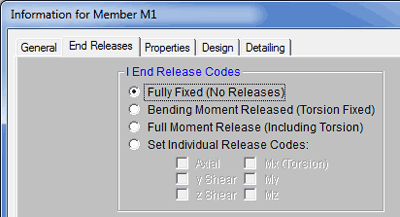
Beam End Release and Moment End Release are the same thing: it can be thought as converting a fixed beam end to a pivoted one (see later), relaxing the constraint on rotation while enforcing a new constraint on bending moment. The idea of applying constraints to beam ends is useful in understanding the mathematical implication in changing a fixed beam end to a pivoted one, and is discussed below.
Any continuous element of a beam, for small deflections, is governed by the following differential equation:
$$\frac{d^2}{dx^2}\left( E(x) I(x) \frac{d^2 u}{dx^2}\right) + q(x) = 0$$
Where $E(x)$ is the Young's Modulus of the beam, $I(x)$ is the Second Moment of Area about the axis of bending for the beam's cross section, $u(x)$ is the upward displacement of the beam, and $q(x)$ is the downward force per unit length acting on the beam. $x$ is a coordinate such that, for a beam of length $L$, $x=0$ at one end, and $x=L$ at the other end.
This equation is a forth order differential equation, and so it requires four boundary equations. This is done by applying two constraints at each end. There are three different types of beam end, each with different constraints to be applied:
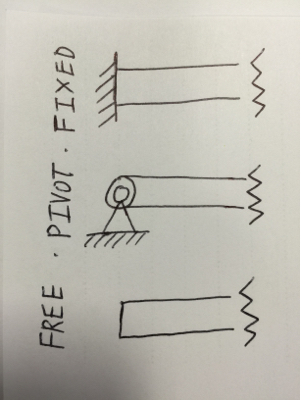
FIXED END
This is where the end of the beam is rigidly clamped to e.g. a wall. This end allows both shear forces and bending moments to be transmitted from the beam to the wall. The end of the beam here cannot rotate nor vertically displace. This is like the beam ends shown in the first diagram of your question before releasing either end.
If the end at $x=0$ was a fixed end, the following two constraints are applied:
No vertical displacement
$u(0) = 0$
No rotation
$\theta(0) = \frac{du(0)}{dx} = 0$
PIVOTED END
This is your moment-released end: the beam still may not vertical displace at the end, but it can rotate. However, since it may rotate, the beam can no longer transmit bending moments to the support, so a bending moment of zero must be set at the end. Hence moment-released.
If the end at $x=0$ was pivoted, the following constraints are applied:
No vertical displacement
$u(0) = 0$
No bending moment
$M(0) = 0$
Where $M(x)=-E(x)I(x) \frac{d^2 u}{dx^2}$
FREE END
This type of beam end is connected to nothing: it is free to displace and rotate at the ends. However, no bending moments or shear forces can be transmitted from the end since there is nothing attached.
If the end at $x=0$ is free, the following constraints are applied:
No bending moment
$M(0) = 0$
No shear force
$S(0) =0$
Where $S(x) = -\frac{d}{dx} \left(E(x)I(x) \frac{d^2 u}{dx^2} \right)$
Note that a cantilever is a beam with one fixed end and one free end, and a simply supported beam has both ends pivoted.
Upon setting two constraints to each end, the general solution to the differential equation can be obtained, allowing the vertical displacement, rotation, curvature, bending moments and shear forces to be determined.