I have a DC motor with a shaft with a diameter of x. It would produce some torque T because torque is proportional to radius. If I stuck a pulley on the shaft of radius 2x, then I would produce twice as much torque, right? And then if I attach this to a gear with a ratio of 2:1, I would again double the torque.
Here is an image to clarify:
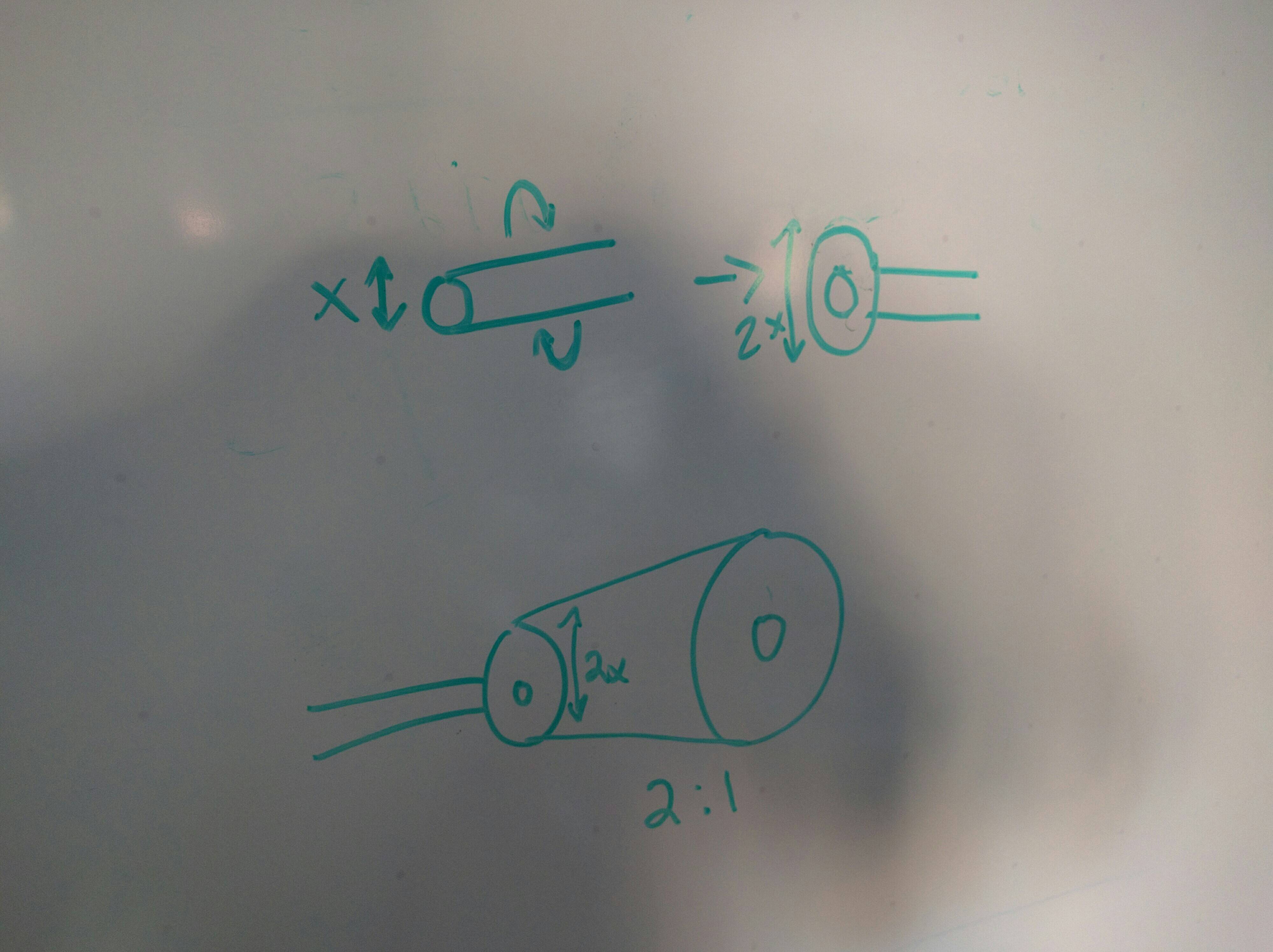
This bugs me because it means the larger a pulley I can fit on a shaft the more torque I get. What am I missing? Are the RPMs reduced?
No. Your basic problem is that "torque is proportional to radius" is wrong. Torque is the expression of normalized rotational force independent of radius.
For example if a motor can deliver 10 Nm torque, then a pulley with 1 m radius can produce 10 N of force, at 500 mm radius 20 N, at 2 m radius 5 N, etc.
It is more useful to say that torque is proportional to the ratio of the radii of a pair of pulleys or gears. Also RPM is reduced or increased in the same ratio so a small diameter gear driving a larger one increases torque but reduces RPM at the output shaft.
E.g., if you had a motor producing 100 Nm of torque at 100 RPM connected to a pulley of diameter 100 mm to an output shaft via a pulley with diameter 200 mm, the torque on the output shaft would be 200 Nm but the RPM would be 50 RPM.
To put it another way, if you ignore friction, gearing systems allow you to have more torque and less RPM or less torque and more RPM but you can't gain any power (in fact you always lose a bit).
