I see some topics in your question that deserve comments, before answering your question, at the end, when I provided a classic reference.
First, head loss coefficient for a valve with respect to the open position is generally provided as a table. e.g. head loss coefficient for a gate valve (link 1)
Topic #1 - Valve selection, valve operation and flow throttling.
I checked the “minor” head loss for accessories listing the Gate valve (I presume a wedge gate one) operated at intermediate position. To confirm, I downloaded the full article (@researchgate) where it appears as Table 7, but it is not indicated which reference it is based on. An issue in that Table is to list data for a wedge gate (I assumed as wedge type, a common type used in industrial piping and pipelines - like API600 std) hinting to operate at 25%, 50% or 75% positions. Something that was not said in the article, but I understood you might have extracted data to make your table, was because it presented head loss references for some intermediate position of a “gate valve”.
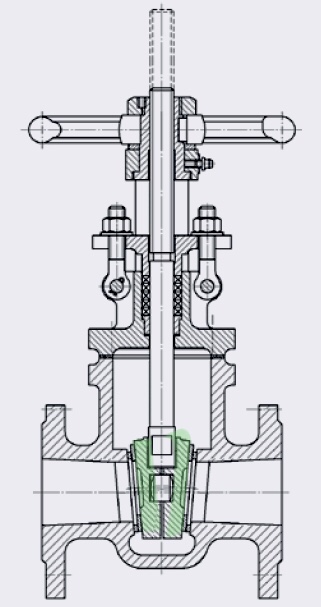
However, when operated in intermediate positions, the gate part (above picture in green) will oscillate, dangling loosely-guided inside the valve body. It would aggravate or not, depending of the flow rate and also of valve orientation (e.g. horizontal or vertical up/down flow); because of that, the actual head loss coefficient would be uncertain on a larger margin. So valve wear will happen and result in early failure.
Side note: I found a short 4 min animation of a wedge gate valve and another longer (40 min) from L&T valves showing most constructive and design features and points of attention.
Getting back on track: it is simply not-recommended to modulate a flow using a gate valve. Restricting a flow with a special slab-gate valve is is already a bad choice and using a wedge-gate valve is just really sad & worse.
Reading the referred article, we see the emphasis is on the thermal analysis of heat-exchangers and the author selected a ball valve for its lower localized head loss - a good choice for that, but that’s it.
Don’t take that data too seriously, as the article simply dumps the head loss data there, no clear units were provided, and readers (as us here) can only make conjectures where it came from.
I prefer to use data from better known tables as here or here, both based on the equivalent length method to estimate the head-loss of fittings and valves; see below the reference to Crane’s TP-410 too.
General method to consider both the Darcy friction factor and valve head loss coeffient for a pipe flow head loss is plain addition: each head loss is considered as a major or minor loss term.
Topic #2 - “Minor” and “Localized” designations of head losses.
Although it is common to see the “Major” and “Minor” wording to head losses in English, the minor head losses are more likely in long pipelines, but this designation can be misleading for piping systems because these localized head losses (as in fittings and valves) can be bigger than the major ones (attributed to piping frictions), as others highlight it too, as in here (Introduction, parag.#4) and here (see misnomer).
That is why I prefer to use terms as localized and distributed head losses. The localized term is for fittings and valves, while the distributed term goes for piping.
Depending of the comparative “equivalent length” of fittings&valves versus the actual piping length, head loss can be major and minor respectively, especially if a valve is throttling or modulating the flow - as globe, needle and choke valves do - or if an orifice plate is used.
Topic #3 - about head loss in valves.
General method to consider both the Darcy friction factor and valve head loss coeffient for a pipe flow head loss is plain addition: each head loss is considered as a major or minor loss term.
Yes, that is correct, discounting my rephrasing preference of minor to localized and major to distributed.
However, because the flow that passes through the valve is also a pipe flow, the head loss coefficient for a valve would be also affected by the flow rate (flow speed).
This comes from the way head-losses are modeled to be computed when using “Darcy-Weisbach equation for a pipe flow”, as you said in the beginning.
Note that most valves even fully open (with rare exceptions) perturb the flow much more than the length in the piping would do. The exceptions are mostly through-conduit valves, where the slab-gate valve is a common example and a full-bore ball valve is another.
Because of that, you simply count the overall length of the piping line (= pipe with fittings and valves) to calculate the distributed losses. Using the equivalent length method, then you add each localized loss for each fitting/valve’s equivalent length - or use the K values (your decision).
Remember that when we select the value of “K” or “L.equivalent” for a given valve, this is just a modeled approximation from actual experimental data; observing numbers more closely (i.e. higher number of significant digits/figures), it also varies from manufacturer A to B.
Another source of (small) inaccuracy comes from the use of “Table of Colebrook equation approximations”, with equations that are calculated faster computationally (being non-iterative), they show deviations of variable degree when compared with the results obtained for Colebrook’s coefficient iterative calculations.
So, there are several contributors to the overall uncertainty of the computer-based modeled solution. Similarly adequate methods give results that would numerically vary, obviously, but to a smaller degree that is within the uncertainty (or inaccuracies) of the models and approximations used, if their boundaries and limitations (as Reynolds, fluid properties, heat transfer, piping geometry, fluid state, etc) are respected.
This effect with respect to the flowrate may be very small compared to the effect of the valve open position…
Not exactly - see “especially if” highlighted considerations, in Topic #2, above.
Your Question: I want to know if there is any table, chart, or experimental result that presents this change in valve head loss coefficient.
ANSWER: Yes, there are practical good ones, probably less in Fluid-mechanics textbooks than in Piping & Hydraulic System Manuals and Technical Papers. Same for articles from internet.
There is a Technical Paper # 410 from Crane that is a classic repository of knowledge and even considers several similar methods to calculate head-loss of fluids in general (known as “Flow of Fluids Through Valves, Fittings, and Pipe TP-410”).
Searching for good books in Piping and Hydraulic Systems, I discovered that for me it was not so easy to recommend good books in English. I’m a Mechanical Engineer, but I live in Brazil, so many practical references as Azevedo Netto’s Manual and Silva Telles’ Book and Charts&Tables are in Portuguese - sorry, I didn’t find them in English - as a picture is worth 1000 words, maybe Charts&Tables continues to be useful regardless of text language (google a sample of it).
In English I could find one textbook that I liked the index (see chapter 11): “Piping Systems Manual - by Brian Silowash”. Other internet-based references, as I linked above, deliver rich data for practical use.
I invite other Engineering fellows here to share their good, practical and reliable piping & hydraulic system titles (sites, textbooks, etc).
Adding them to the comment section would be greatly appreciated.
I believe I have addressed all topics and question you asked. If not so, let me know.
