Flow through Pipe annulus with pressure controlled opening

Question

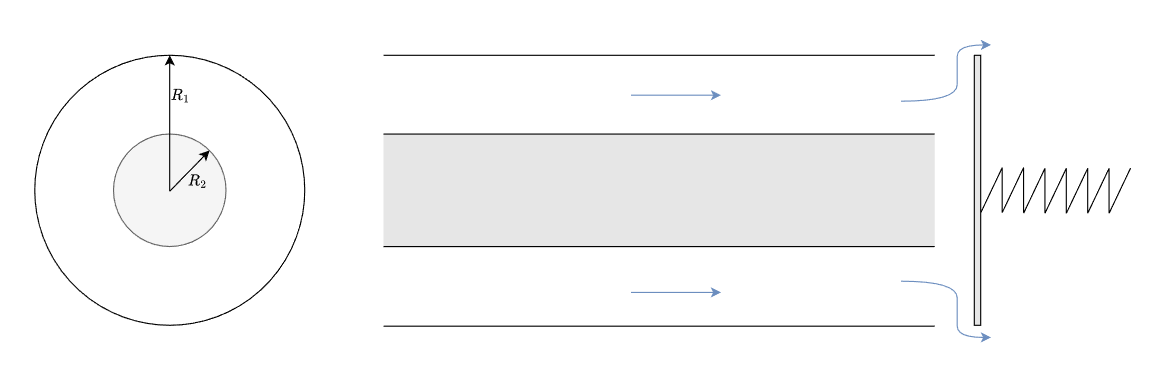
I have a pipe with radius $R_1$ with a smaller pipe within (radius $R_2$). I want to find an expression for the flow through the pipe which flows in the annulus area. However, the known values are the dimensions of the pipe, including the $R_1$ and $R_2$, the length of the pipe (L), and the pressure at each side of the pipe ($p_1$ and $p_2$).

The fluid is fresh water at 10 degrees.

I tried to use Poisseulles law under the assumption of laminar flow: $Q = \frac{(p_1-p_2) \cdot \pi \cdot (R_1^4-R_2^4)}{8 \cdot \mu \cdot L}$

However, this yields an unrealistically high velocity, and flow rate, which I guess means that it is not laminar flow.

So to Calculate the flow in turbulent, I use Darcy-Weisbach : $Q = \Delta p = f \cdot \frac{L}{D} \cdot\frac{\rho}{2} \cdot v_{avg}^2$

Where I find the friction factor in a moody diagram from a Reynolds number where the velocity is neglected.

$Re = \frac{\rho \cdot v_{avg} \cdot D_h}{\mu} = \frac{\rho \cdot D_h}{\mu} \approx 18000 \rightarrow f \approx 0.03 $

I am not sure this is a good way to estimate the Reynolds number. Does anyone have a better way?

Furthermore, the opening at the end of the pipe is controlled by the pressure how can I include this?

Answer 1

For the pressure drop in pipe with annular section, you need to consider hydraulic diameter $D_h$, which is 4 times the ratio of section area to wetted perimeter:

$$D_h = 4\cdot \frac{\pi\cdot \left(R_1^2-R_2^2\right)}{2\cdot \pi\cdot \left(R_1+R_2\right)} = 2\cdot \left(R_1-R_2\right)$$

If $p_2$ is the pressure behind the spring valve, you need to define the pressure just before it $p_{valve}$. When all the pressures are equal, the valve is probably closed with force $F_{closed}$. Now in order to achieve some flow, you need to open the valve, so $p_{valve}-p_2$ has to generate a force $F_p$ higher than $F_{closed}$. For this threshold, you can use static pressure analysis, i.e. $p_1 = p_{valve}$:

$$F_p = \pi\cdot R_1^2\cdot \left(p_{valve}-p_2\right) > F_{closed}$$

Pressure drop at the valve will surely depend on how much the valve is opened (distance $d$), which can be controlled by linear spring with stiffness $k$:

$$d = \frac{F}{k} = \frac{F_p-F_{closed}}{k} = \frac{\pi\cdot R_1^2\cdot \left(p_{valve}-p_2\right) - F_{closed}}{k} = \frac{\pi\cdot R_1^2\cdot \Delta p_{valve} - F_{closed}}{k}$$

The local pressure drop at the valve can be expressed using minor losses formula and it depends on fluid density $\rho$, fluid velocity $v_{valve}$ and a minor loss coefficient $\zeta$:

$$\Delta p_{valve} = \zeta\cdot \frac{\rho}{2}\cdot v_{valve}^2$$

If you express fluid velocity at the valve using volumetric flow $v_{valve} = \frac{Q}{d\cdot 2\cdot \pi\cdot R_1}$ and combine the 2 equations above, you can derive relationship between volumetric flow and opening distance:

$$Q = \sqrt{\frac{8\pi}{\rho\cdot \zeta}\cdot \left(k\cdot d^3+F_{closed}\cdot d^2\right)}$$

As you can see, there is no easy direct solution for this problem, so you will have to use some iterative method. I would recommend using bisection method, where the volumetric flow has to be between zero and $Q_{no\;valve}$, a value you would obtain if there was no valve at all from the pressure difference $p_2-p_1$.

The biggest unknown in this is the minor loss coefficient $\zeta$. Also, be aware about the fact that dynamic pressure of the flow can also contribute to the opening of the valve.

Answer 2

I believe this may be solved by using Bernoulli's Equation Simply expressed Q1=Q2 Inflow must equal outflow regardless of size and shape of the pipe.

The annulus sectional area is all that matters. $$\frac{{^𝑃}{1}} {\rho g}+\frac{{^{V^2}}{1}}{2g}=\frac{{^p}{2}} {\rho g}+\frac{(\frac{{^A} {1}}{{^A} {2}}×{^V} {1})^2} {2g}$$ I hope this this will help answer your question.

Ref:Douglas, J. F., et al. (2005). Fluid Mechanics, Pearson Education Limited.