Is the drag coefficient/velocity of of a vehicle based on frontal area?

Question

Does the frontal area of a vehicle determine the velocity/drag coefficient of a car? A Ferrari 458 has a Cd of 0.33 while a Corvette C6 has a Cd of 0.29. Does this mean the Corvette will have less frontal area and more speed while the Ferrari should make more downforce?

Answer 1

The drag coefficient $C_d$ is defined as:

$C_d = \dfrac{2F_d}{\rho u^2A}$

where:

• $F_d$ is the drag force, which is by definition the force component in the direction of the flow velocity;

• $\rho$ is the mass density of the fluid;

• $u$ is the flow speed of the object relative to the fluid;

• $A$ is the reference area.

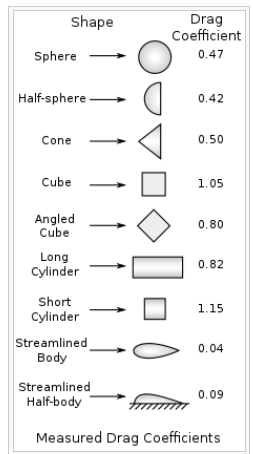
The answer to your first question is "Yes, the reference area for automobiles and many other objects is the projected frontal area of the vehicle". However, the drag coefficient is usually determined experimentally, and the known experimental results indicate that it also depends on the objects' shape as shown below, assuming the reference area is constant for all cases:

It is to be noted that the "shape" also influences the "drag force", in turn, the "down thrust", as $F_d = f(C_d, \rho, u, A)$, and $u = f(C_d, \rho, A, F_d)$. So, owing to the complex inter-dependencies, there is no simple answer to your latter questions.

Answer 2

No Cd is not directly related to the frontal area, it has to do with how much that frontal area moving against the stream of air has to fight drag.

For example, consider the old semi trucks' trailers. Because they were boxy they had a large Cd and needed a lot of fuel for even a smalled frontal area.

In modern trailers, they have round corners and adjustable trailer skirts at the end to make them more streamlined.

So a modern trailer with a larger frontal area has a smaller Cd and produces less drag and is much more fuel-efficient.

If we pick two identical cubes and compare them they have identical Cd. Now if you add a half sphere to the front and end of one of the cubes, Its Cd drop to almost half of the other cube.

Answer 3

The $C_D$ is a convenience factor used by engineers to simplify fluid mechanics for cars and aerodynamics.

A simplified explanation is that the $C_D$ normalises the aerodynamic drag to the frontal area, so that is easier to compare different vehicles.

The statement

"Does the frontal area of a vehicle determine the velocity/drag coefficient of a car?"

to me implies that the size of the frontal area affects the $C_D$ (it implies that the larger the area the greater the $C_D$ or vice versa. However, the $C_D$ it largely independent of the frontal area, although it is calculated from it.

$$C_D = \frac{2\cdot Drag}{\rho u^2 A}$$

where:

• $Drag$ aerodynamic force
• $\rho$ air density
• $u$ is velocity
• $A$ is frontal area.

So for example, changing the angles on a rear spoiler, has zero to little effect on the frontal area, but it can change significantly the $C_D$. In that respect those two are independent.

The one case I can think of (there are probably others) that $C_D$ depends on the frontal area, is when the frontal area becomes too small, and therefore the Reynolds number is significantly affected. However, this is probably not too relevant to cars.

---

So regarding the statement:

Does this mean the Corvette will have less frontal area and more speed while the Ferrari should make more downforce?

Speed is depended on a number of things. I.e. the engine horsepower is important, the gear shift ratio, wheels etc.

Therefore:

• you can't make an assessment of the frontal area based on the $C_D$
• $C_D$ is responsible for the drag while $C_L$ (coeffient of lift) is responsible for the downforce (Although there is a correlation between $C_D$ and $C_L$).
• you can't make an assessment for speed. See below. Which of the two would you think has the highest speed.

	Image	$C_D$
Aptera -2		0.15
Buggati chiron		0.38