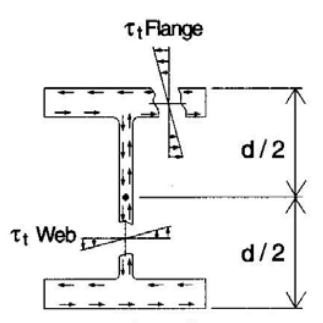
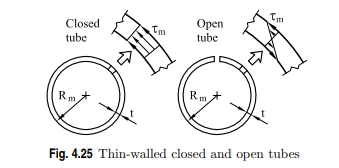
It is already known that the I-beam is very bending resistant (causes less deflection for the same transverse load and also results in lesser stresses relatively). But for an eccentric transverse load (i.e. the line of action of load does not pass through the axis of the beam), the I-beam can also be subjected to torsion. And it is believed that I-beam are not very strong in torsion.
Can anyone point out the reason that why I-beams are not very resistant and strong in torsion, but when subjected to a transverse load, they can resist the transverse shear stress? What is the difference between the shear stresses coming from torsion and coming from tranverse loading?