0
$\begingroup$

I was studying about shear stresses in beams, where one of the ways the textbook tried explaining intuitively the presence of shear stresses in beams was:

enter image description here Source : Mechanics of Materials, R.C. Hibbeler

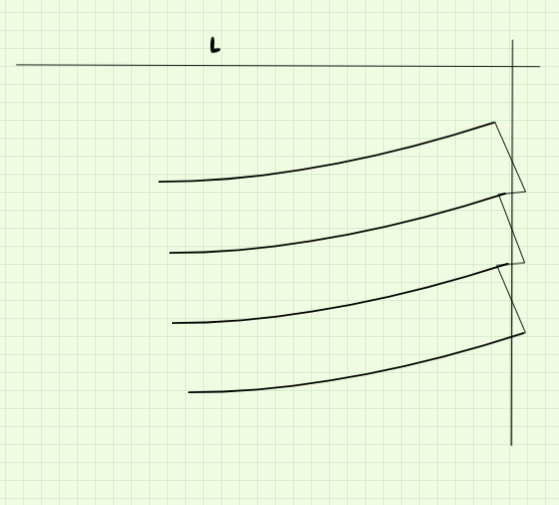
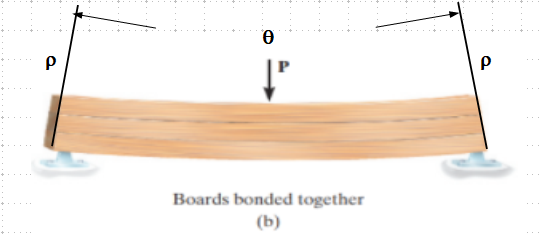
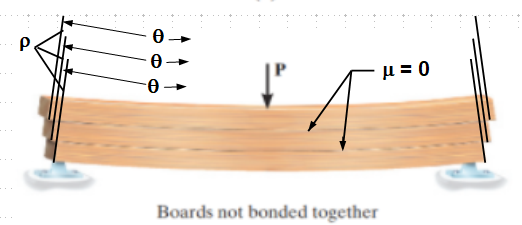
It stated that if the three beams stacked on the top of the other are not bonded then after the application of load they will look like as shown in (a). (Assume the surfaces on the beams are frictionless). In the bonded system, the beams will look like as shown in (b).

Even though I can feel intuitively, in case (a) the beams will be as shown, but I don't get

What is causing them to elongate? Why the topmost beam elongates more than middle one?

Improve this question
$\endgroup$
0

4 Answers 4

Reset to default
2
$\begingroup$

The truth is that the elongation is less when the beams are not bonded. What is causing the contraction and extension is the compatibility constraints which are applied due to the bonding.

HAve a look at this question also which deals with this problem.

Improve this answer
$\endgroup$
1
$\begingroup$

We cannot model each and every physically occurring phenomena in structures using analytic mathematical models, since this would mean we have develop infinite number of analytic mathematical models to represent each and every combination of structures and loads, which is practically impossible. The only thing you can do here is to use your natural instincts in predicting why and how.

You can do an experiment at home with 3 cards or wooden planks, and check it for yourself. You will find out what is said in the book is correct, (without referring to any mathematical model). You keep this phenomena in mind, and use it somewhere else in the future.

The 3 planks in figure 1 are not elongating, btw. They are just turning to obtain a curve about a certain origin. This center of curvature will be different for each plank in the figure 1, but the same in figure 2.

Improve this answer
$\endgroup$
1
  • $\begingroup$ So is it that each plank below the load is deflecting by different amounts, with the topmost plank deflecting the least and bottomost plank deflecting the most? Because then it makes sense why the shortest distance between the ends of the top plank is the largest as compared to the shortest distance between the ends of other two planks. For eg. if I take three wires of same length, and curve them by different amounts, the one with lowest curvature will appear "longest" (I mean if I join the ends after curving, that length will be longest for the one with least curvature) $\endgroup$
    – Harshit Rajput
    Commented Feb 20, 2022 at 17:33
1
$\begingroup$

The three planks do not elongate they deflect more and because their ends stay perpendicular to their deformed centroid they look like that.

The beams deflection in this case is

$$\delta= \frac{PL^3}{48EI} \quad and, \ I=\frac{bh^3}{12}$$

Meaning the deflection of the 3 stacked beams will be proportional to

$I_{stacked-beam }\propto3h(1/3)^3=3h/27=h/9$

$$I_{stacked-beam}=1/9*I_{original}$$

So the planked version deflects 9 times and the rotation of the end causes the illusion that they have elongated. Check the drawing.

'

planked beam

.

Improve this answer
$\endgroup$
2
  • $\begingroup$ The deflection under the load is the largest for bottommost beam and smallest for topmost? in the unbonded case? $\endgroup$
    – Harshit Rajput
    Commented Feb 20, 2022 at 17:43
  • $\begingroup$ @HarshitRajput No, The deflection is the same for all boards. You could imagine them as 3 identical springs in a series, they all expand equally. $\endgroup$
    – kamran
    Commented Feb 20, 2022 at 18:13
1
$\begingroup$

The elongation is due to the deflection

This is the phenomenon I wanted to address in your question over the composite beam - composite beam requires adequate bond strength in between layers to make it as a monolith, otherwise, it is a stacked beam, with each layer working as an independent beam.

Simply to say, while the deflection of the layers (of different materials or shapes) in a composite beam conforms to a single curvature everywhere along the beam; in the stacked beam, each of the layers will deflect the same amount everywhere along the beam, if no friction at the interface, otherwise, the length of each layer will be influenced by the interface friction of the materials, as shown in the original case (a) and/or the last figure below.

enter image description here.

enter image description here

enter image description here

Note, there are many factors affecting the behavior of the stacked beam. For example, a short beam lays on top of a longer beam below, depending on the loading and stiffness of each beam layer, the sketch below could result:

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ r13, I need to understand this first, the deflection under the load in unbonded case, is same for each plank or different? $\endgroup$
    – Harshit Rajput
    Commented Feb 20, 2022 at 17:41
  • $\begingroup$ Yes, for layers with identical material (E) and geometry properties (b, t), and without shear friction at their interface, the layers will deflect/stretch to the same amount. However, it is not the reality, but by theorey. $\endgroup$
    – r13
    Commented Feb 20, 2022 at 18:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.