Why does concentrated shear force on a beam influence the whole beam?

Question

This might be a silly question but can someone explain how this shear force on the right side of the beam causes Qy throught the whole beam?

I understand it mathematically but not conceptually so I would be grateful is someone could help me understand this via an analogy or something, because when I solve mechanics I am often stuck on such simple problems because intuitively it feels incorrect to me.

I'm aware there is also the M force on the beam but I left it out of the picture because that one I can visualize and I understand it.

In other words, why does the whole beam "feel" this force (if we ignore bending for a moment)? if you press down on my hand I don't feel that pressure anywhere else on my arm.

Answer 1

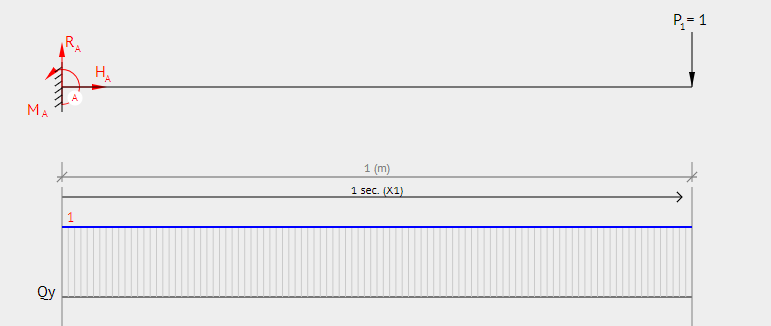
So, we apply a point load to the end of the cantilever beam and can see that for external equilibrium, there must be shear reaction at the support. (Leaving aside for now the moment reaction that also exists.) We have an intuitive understanding of this Newtonian action-reaction from our daily lives.

So, the next question is, how is the load 'getting' from the point of application to the support? We can assess that by looking at internal equilibrium. If we start from the right end of the beam (where the load is applied) and cut the beam at any section, there must be a shear reaction for the beam segment to be in equilibrium. We can see this using the same logic as for the external equilibrium.

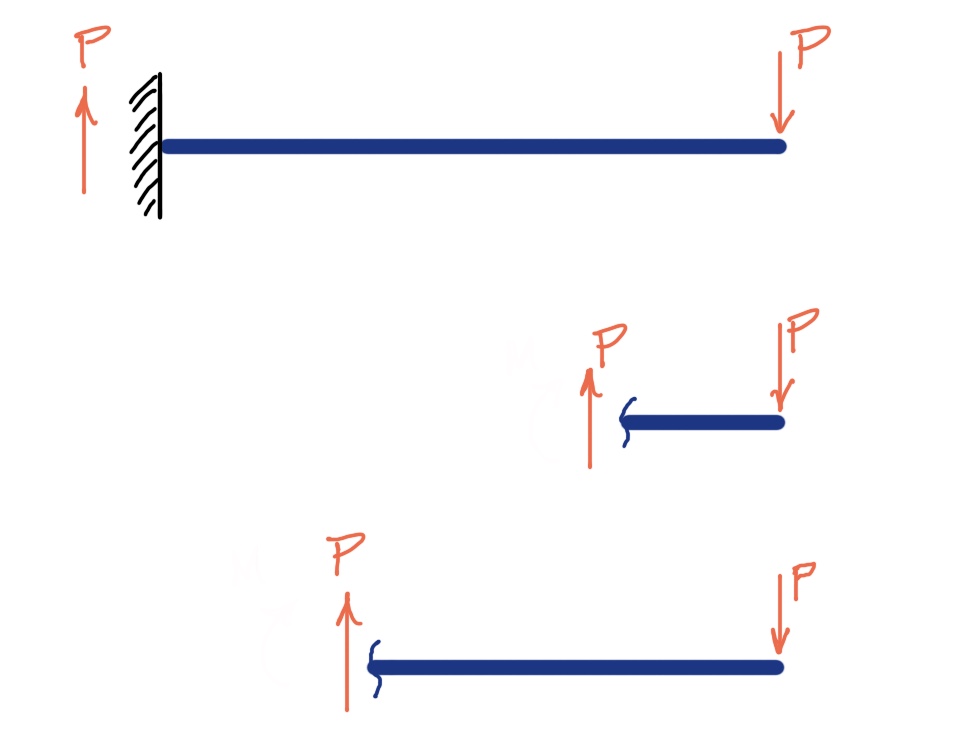
This illustrates that the applied load creates a shear force at every point along the beam.

The example you gave from the human body is perhaps a difficult one for developing this intuition, given the complexity of muscle, tendon, and bone. However, you mention that if you you press down on your hand you don't feel the "pressure" anywhere else, so this may be about distinguishing between internal and external equilibrium. Pressure is external. The beam (or your hand) only feels that external pressure at the point of load application and at the support. However, if you hold out your forearm and hand rigidly and push down on the tips of your fingers, you will feel muscles and tendons reacting to the load to maintain equilibrium.

Answer 2

If we cut a section at any point of the beam, an equal and opposite force will be there (shear reaction) to maintain the equilibrium. So if we think it as a differential section, it will be easy to understand.

Now imagine this differential section extended to length 'l', each fiber of the beam will have a shear resistance until it becomes equal to the force magnitude.

An understandable analogy (probable explanation) to this would be:

Imagine this cantilever beam is cut at any point away from the end points, the section not connected to rigid support will end up in a circular motion along the trajectory of the tangent. This is because the force applied and shear resistance at the cut end will form a couple which will make the beam rotate. Hence this proves that if we apply force at one end of the cantilever beam, it's resisting force is felt at every point of the beam. Hope this clears you confusion.

Answer 3

The shear force has to continue along the beam until a point where it meets a force of equal magnitude and opposite direction to set it off, which in you case is the left hand support reaction.

Intuitively if you cut the beam at 1 cm from the load, at the left you have P1 force which has not been countered by any reaction yet, pushing this section down and you need to apply a balancing vertical force of P1 directed up, to keep it in equilibrium, otherwise it will fall down. Same thing if you cut the beam at 2,3,.. cm. So throughout the beam the shear continues until it has encountered the opposing force at the left support.