Does hitting an obstacle hard enough to break it actually reduce self harm?

Question

Lets say that Car-A hits barrier that is fixed to immovable posts on either side of the road. The car's momentum is such that the barrier absorbs the impact and the car comes to a near instantaneous halt and incurs some degree of smash damage.

An identical Car-B hits an identical barrier, but its momentum is such that the barrier snaps and hinges open like a gate, allowing the car to safely roll to a stop. Obviously, Car-B also incurs some degree of smash damage.

Assuming the difference in speed was about 20%, which car would you expect to incur more smash damage?

Also, given that different materials fail in different ways (snapping, shattering, warping, etc.) Does the nature of the barrier matter? Does the nature of the vehicle matter?

Can you reduce the damage to your car by pushing the accelerator instead of the brakes?

Can Superman cause MORE harm to his nemesis by throwing him more gently into a wall?

Can a stunt actor get an unexpected injury because he didn't collide with the window hard enough to punch through?

Answer 1

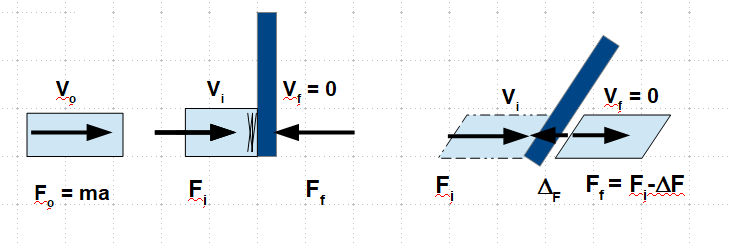
These scenarios are too simplified to be able to form an opinion.

But generally "unyielding barrier" will cause more damage.

Let's say the yielding barrier never breaks, only bends and moves one meter.

assume these factors just for the sake of a perspective.

• car mass M kg

• Car speed at crash V

• d, distance car moves to a full stop 2 meters for 1st case(total pancaked front), 3 meters for 2nd.

$$F_{average}d= -\frac{1}{2}MV^2, \quad F_{average}=\frac{-\frac{1}{2}MV^2}{d}$$

Just by comparing

$V2/V1=1.2^2=1.44\ with\ d2/d1=3/2=1.5,$ we see any deflection larger than one meter of the yielding barrier will be less force to the car.

This is not even considering the deceleration which is greater in the first case.

• As to the part of the question hitting the subject a bit harder:

That is the game plan in martial arts or karate maneuver of breaking planks or concrete blocks safely by hitting fast. Obviously, they have practiced and learned from their master what to hit and what not to.

I would not try it on a car, even though I myself in sudden emerging black ice road conditions get very close to the car in front of me to minimize the potential impact.

Edit

I thought a short review of "hitting a bit harder to break an object could inflict less self-harm!"

Questin of impulse and hitting an object with the aim of destruction has been for centuries the subject of weaponry and armory (Andulosian swords) and using penetrating shells and resisting shields for eons. The War industry does a lot of experiments and research on alloys and speeds of shells but they keep their data well secret.

But theoretically at least in martial art, if one has a trained hand with calluses, with a strengthened skeletal-muscle system and they have the right material which will eventually break as opposed to deform. It is plausible to think if you just hit the target a bit harder to break it your hand will go through with carrying on the motion after a glance of shock and won't have to eat back all its impulse and break your bones.

Basically hitting hard and collision in the real world is a messy physics, half plastic, some elastic, a lot of heat, and in intense collisions cratering and a cascade of molten metal radiating out.

Answer 2

For simplicity, let's hold the speed constant, the rigidity of the walls variable. The sketch below conceptually depicts two scenarios - the car runs into a non-yielding (rigid) wall and then runs into a defective yielding wall.

Car A suffers damage due to the full impact force. The impact of car B will be absorbed partially by the crushed wall, which forces car B to decelerate, so the damage will be less severe if all other things being equal.

Approximate Comparison of Deformations:

Let $\Delta x$ be the deformation of the car at the collision. For case 1:

$ma\Delta x_1 = m\dfrac{V_f^2 - V_i^2}{2}$, $V_f = 0$

$\Delta x_1 = -\dfrac{V_i^2}{2a}$

For case 2: Assume $V_f = 0.5V_i$

$ma\Delta x_2 = m\dfrac{0.25V_i^2 - V_i^2}{2} = -0.75m\dfrac{V_i^2}{2}$

$\Delta x_2 = -0.75\dfrac{V_i^2}{2a} < \Delta x_1$

Answer 3

The energy required to break an object will be independent of the speed of the thing hitting it. This is known as the toughness of a material. That energy is what the striking object will need to impart and this will have the same impact energy imparted to itself. The way this is used in practice on the roadways is by having wooden posts at the end of railings where a vehicle might hit it lengthwise. (Note that some of these designs have been found to be quite faulty as the metal rail itself can become a jousting pole into the car.) Each wooden pole is relatively easy to break, giving up its energy in smaller doses, versus one big I-beam that would decelerate a vehicle suddenly. Water-filled barrels are used to the same effect.

[Edit:] So, hitting an object if the object doesn't break will dump all kinetic energy into both the object and whatever was moving. If you hit the object hard enough to break it, the kinetic energy dump that broke the object will be experienced by the moving object. A faster hit than that will just cause the moving object to continue to travel past the broken thing until it hits something else. If you hit harder you are guaranteeing that maximum kinetic energy dump due to destruction or the struck object will occur.

What the idea of energy needed to break something is that, NO, you can't hit something softer by hitting it harder. And if you strike a barrier and continue through it, expect to hit something on the other side. Dumping energy into your brakes will always be a better idea than accelerating.

I will add that there are cases where breakage is better. These are cases where the object is meant to break. Karate boards come to mind. These are specific types of wood that break when you hit them right. This would not work karate-chopping a piece of dimensional lumber meant for home construction, and certainly not for the objects designed to be hit by cars.