1
$\begingroup$

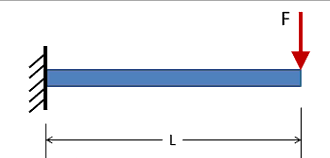
I am not a mech engineer and have been struggling with this for a while, I feel like it is pretty straight forward but I'm missing something.

The problem is a horizontal tube that is supported at one end with the weight on the other end. My specs are length of 3 ft, outer diameter of around 2 inches, and a force of 120 lbs.

A diagram: enter image description here

Basically I want to figure out the optimal outer diameter (2 inches +- .5 inches or so) and minimal wall thickness. (The cheapest tube)

I can get tensile strength from most carbon fiber tube manufacturers.

I believe I want to calculate maximum stress and more specifically sheer stress for a hollow tube. But I haven't been able to find the calculations that are in terms of tensile strength.

Any pointers or references in the right direction would be awesome!

Improve this question
$\endgroup$
3
  • $\begingroup$ The short answer is you can't. The mfg. might have the info. It depends on the very fine details of the fiber orientation within the tube. Tiny variations in manufacturing can have a huge impact. Carbon laminates are horribly non-isotropic. The failure mode here will be buckling, with the stress profile varying along the tube. Finding the buckling mode, and point of buckling, for a non-istropic tube in bending and shear, is not for the faint of heart. $\endgroup$
    – Phil Sweet
    Commented Jun 2, 2022 at 9:55
  • $\begingroup$ Unless you can afford exhaustive testing, you'd be better off with a material that is more isotropic, and can be designed with a wall thick enough to simplify the buckling analysis. Aluminum would be my choice. Sans a testing program, aluminum would probably be lighter for most L/D ratios of interest. $\endgroup$
    – Phil Sweet
    Commented Jun 2, 2022 at 9:56
  • $\begingroup$ Why do you not use metric units? $\endgroup$
    – 12431234123412341234123
    Commented Jan 23 at 12:39

1 Answer 1

Reset to default
0
$\begingroup$

if we assume the tube carbon fiber has equal tensile and compressive strength and young modulus, which is not necessarily true in all cases. and with a negligible mass.

Then we have $$ M= PL = 120*3*12=4320lbs.inch$$

$$\sigma =M/S \quad S= \text{section mudulus}= 4320/s \quad , \ S=\sigma/4320$$ and for a hollow tube beam

$$S= \frac{\pi(d_2^4-d_1^4)}{32d_2}$$ And having $\sigma$ acceptable stress of your material you can find the thickness $d_2-d_1$

Shear stress is $$V=12*1.5 / A_{beam}= 180lbs/(\pi(r^2_2-r_1^2))$$

These are low stresses and most likely any size commercially available will do the job.

But if your carbon fiber has different E, young modulus for tension and compression and different strength then you have to find the neutral axis which will shift up or down from the geometric CG by the ratio E1/E2, E= young modulus, and find the S and take it from there.

Improve this answer
$\endgroup$
6
  • $\begingroup$ Thank you! This was a lightbulb in my head. I did some research and compression is slightly weaker than tensile for standard modulus CF, but not by much. By different E, do you mean modulus/tensile/compressive strength at 0 deg. versus 90? $\endgroup$
    – Joshua von Damm
    Commented Sep 5, 2021 at 4:43
  • $\begingroup$ No E, the Young modulus is defined as E= sigma/proportional deformation. simply said if you have a bar of an area of A and length of L then apply it to a tension T and it elongates 0.01*L its E = (T/A )/ 0.01= T/A*100. E1andE2 are tensile and compresive Es.if you material Es are close just assume normal S and apply the standard formula. $\endgroup$
    – kamran
    Commented Sep 5, 2021 at 5:13
  • $\begingroup$ Alright I see, thank you. And if I could trouble you with another question, what would the σ acceptable stress be? With my current specs and a 2 inch diameter with a .05 inch wall, I get a sigma of about 57 ksi. Typical CF has a modulus of 33 MSI and tensile/compressive of 500 ksi, and I'm not quite sure how to compare the them to the result from the equation. $\endgroup$
    – Joshua von Damm
    Commented Sep 5, 2021 at 5:23
  • $\begingroup$ it means your beam has a factor of safety of about 9. means it is over kill. check the shear too. $\endgroup$
    – kamran
    Commented Sep 5, 2021 at 5:27
  • $\begingroup$ The strength can very by a factor of 50 depending on fiber orientation. The tube will fail in buckling near the wall. The buckling mode can be very hard to predict. If you make it thick enough to not buckle, I suspect aluminum would be lighter and a lot cheaper. $\endgroup$
    – Phil Sweet
    Commented Jun 2, 2022 at 10:02

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.