the question is clear and straight forward but when i get the velocity it should be ±√2
what i don't understand is the guide answer refusing the -ve value because acc is always +ve
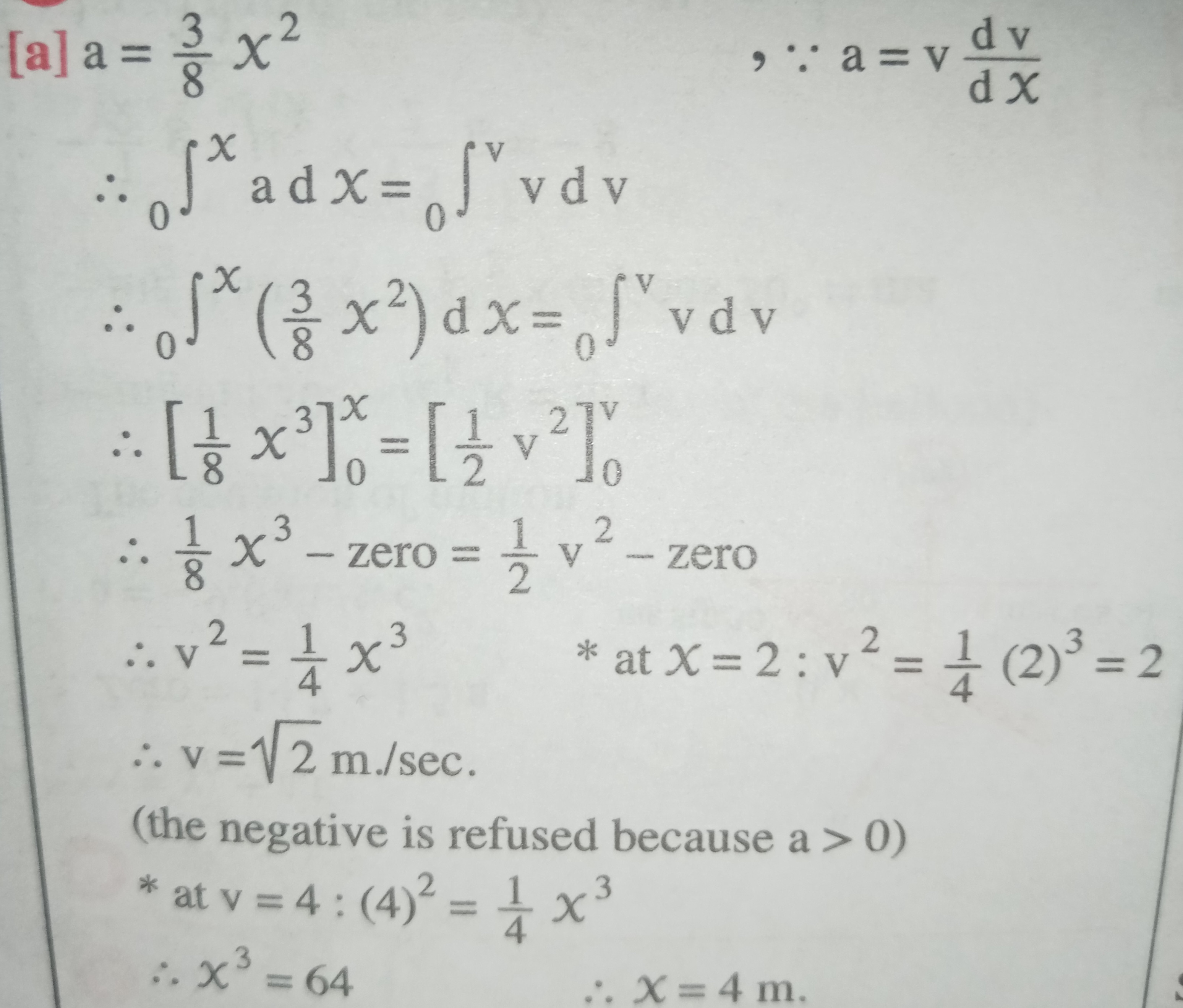
thank you in advance
the question is clear and straight forward but when i get the velocity it should be ±√2
what i don't understand is the guide answer refusing the -ve value because acc is always +ve
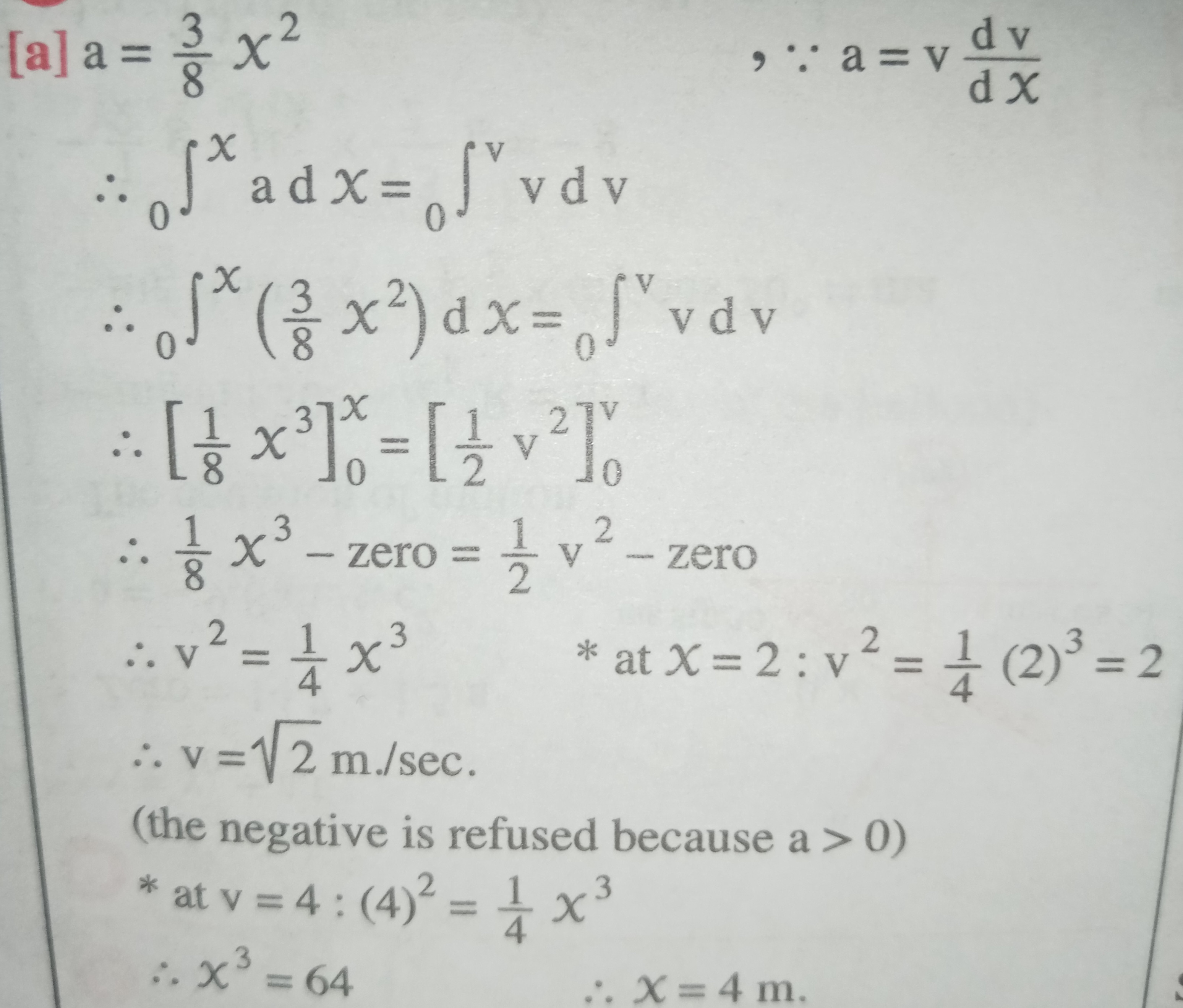
thank you in advance
No, positive acceleration, alone, does not need to imply positive velocity. Another term for "acceleration in the opposite direction of the velocity" is just "deceleration".
The negative value is being rejected based on physical context. That is, it is being rejected based on the fact you are starting from rest along with the fact that acceleration is always positive.
I will rephrase DKNGuyen's answer (which is correct - I upvoted ).
I assume that the positive (+) sign is for position, velocities and acceleration is to the right.
The object is starting from rest. Therefore its velocity is zero. We don't explicitly know the initial position, but we can assume that its a very small value different that zero (any value however small would do and whichever direction $\pm$).
The acceleration is given by:
$$a(x)= \frac{3}{8} x^2 >0 $$
Therefore, because a is greater that zero, the object will accelerate towards the right. There is no position where acceleration can become negative (and therefore at any given time the acceleration can be positive or zero), therefore, the equation:
$$V = V_0 + a\cdot t$$
means that the velocity is always greater than zero (since the initial velocity is $V_0$) in the parameters of this problem.
The other solution (-ve) the post mentions is reserved for the case when:
$$a = \color{red}{\mathbf{-}}\frac 3 8 x^2$$