7
$\begingroup$

enter image description here

These are suction-mounting hooks. I want to visualize and understand how do they stick on walls.

After these hooks stick on wall. Like we compressed them. How? Since gases are compressible up to a good finite limit. What makes it difficult for them to come off if I pull them. It should be easily able come off also. Online , it says that the air gets enclosed inside . But I am not getting exactly what it means by that. How does that air enclosed creates a way in which it becomes difficult for us to pull the hook off.

Improve this question
$\endgroup$
2
  • 9
    $\begingroup$ I would call this a "suction cup". I've never seen "airlock hook" or "air lock hook" before. Googling those phrases brings up this very question as a top result. $\endgroup$
    – Harabeck
    Commented Apr 27, 2021 at 16:31
  • $\begingroup$ Changed the title and content to refer to "suction cups." Original post called these "air locks" which are something entirely different. $\endgroup$
    – feetwet
    Commented Apr 28, 2021 at 16:28

2 Answers 2

Reset to default
24
$\begingroup$

TL;DR: Its not the air that's enclosed inside that creates the force, but the lack of air - or more precisely pressure.

First of all some nomenclature (this is for the closed type, there is also the open type):

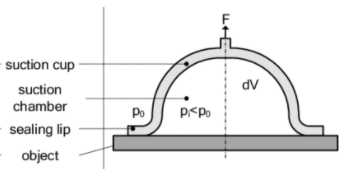
enter image description here

When you press a suction cup on a surface what happens is that the air inside it is pushed out. During the compression phase of the cup the air comes out of the lip to the atmospheric pressure. So if you have an original volume $V_0$ of the suction cup, and then the volume reduces to $V_2 = \frac{V_0}{2}$ (usually there is even less volume), then the mass of the air trapped inside the suction cup is about half the original (keep this in mind for later on).

enter image description here Figure 2: Air exiting . (source: ijiset.com)

The dome is flexible, so while pushing it is easy to change its shape (thus force the the air out). Additionally the shape of the lip (at a tangent with the object makes it easy for the air to be driven out). However, after the external force is removed then because of the material elasticity, the suction cup is trying to return to the original shape.

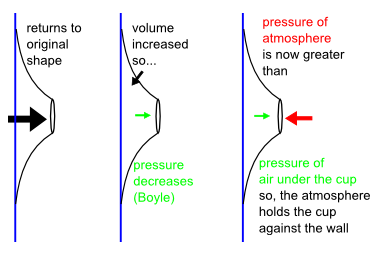
enter image description here

Figure 3: Different stages. (source: Abetterchemtext)

Following on with the example, because the suction cup tries to recover (partially), as the volume of the suction cup tries to increase the air density reduces, and so does the pressure. So what you end up is a low pressure underneath the suction cup. If the suction cup were allowed to recover its original shape (it doesn't) then the pressure in the example would be half the pressure.

enter image description here

Figure 4: Low and high pressure regions. (source: wired)

Then the "suction" force is equal to :

$$F = A\cdot dP $$

where :

  • A is the area of the suction cup (its projection)
  • dP is the difference in pressure ($P_{High}- P_{low}$)

numerical example

Assuming a diameter of 50 mm, and a pressure difference at about half the pressure of the atmospheric pressure, then you can estimate that the pull out force would be approximately:

$$F = \frac{\pi d^2}{4} \Delta P $$ $$F \approx 2 \; [N]$$

Notice that if you double the diameter the force quadruples, so you can get significantly higher forces.

Why some surfaces are better

The reason why smooth non porous surfaces tend to behave better with suction cups is that there is not much leakage (from the outside in).

enter image description here

Air coming in the suction chamber would increase the pressure inside the chamber, and therefore reduce the pressure differential $\Delta P$.

Improve this answer
$\endgroup$
3
$\begingroup$

When you push that onto a flat surface it pushes most of the air out.

The elasticity of the item makes want to flex and come off but the air pressure is pushing it onto the surface.

Improve this answer
$\endgroup$
6
  • $\begingroup$ After you put in wall , it can’t push any air out right since once It is on t wall , it can only push that air which has come inside of it. Then , you talk about air pressure. How does that air pressure push it onto the surface ? Pressure is exerted in all directions. $\endgroup$
    – Rider
    Commented Apr 26, 2021 at 17:24
  • $\begingroup$ So true pressure is exerted in all directions, but how do you think the air pressure is coming through the surface of the material you have just pushed it on? $\endgroup$
    – Solar Mike
    Commented Apr 26, 2021 at 17:35
  • $\begingroup$ The air inside which is trapped , it will try to apply force in direction opposite to wall. Right ? Therefore , loosens up $\endgroup$
    – Rider
    Commented Apr 26, 2021 at 17:41
  • $\begingroup$ That air inside which is trapped is now being forced to expand into a slightly larger volume due to the elasticity of the hook trying to flex away from the surface, so the outside air pressure is greater than the internal. $\endgroup$
    – Solar Mike
    Commented Apr 26, 2021 at 17:46
  • 2
    $\begingroup$ @Rider You're correct, the air inside is applying a pressure on the inside, away from the wall, but the air outside is applying a force on the outside, towards the wall, and that force is much greater. $\endgroup$
    – anaximander
    Commented Apr 27, 2021 at 13:07

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.