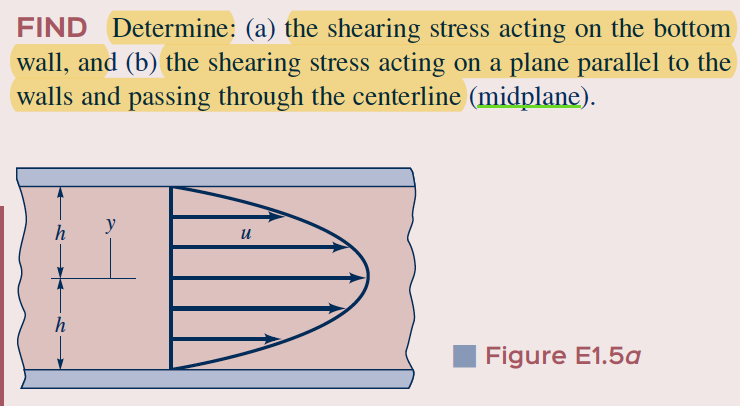
If I understand your question correctly your qualm is about $\tau$ being negative.
I will use the same image as karman because it is a very good one:
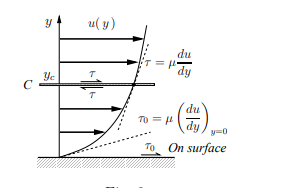
you will notice that shear stress is defined as:
$$\tau = \mu\frac{d u}{dy}$$
Now notice in your image:

that for increasing y, the velocity increases up to the middle of the pipe. (That is because the fluid in the center transfers momentum to the fluid that is closer to the wall, although this is incosequential to your question).
The important thing is the gradient of the velocity $\frac{d u}{dy}$. As you can see for increasing y, from the lower wall up to the middle of the cross-section the gradient is positive (ie. velocity increases).
Right at the middle, the velocity gradient is zero (local extreme/local maximum), therefore the shear stress is zero.
Above the middle of the section, velocity drops, therefore the shear stress is negative.
This is all a mater of convention. If you had y, increasing downwards then the upper half would be positive, and the lower half negative.
UPDATE 1
(The way I perceive it) shear stress is a measure of the forces between adjacent layers of the fluid (in laminar flow specifically). High shear stress, means that between each layers there are high forces and therefore there will be a greater change in velocity. However, as you can see in order to determine the change in velocity you need to consider a direction orthogonal to the velocity (hence the gradient). So, (as in other cases of shear stress definitions), shear stress is positive when:
- for increasing y ($\Uparrow$), the velocity increases($\Uparrow$).
- for decreasing y($\Downarrow$), the velocity decreases($\Downarrow$).
while it is negative when:
- for increasing y($\Uparrow$), the velocity decreases($\Downarrow$).
- for decreasing y($\Downarrow$), the velocity increases($\Uparrow$).
UPDATE 2:
if you notice the graph with the definition of the shear stress, there is a very interesting detail. (see bollow for detail). You can see that $\tau$ is presented as half arrow.

The half arrow (among other things) make sense because $\tau$ is applied on a surface/plane. So the top half arrow is applied on the top of the fluid surface "layer". Also notice that immediately below there is another half arrow pointing the opposite direction. It is useful to note that this $\tau$ is also positive. You might ask: "How can it be?"
The convention is that the sign of the shear stress (Actually all stresses but that;s another matter) is defined by two things:
- the direction of the shear stress.
- the direction of the normal to the surface compared to the positive direction (in that respective axis).
if both directions are positive or negative then the shear stress is positive. If one direction is positive and the other is negative the shear stress is negative. (I tend to think about it as multiplication +1*+1 = 1, (-1)(-1) =1, and (1)(-1) =-1)
So for example: the top half arrow:
- $\tau$ points to the positive x
- the normal of the surface that $\tau$ (the top half) is applied is pointing upwards (the normal to a surface is always points to the side that the stress is applied so top). Upwards is positive y.
So the top half of $\tau$ is pointing to positive x and the direction of the normal to the surface point to positive y. Since both are positive then the $\tau$ is positive
Also for the bottom half $\tau$ arrow:
- $\tau$ points to the negative x
- the normal of the surface (that $\tau$ (the top half) is applied) is pointing downwards. Downwards is negative y.
So the bottom half of $\tau$ is pointing to negative x (-1) and the direction of the normal to the surface point to negative y(-1). Therefore, the bottom half is also positive.
This so far applies for the bottom half of your original post. For the top half, the direction of the shear stresses would be reversed, and as such the sign of the shear stresses with this convention that is solely based on the directions would be negative (try it out for your self).
Following this convention for shear stress, uses the positive direction on the horizontal axis (where velocity is positive).