Air - rectangular membrane system vibration and natural frequency

Question

I'm struggling with the following problem:

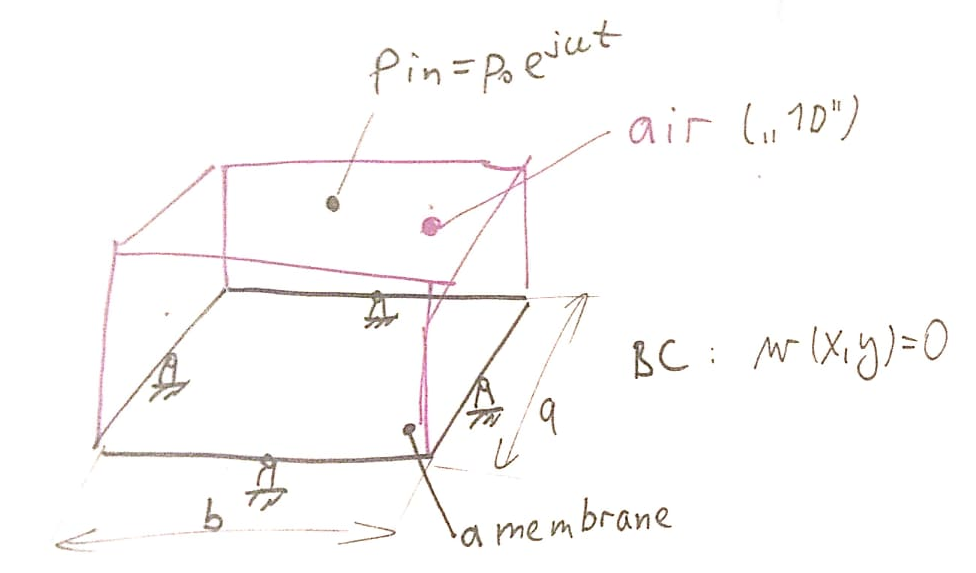
The boundary conditions are $w(x,y) = 0$ around the edges.

I am supposed to find the natural frequencies of the system as a whole, and find the solution for $w(x,y,t)$ based on the inout $p_{in}$. The air can be perceived as a 1-D continuum to simplify the equations.

There is a body of air above the membrane with the pressure at height L above the membrane being defined as $p_{in} = p_0 e^{jwt}$ I know how to solve this problem for the membrane alone with some initial conditions but I'm lost as how to work with this problem as a whole. I don't see how could I use the known equation $\frac{\partial^2 w}{\partial t^2} = \frac{\sigma_1}{\rho}(\frac{\partial^2 w}{\partial x^2} + \frac{\partial^2 w}{\partial y^2})$ to find the solution.

So my guess would be that I have to find the solution for a 2D wave equation with a source, then write a boundary condition equating the pressure in the air at the surface of the membrane to the source in the membrane equation and go from there. Meaning $\frac{\partial^2 w}{\partial t^2} = \frac{\sigma_1}{\rho}(\frac{\partial^2 w}{\partial x^2} + \frac{\partial^2 w}{\partial y^2}) - \frac{p(x,y,t)}{h \rho}$ and $p_{air}(x = L, t) = p(x,y,t)$.

Any help, insight or link to relevant materials will be greatly appreaciated.

Answer 1

I wasn't able to find the analytical solution but I at least tried to make a few steps in the right direction, which was enough for a B from the professor, which likely means it was indeed a step in the correct direction.

The solution of a 2D wave equation with a source is a sum of homogenous + particular solution of the equation. $$ w(x,y,t) = w_h(x,y,t) + w_p(x,y,t)$$ The boundary conditions for the system at the air-membrane junction are $$\dot{\xi}_{air}((L-w(x,y,t),t) = \frac{\partial w(x,y,t)}{\partial t}$$ and $$p(x,y,t) = p_{air}(L-w(x,y,t),t)$$

The obstacles in being able to solve this equation with given boundary conditions are

i) $(L-w(x,y,t))$ is problematic. The approximation of $p_{air}(L-w(x,y,t),t) = p_{air}(L,t)$ is not feasible.

ii) The necessity to find a particular solution of a sourced 2D wave equation satisfying these conditions.

Due to my background in Mechanical engineering (and lack of experience with finding solution of this type of PDEs) I wasn't able to continue any further.

If anyone ever faces a similar problem and manages to make any further progress, please do contact me as I'm interested in what the actual solution looks like.