What is the maximum angle of a shelf angled brace?

Question

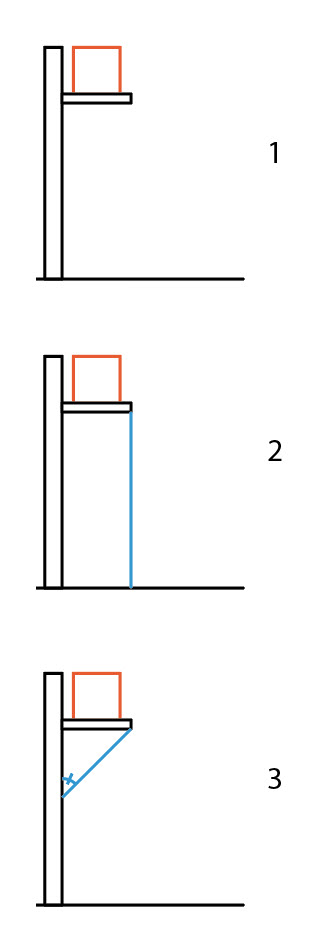
So suppose I want to make a single shelf protruding from a wall and to place a heavy object on it (Figure 1). A shelf like this would fail (assuming no internal mounting that penetrates into the wall).

As such, in an ideal scenario, I would want to place a vertical column/beam directly below the shelf to help support the weight on top (Figure 2).

Yet this isn't always ideal due to other objects below the shelf. So in this case I've seen angle braces being used below the shelf (Figure 3). Now generally, from what I've seen, these angle braces are roughly 45 degrees from vertical. Why is that the common angle?

I haven't been able to find any calculations online to determine the ideal or maximum angle here. What would be the maximum (from vertical) angle that a shelf like this could support? I understand that there are many variables at play including the distance that the shelf hangs from the wall, the thickness and strength of the shelf material, the weight of the object on top of it, etc., but is there a rough calculation that could be used?

Answer 1

The angle is arbitrary. One can decide which angle is more practical.

However, it comes at a cost: the more the angle from the vertical the more pulling and pushing force on the anchors or escrows.

Let's say your box is 100lbs such that its center of the gravity is one foot away from the wall.

We check the pullout force on the top anchor/s which happens to be equal to the push-in force on the bottom anchor/s

We check 2 cases for the angle is 30 and 60 degrees.

For 30 degrees from vertical Fanchor is:

$ \Sigma M=0 \ \rightarrow\ 100*12 -\cos30*\mathit{F_a}=0 \ \rightarrow\ \mathit{F_a}=\frac{100*12}{0.866*12=10.39}=115.4 \text{lbs}$

For 60 degrees Fanchor is:

$ \Sigma M=0 \ \rightarrow\ 100*12 -\cos60*\mathit{F_a}=0 \ \rightarrow\ \mathit{F_a}=\frac{100*12}{6}=200\text{lbs} $

We note that the higher angle needs a stronger anchor.

We also have a vertical load on each anchor equal to 1/2 of the shear which is 50lbs, but this load is constant and independent of the angle of the diagonal brace.

Answer 2

There is no maximum angle. Theoretically a shelf could be supported solely via cantilever (a support coming straight out from the wall.

If the angle in use is the angle from the wall to the support, the compression stress on the support will be the load divided by the cosine of the angle. At straight up and down, the angle is zero, and the cosine is 1, so the force is equal to the load. As the support moves up the wall, the compression force on the support will rise (practically until the force creates a bending moment in the support for small angles). These calculations in this case are simple, but can get complex in many-membered supports. This is the basis of the Engineering Class Statics, which all Civil and Mechanical Engineering students take, usually one of the very first engineering classes taken. At its core, it is resolving vector loads (the angled support) into and out of vertical and horizontal loads using sines and cosines.

So the limits involve the strength of the support and the desired space limitations.

Answer 3

Here's a way to think about it without the math

The supporting angle is the same as a full depth bracket but with the 'unneeded' stuff removed

You need the brace because your shelf bracket is too 'skinny'. If you made the bracket deeper, how deep would you make it? That's your question in a nutshell.

BTW: I'm a structural engineer, if that makes a difference

Answer 4

What is the maximum weight for a shelf with angled braces?

With two of these it's 1000 pounds "when properly installed", which means filling all three holes with three inch exterior grade screws or better, directly into studs.

(source)

The angle looks to be bout 60 degrees. I don't think I've ever seen one any less, at least, not one that I'd install, like the ones that are stamped with a curve to provide stability and sometimes don't even have a brace.

Construction of the bracket itself and its fasteners will dictate its application. Notice how the bracket has a vertical brace and ~1/8" thickness throughout, instead of a flimsy stamped curve laying horizontal.

Maximum weight? Whatever the manufacturer says. Safety factor? As large as possible.

When I do closets with these (when the entire old system fell down because it wasn't brackets like these), it's one bracket every 16" center into a stud with 3" Deckmate screws. I don't get callbacks after installing these. And I don't install anything that you couldn't do pull-ups on, because fully loaded and grabbed while falling and injuring you isn't going to be on my conscious.

---

In what orientation should this L-bracket be when hanging shelves? : Kept as high as possible so that you don't hit your head on it and to provide the most clearance below it (as pictured above).