How to determinine the steady state accuracy (stationäre Genauigkeit) of a disturbance transfer function?

Question

Hello Engineering community,

I am currently studying Aerospace Engineering at TUM and have one module called Automatic Control Engineering. Right now, I'm just going through some practice exam questions. I stumbled upon one where I did not fully understand the answer of:

Given is the following disturbance transfer function:

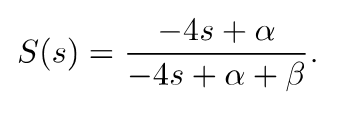
The task is to determine the constants alpha and beta so that there is a steady state accuracy (stationäre Genauigkeit) in the closed control loop.

The answer was that 1. "In order for steady state accuracy with respect to disturbance to happen, the DTF S(s) must contain a zero and therefore alpha must be zero". 2. "All poles of the system must lie in the left complex half plane for stability therefore beta < 0".

What I understood was the 2nd part. I just do not understand the first part and why S(s) must contain a zero in order for it to be steady state accurate. Can anyone help me?

Answer 1

I am assuming that the term disturbance transfer function refers to the ratio of plant output to the disturbance input and that we desire the output to be zero (or as close to zero as possible) at steady state.

According to the final value theorem, the final (or steady state) output of a signal is given by

$$ \lim _{t\,\to \,\infty }f(t)=\lim _{s\,\to \,0}{sF(s)} $$

For the disturbance input $D(s)$ and system DTF $S(s)$, the output is $D(s)\cdot S(s)$. So its final value is $\lim _{s\,\to \,0}{sD(s)S(s)}$.

If we assume a step disturbance input, then $D(s)=\frac{1}{s}$. So the final output is $ \lim _{s\,\to \,0}{S(s)}$. This will be zero if $\alpha = 0$ and $\beta \neq 0$.

The assumptions involved are

1. DTF means $S(s) = \frac{Y(s)}{D(s)}$.
2. Disturbance is the form $D(s) = \frac{k}{s}$.
3. "steady state accuracy (stationäre Genauigkeit) in the closed control loop." means $y(t)\rightarrow 0, t\rightarrow \infty$.