Is the a similar method of calculating moment in inelastic bending like in the case of torque for inelastic torsion?

Question

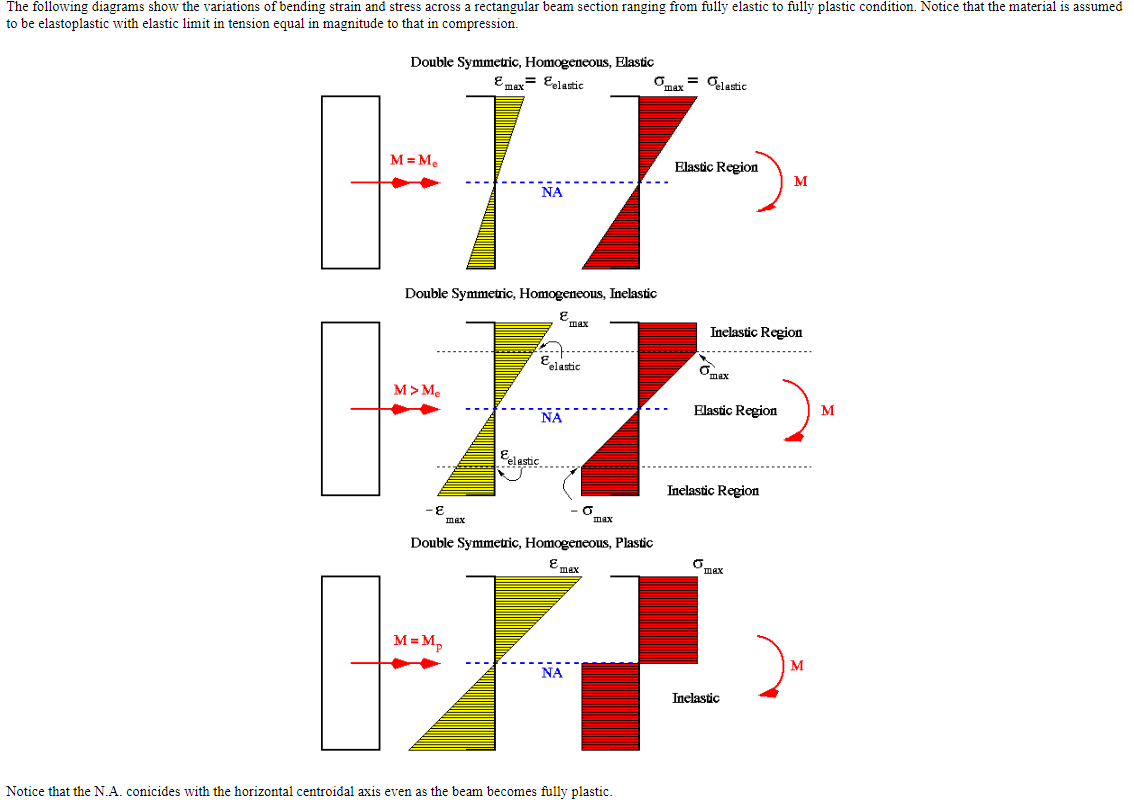
When calculating for Torque in torsion or for Moment in the case of bending, the concept is usually first introduced with the simplification of the assumption that the stresses and strains are in the elastic region of the material, appended by perfect elasto-plastic assumption of the material. And later for the same calculations in the case of the inelastic torsion the operation was to find the region upto which the material remained elastic and divvy the cross-section into two and calculate separately.

For torsion

T _Total =T _{Elastic_Region} + T _{Plastic_Region}

In the Elastic region the Elastic torsion formula is applicable and for the Plastic region the constant stress is integrated over the whole area of the plastic region. Can we do something similar for the case of inelastic bending too ?

Answer 1

Yes, it is explained here. You can take it even further and assume, that in a limiting case, the whole section will be plastic (although this cannot occur in practice, real materials may have some deformation hardening so the actual limit is even higher). The fully plastic sections can then be treated as plastic hinges.

Although elasto-plastic analysis may lead to higher load limits, not all beam sections will behave in this way. Because of this, in Eurocodes the sections are classified into 4 different classes, where the first one can develop plastic hinge, but the last one cannot. For example an I-section with very thin web and flanges may buckle before reaching plastic region.

Answer 2

In a beam, as the moment at a section increases beyond the yield strain in outer fibers, it will cause those fibers to yield. At this stage, we have the core section of the beam acting elastic and the outer top and bottom plastic, elastoplastic. But the axial strain is still linear, and the planes of the cross section remain plane.

comparable to a shaft under torsion not fully stressed to the plastic range with a core rim of elastic and an outer rim of plastic.

For this case we need to calculate the fiber at coordinate,C, where elastic limit ends and plastic starts. Then we can calculate the section moment badding the triangular elastic moment to the rectangular plastic moment.

If we kepp increasing the moment past the section plastic limit the entire cross section turns to a hing and will keep bending without any additional load.

The plastic section modulus is

$$Z_x = B(H/2)(H/4) + B(H/2)(H/4) = BH2/4.$$

'

• B width of the beam
• H height of the beam