11
$\begingroup$

Most people have had the experience of moving the tab of an aluminum can back and forth until it breaks off. It usually only takes a few complete back and forth motions before the tab breaks off.

Aluminum can with stay tab

What is the root cause of the tab breaking off?

The possible causes seem to be:

  • A fatigue fracture.
  • An overstressing of the metal.
  • A result of plastic deformation.

But which one is it?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ I'm pretty sure this is mostly to the stress-strain response of a material under cyclic loading that exceeds the yield stress, but I may have to buy a can of something on the way home to verify my thoughts experimentally. $\endgroup$
    – Trevor Archibald
    Commented Apr 9, 2015 at 20:56

3 Answers 3

Reset to default
14
$\begingroup$

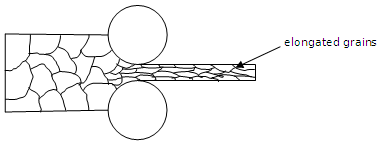
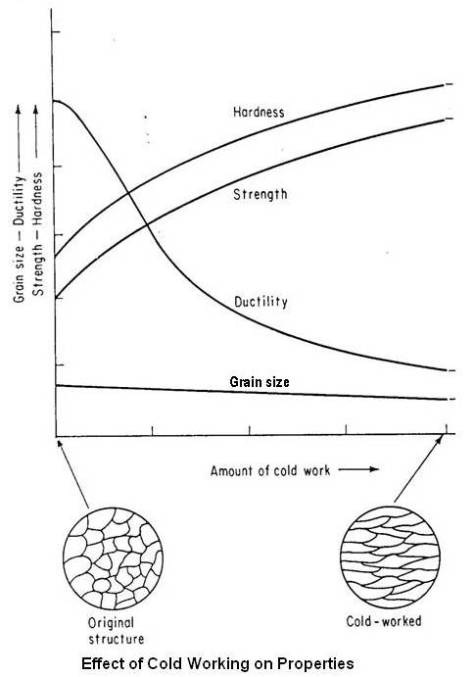
Every time you bend aluminium below the recrystallisation temperature, the macroscopic grains become smaller: this is known as cold work. The sides of the tab are either stretched or compressed. The effect is the same as the rolling example below. This is indeed a plastic deformation: the tab stays in place and doesn't bend back and stays in place.

cold work

Source

What you've just done is to make it harder for those grains to slip over each other, making the material harder and increasing it strength. You have however also made it a lot less ductile (and more brittle). If you bend a spoon, it's hard to bend it back to the correct shape. This is because that area has been cold worked and is stiffer than the surrounding metal.

graph

Source

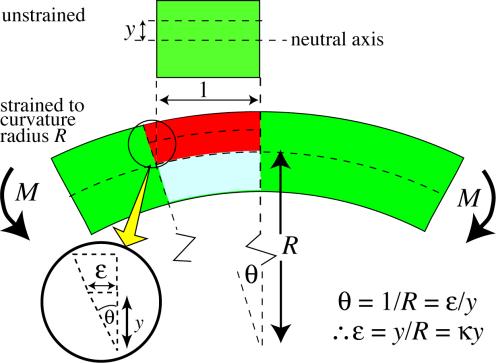
The same happens with your aluminium tab, except that there comes a point where it snaps. You can think of it like the beam below: You're bending it with a fixed deflection that corresponds to a certain angle θ. At some point as your ductility drops, you're going to go past the strain limit and it will snap.

bending

Source

While it might appear to be fatigue, from an engineering perspective is really not the correct term to use. Fatigue is used to describe problems typically appearing after several thousand load cycles.

Improve this answer
$\endgroup$
3
  • 1
    $\begingroup$ I want to +1 but I think there are a few misconceptions that need clearing up. (1) Grains do not get smaller, volume is (approximately) conserved in plastic deformation. They only change shape. (2) Grains do not slip relative to one another. Instead, atomic plains in individual grains slip due to dislocations. Dislocations build up as slip proceeds, causing work hardening, which you should mention. This causes grain shape changes. (3) Work hardening causes the increase in strength and decrease in ductility, see the wiki $\endgroup$
    – do-the-thing-please
    Commented Sep 3, 2015 at 21:12
  • 1
    $\begingroup$ (4) As plastic deformation continues past the tensile strength of the metal, necking begins. The theory behind what happens next at a microstructural level is not fully clear, but it is believed that as necking occurs, the nano-scale dislocations build up to the point where they begin forming micro-scale pores and crack initiation sites. Once this happens, the pores act as stress concentrators, creating further deformation near the pores, causing them to expand and eventually join together, or coalesce. As the pores coalesce, macro-scale cracks form. $\endgroup$
    – do-the-thing-please
    Commented Sep 3, 2015 at 21:19
  • $\begingroup$ As the pores enlarge, less material connects the two sides of the necking zone, and the stress continues to concentrate on the remaining material, accelerating deformation given constant force. You can actually feel this occur as the tab is bent back and forth. Eventually, the last connections between the two parts rupture, leading to the final macroscale rupture, and the tab breaks off. $\endgroup$
    – do-the-thing-please
    Commented Sep 3, 2015 at 21:20
1
$\begingroup$

Fatigue is the stress on a material due to cyclic loading. The best comparison is a rubber band. When you pull it over and over it stretches out because the fatigue is causing the elasticity to wear out and it becomes more and more plastic until the band snaps. While technically the the tab is breaking because of plastic deformation, fatigue fracture would be more correct be the plastic deformation doesn't cause a fracture until the tab has been loaded significantly more times than the life-cycle intends.

Improve this answer
$\endgroup$
7
  • $\begingroup$ I disagree, I don't think fatigue is really a consideration when the part lasts for 3 cycles. The cyclic loading is a factor, but it's more because the plastic deformation remains after the tab is returned to its natural position. $\endgroup$
    – Trevor Archibald
    Commented Apr 9, 2015 at 20:45
  • 1
    $\begingroup$ @TrevorArchibald we might be splitting hairs here, but what you're describing seems to be what is referred to as 'low-cycle fatigue'. $\endgroup$
    – Dan
    Commented Apr 10, 2015 at 3:22
  • $\begingroup$ That link says "low-cycle fatigue." is 10,000 cycles or fewer. 3 is in fact fewer that 10,000, but I still think we're more in the range of plastically deforming it until it breaks. Fatigue loading will still not go above the UTS or the elongation limit of the material, I think this failure mode does. $\endgroup$
    – Trevor Archibald
    Commented Apr 10, 2015 at 3:24
  • $\begingroup$ Fatigue (to the best of my knowledge) only applies when the loading is below the material's yield stress, which is definitely not the case here since it plastically deforms with every bend. $\endgroup$
    – BeyondLego
    Commented Apr 10, 2015 at 16:27
  • 1
    $\begingroup$ The magnitude of this deformation is on a totally different scale from what you would expect in a cyclic loading scenario. You can break the tab off without even performing a complete cycle. The cold working/embrittlement answer goes directly to the root cause without requiring the special case of cycling loading, so I think it's a bit better of an explanation. $\endgroup$
    – Air
    Commented Apr 10, 2015 at 18:14
1
$\begingroup$

Almost everyone is partly right. You can fail the ring-pull by simple overload in one 'cycle' or you can accumulate plastic strain in three or four cycles. This wouldn't normally be considered even low-cycle fatigue but I don't know that there's a lower limit on how many cycles Paris' Law can be applied.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.