1
$\begingroup$

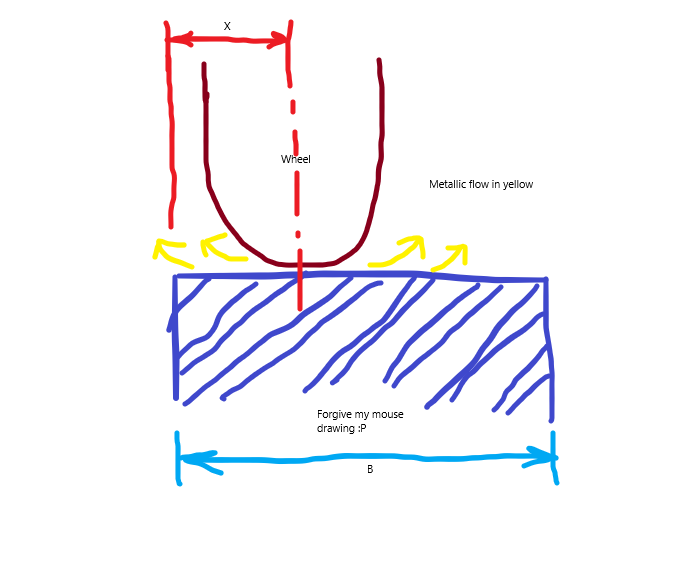
So, I did a quick search around and didn't seem to find anything regarding this, so I thought perhaps I should just ask a question.

Metals are malleable and will generally flow/deform if you roll it repeatedly. Localized concentrated loads will thus change the shape of a piece of metal bit-by-bit if exposed to repeated loading of the same/similar orientations (kind of like molding a piece of playdoh, with each repeated load only displacing a very minor amount of material). Presumably, flow should be the same in all directions of the contact point non-isotropically for a homogeneous material.

But if there is eccentricity within the load, will that still hold true? If it does not, where is that critical limit for for X:B within the figure below that will produce some degree of eccentricity with the amount of metallic flow? Or am I thinking about this in a completely wrong way? See below example for what X and B refers to, and an example of what I am trying to figure out. Thanks!

Edit: I know flow will happen. What I am trying to understand is if load is eccentric, how will that affect flow behavior? Will it cause one side to flow more than the other IN THE ABSENCE of surface shear type loads? The below example will have all vertical loads (up/down) with assumed 0 lateral loads (left/right), and if you are just rolling it back and forth, will it flow more on the side closer to the edge just because it is closer to the edge? I don't think there are any formal theories but I can see it happening that way because flow ultimately is a localized plastic deformation that is constrained by surrounding material structure. If the surrounding material structure weakens, it somewhat makes sense that it will tend to have more plastic deformation in that direction. But is there some limit as to how far X has to be from the edge for it to be negligible? It's kind of like us saying eccentric loading on a concrete shallow foundation footing is okay if it is within the middle third. Hope that makes more sense what I am asking.

enter image description here

Improve this question
$\endgroup$
6
  • $\begingroup$ Perhaps you mean an English wheel : youtube.com/watch?v=omRlIBONJAM $\endgroup$
    – Solar Mike
    Commented Nov 25, 2019 at 18:26
  • $\begingroup$ Yes, an english wheel will be a good illustration of what I am asking about. So, if you roll a piece of sheet steel close to its edge, and say, we print a grid on the sheet before rolling it, will it show more growth on the side closer to the edge and less for the other side towards the center, or will it not? Does how close the wheel to the edge makes a difference in terms whether a difference is observed or not? What about the thickness of steel affecting how such differential flow show up based where the wheel load is applied? $\endgroup$
    – Isa
    Commented Nov 25, 2019 at 20:51
  • $\begingroup$ Answered in the video stretching and shrinking forms the curve... $\endgroup$
    – Solar Mike
    Commented Nov 25, 2019 at 20:55
  • $\begingroup$ You mean the first portion? The first portion rolls the entire piece. It does not answer the question. The scenario we are looking at with respect to eccentric loading is where you have say a plate bigger than the wheel itself, similar to the embossing piece. When you roll a plate like that, and you put the wheel just 1cm from the edge of one side and 90 cm on the other side, will it cause the 1cm side to stretch more than the other side. $\endgroup$
    – Isa
    Commented Nov 25, 2019 at 21:10
  • $\begingroup$ Also see youtube.com/watch?v=L7B7lCDq7cY for another example... $\endgroup$
    – Solar Mike
    Commented Nov 25, 2019 at 21:59

1 Answer 1

Reset to default
0
$\begingroup$

Yes, it is still going to reshape and damage the wheel and the support. Maybe an example of unsymmetrical loading is a train wheel in relation to the tracks.

There has been extensive research and tests on that, to help the design the wheels and rails to lower the routine maintenance costs.

.

  • 1 Contact points

contact points

-

  • 2 Wear model of the flange.

flange wear

This is a brief but useful research on the railroad wheel.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Right, but what I am trying to figure out is will eccentric loading change how flow occur. Like, will it cause more flow on one side than the other? $\endgroup$
    – Isa
    Commented Nov 25, 2019 at 19:45
  • $\begingroup$ there are equations to estimate the flow and fatigue, abrasion and interestingly new to me debris of wear secondary effect in the paper. i recommend you read it. $\endgroup$
    – kamran
    Commented Nov 25, 2019 at 19:56
  • $\begingroup$ The paper appears to focus on RCF and SSC problems, but does not specifically have equations estimating flow or eccentric flow? $\endgroup$
    – Isa
    Commented Nov 25, 2019 at 20:47

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.