The confusion seems to arise from a misconception about how $\sigma$ is defined. For example, $\sigma_{yy}$ corresponds to a force in the $y$ direction applied to a surface facing the $y$ direction. Both $\sigma_{yy}$ and $\sigma_{zz}$ are zero essentially everywhere in the shaft (idealizing it as long and narrow) because there's no distributed region where a $y$-or $z$-direction force is internally or externally applied to a $y$- or $z$-direction surface, respectively.
(I'm not including the point loads at either end, which are localized. It's implicit in these problems that stress concentrations can be ignored and that failure doesn't arise directly in the attachment, though it may occur within the shaft adjacent to the attachment or point of load application.)
Instead, $\sigma_{xx}$ and $\tau$ are the key parameters (and the only nonzero stress components in this idealized problem), and the first varies strongly with the location on the shaft. For example, the $y$-direction component of $P$ causes a location-dependent tensile stress $\sigma_{xx}$ (i.e., locations with positive $\sigma_{xx}$) on the top of the shaft as a result of the bending moment). The $z$-direction component causes a location-dependent compressive stress $\sigma_{xx}$ on the left side of the shaft, also arising from the bending moment. The torque causes a shear $\tau$ that's maximized on the surface of the shaft.
The key point is that a single stress component is not necessarily uniform in an object; here, for example, $\sigma_{xx}$ varies across the surface and interior of the shaft.
This is a very important point, so I suggest reviewing a good mechanics of materials text (e.g., Beer and Johnston) until it's familiar.
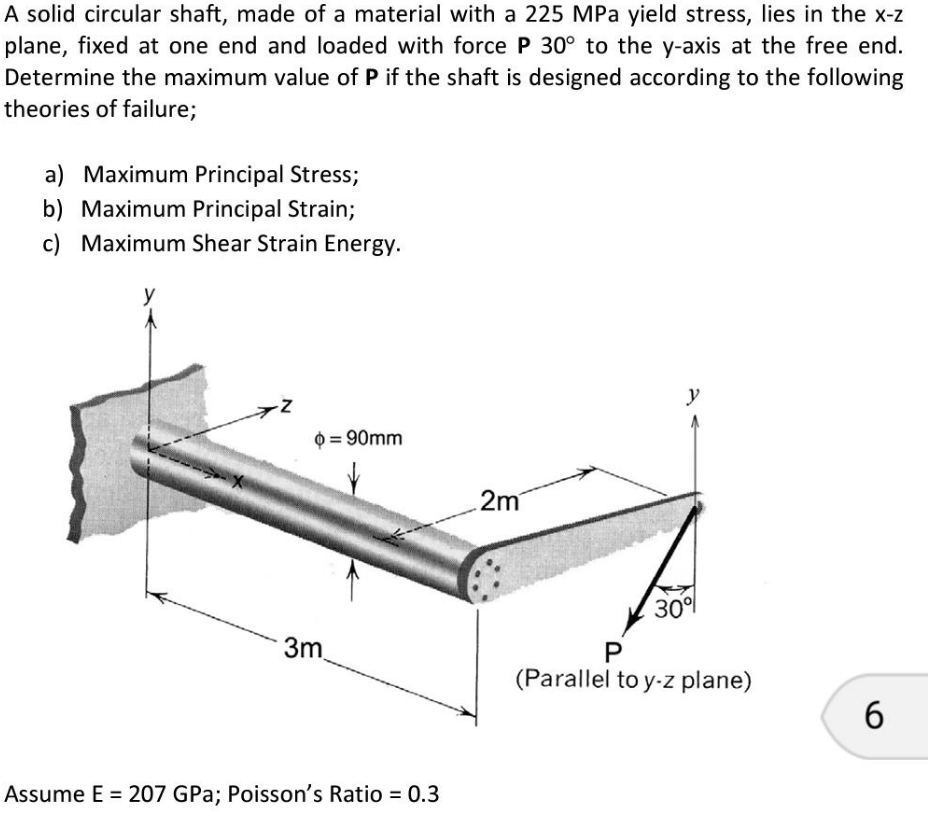
Pand torque2 P Cos(30)), then work the shaft problem in a coordinate system aligned with the vector load direction. $\endgroup$