I try to design a shaft with yet unknown diameter, i know the distribution of forces and stresses (shear, torsion and normal) in/ along the shaft. I need just one more parameter nl. the tensile strength of martial. I use Rolloff-Matek machine elements table book. In the table i choose a material with tensile stress say $1000 Mpa$, but all the values in the table are normalised for a rod with diameter $d_N=16 mm$.
May i use the tensile stress as mentioned in the tabel ? or i should find a correction factor somewhere else ?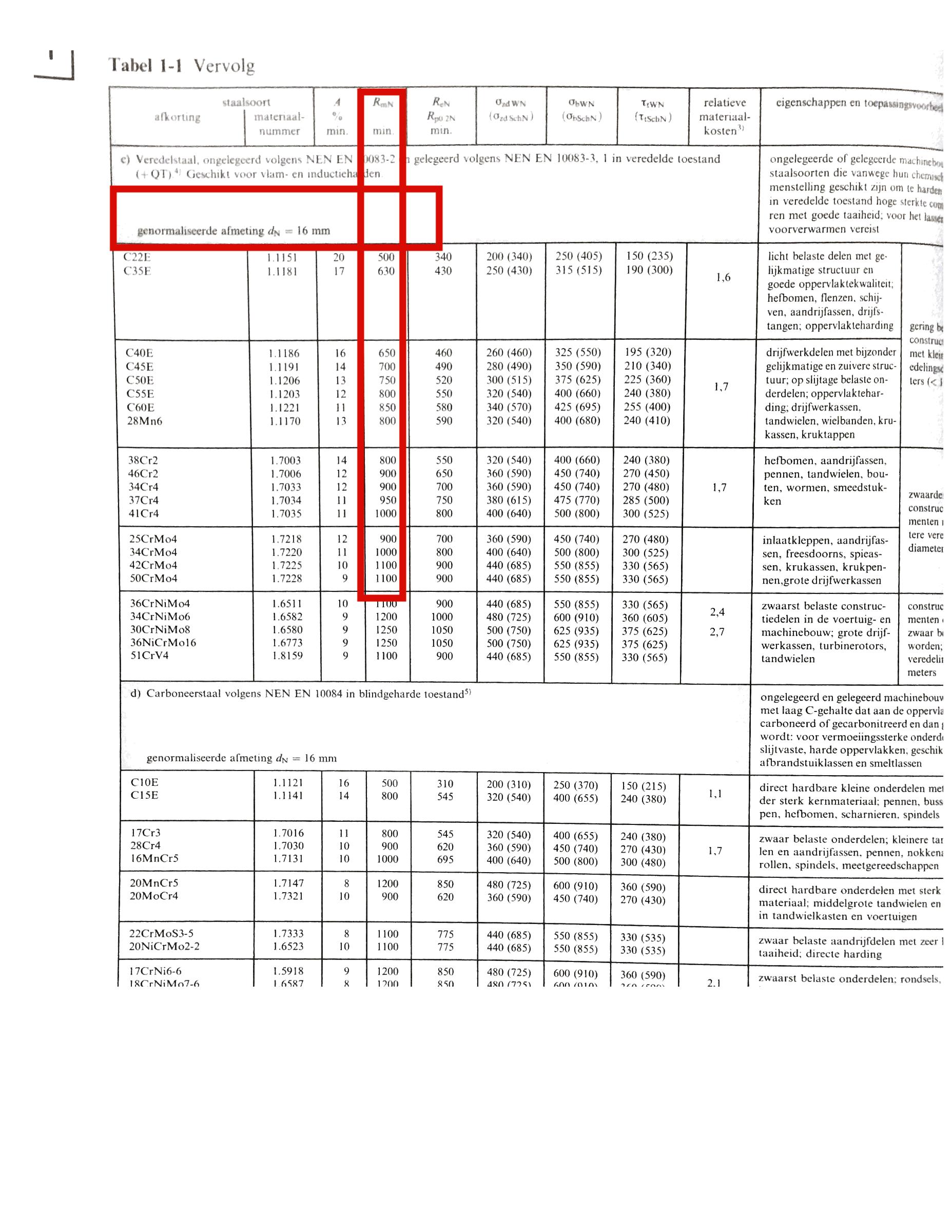 
$\begingroup$
$\endgroup$
3
-
$\begingroup$ Something lost in translation ? I see the metals are listed in Russian designations; maybe the text is also in Russian. $\endgroup$– blacksmith37Commented Mar 9, 2018 at 18:05
-
$\begingroup$ @blacksmith37 The book Rolloff-Matek is written is German, all the symbols are also in german, the translation is in dutch. The horizontal red marked text says , all dimensions are normalised d_N = 16 mm, by mm means millimetre $\endgroup$– user14407Commented Mar 9, 2018 at 20:34
-
$\begingroup$ My mistake , at a glance GOST look similar to DIN to someone not using them. "Normalize" is a common steel heat-treatment , but I don't see how it fits your question. $\endgroup$– blacksmith37Commented Mar 10, 2018 at 4:17
Add a comment
|
2 Answers
$\begingroup$
$\endgroup$
1
There are correction factors in academic table books. A very detailed explanation is in the "FKM Richtlinie" chapter 1.2 material characteristic.
But you mentioned that you have the book Roloff / Matek:
- Look at formula 3.7: $R_m=K_t*R_{mN}$ and $R_p=K_t*R_{pN}$
- $K_t$ is the technological size influence factor – not sure if this is the right translation
- And $K_t$ you can find in your table book. Table 3.11 a) and b) depending what kind of metal you are using
- The values of $R_{mN}$ and $R_{pN}$ are given in your already shown table. I guess you picked 41Cr4, then $R_{mN}$ is 1000 MPa and $R_{pN}$ is 800 MPa
- 41Cr4 is a metal out of the group “heat treatable steel” – so you have to take table 3.11 a) graph 3
- Now you take your desired diameter, say 30 mm, and then you get $K_t=0.93$ or calculated with the formula $K_t=1-0.26*lg(30/16)=0.929$
- Put $K_t$ in the formulas above you get $R_m=930 MPa$ and $R_p=744 MPa$
An additional hint also concerning the comments: the $N$ in $d_N$ has nothing to do with the normalization of metal (the heat treatment)! $N$ stands for standard part size. In your table (nearly) all metals are tested with $d_N=16 mm$ to have comparability.
-
$\begingroup$ Later after i asked this question, i found the exact same thing in my book, but as my book is a translation from German to Dutch, the translator didn't give any explanation about parameters thank you for your explanation. $\endgroup$– user14407Commented Oct 19, 2018 at 13:27
$\begingroup$
$\endgroup$
The tensile strength is diameter dependent. How greater the diameter, how longer it takes to discharge the heat, so, the time it takes to cool down the material (here is steel) has an impact on the microstructure and consequently the mechanical characteristic of the material.
The tensile strength would be lower than what is already mentioned in the table, because the shaft has a bigger diameter than $16mm$.
The correction factors are located at the manufacturing catalogues and not in academic table books.