0
$\begingroup$

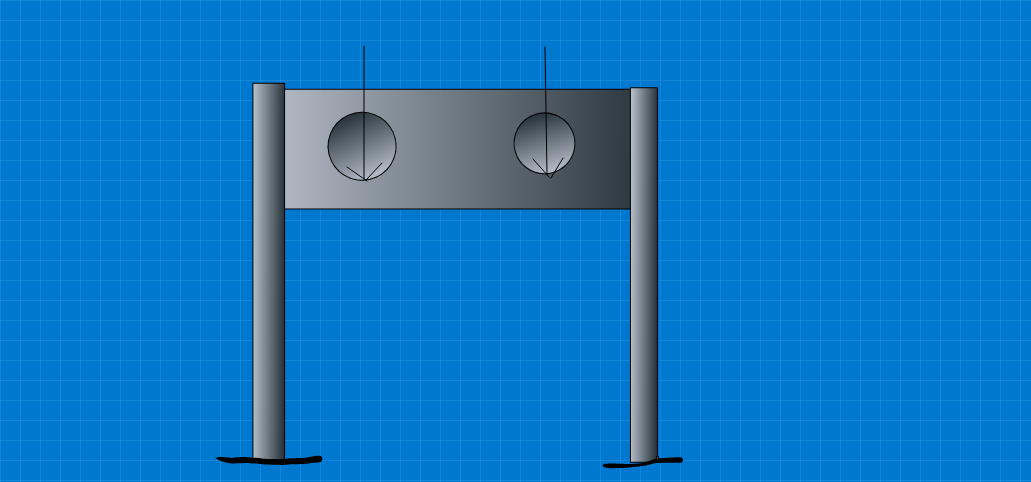
I have a structure that is used to support two shafts which are carrying relatively high loads. It is composed of two columns and a "shaft supporting block" in the centre (almost acting as a beam). The block is just a rectangular block of steel with two through holes in the centre. I want to analyse the stresses in the columns and block in order to verify if they are suitable and do not yield or fail. I need a relatively accurate solution and so I want to avoid approximate methods. The loads act at the bottom of each hole, in the centre of the thickness and therefore torsion is negligible or can be considered as 0.

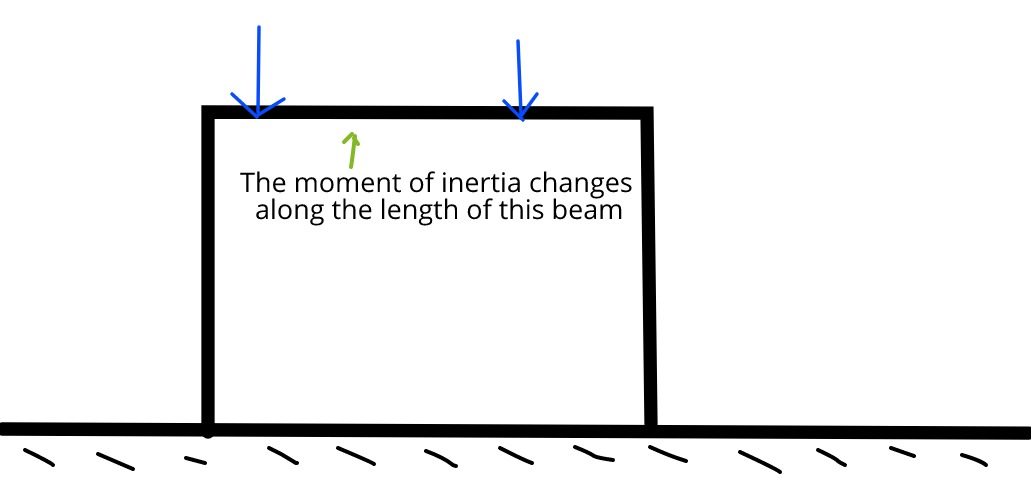
My first thought was to idealise the shaft supporting block as a beam and apply the moment distribution method. However, due to the two holes for the shafts to pass through, the block has a varying moment of inertia and therefore cannot (at least in my understanding,) be solved using moment distribution.

I also thought of trying the column analogy method however, did not work due to the same above reason.

I do not have access to use finite element analysis (computer) and want to use standard structural analysis methods (e.g. moment distribution and not stiffness method). I have attached a rough sketch image of the real structure and idealised system for reference. Idealised Structure Actual Structure

Improve this question
$\endgroup$
6
  • $\begingroup$ Is the drawing of the holes to scale? That is, is the diameter of the holes almost half the height of the beam? $\endgroup$
    – Wasabi
    Commented Jul 20, 2019 at 19:15
  • $\begingroup$ Yes approximately. By the way, the above sketch is just a rough image for reference of what the system looks like. The dimensions are not to scale. $\endgroup$
    – Amit
    Commented Jul 21, 2019 at 13:30
  • $\begingroup$ Ok, your two phrases contradict each other a bit. To clarify: the image isn't perfectly to scale, but the holes really are huge compared to the height of the beam, correct? $\endgroup$
    – Wasabi
    Commented Jul 21, 2019 at 14:30
  • $\begingroup$ Yes, however, if required I can increase the size of the beam however the hole size is fixed. I just want to understand which method to apply in order to analyse the structure. $\endgroup$
    – Amit
    Commented Jul 22, 2019 at 3:14
  • $\begingroup$ How free are you to increase the size of the beam? Also, what is the beam made of? Concrete or steel? $\endgroup$
    – Wasabi
    Commented Jul 22, 2019 at 13:43

3 Answers 3

Reset to default
0
$\begingroup$

This is an indeterminate structure, meaning the moments and shears can not be determined by just the loads and reactions.

you need the detailed measurements, thicknesses, the radius of the holes and define the posts as to their stiffness and dimensions and define supports at the base, whether they are roller, pin or fixed.

However assuming pin supports and assuming your sketch is to scale, by inspection, it can be assumed two cantilever beams at the two sides imparting compression to the top half of the middle part and tension to the lower half.

then you can solve the frame by Hardy Cross moment distribution method.

here is the Wikipedia link,Wiki moment

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thanks for your response. Assuming that all dimensions are known and all joints are fixed, how would I analyse the structure? And also, as I asked above, since the stiffness in moment distribution method (same as the Hardy Cross method) is equal to 4EI/L, how do I get around the moment of inertia changing in the beam (shaft supporting block) due to the holes? $\endgroup$
    – Amit
    Commented Jul 20, 2019 at 8:29
0
$\begingroup$

Without access to FEM program, you have to refresh or learn the "Column Analogy Method" developed by Prof. Hardy Cross. Here is a paper provides the basic for using such method to find the fixed end moment, carry over factor and distribution factor, that all are required for solving indeterminate beams with the varying moment of inertia using the moment distribution method.

Albeit this paper was written for the author's computer program, nevertheless it is a good start point. PRINCIPLES OF THE COLUMN ANALOGY METHOD

Also, please keep in mind, depends on the depth-span ratio, the beam might be classified as a deep member, given your situation, it would be very difficult to get results with high accuracy without using FEM or classic advanced analytical method. You can try to use the "truss analogy" that is considered more appropriate for problems involving deep members.

Improve this answer
$\endgroup$
0
$\begingroup$

You can solve it from first principles. Assume the beam is pin jointed to the vertical poles. This is conservative.

first construct a bending moment diagram

Then M/I=E/R=s/y

s being the stress at the extreme surface of the beam If you want the deflection

1/R=the curvature=d^2y/dx^2

Piecewise integrate along the length of the beam. Excel should do it.

If the poles are sufficiently stout then you may want to account for them, if your proportions are accurate then eyeball FEM says it won't make much odds.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.