Why does a simple pin-ended triangular (arched/curved) beam deflect more due to secondary effects (non-linear analysis)?

Question

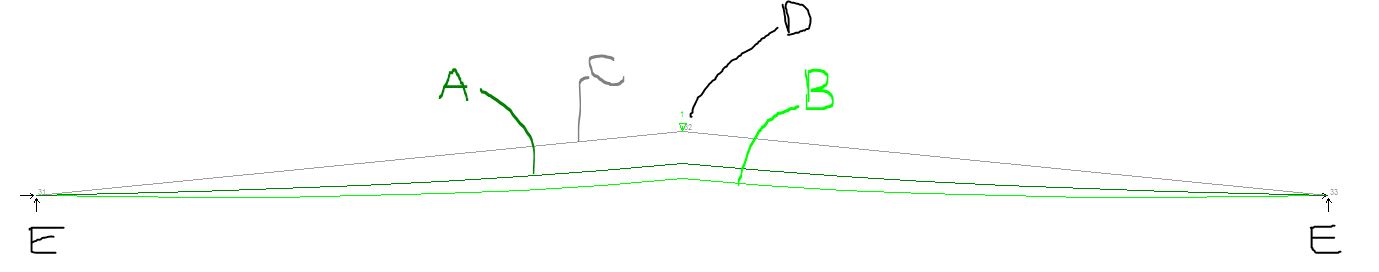
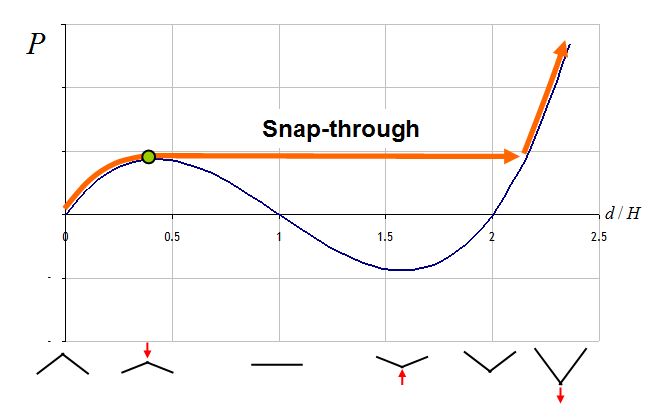
I cannot wrap my head around this.

I have made two simple models, a triangular (or curved or arched) beam that is pin ended and has a single point load of 1N downwards at it's center, and a flat beam with the same loading/supports.

The secondary effects response for the flat beam makes sense to me, It looks like this: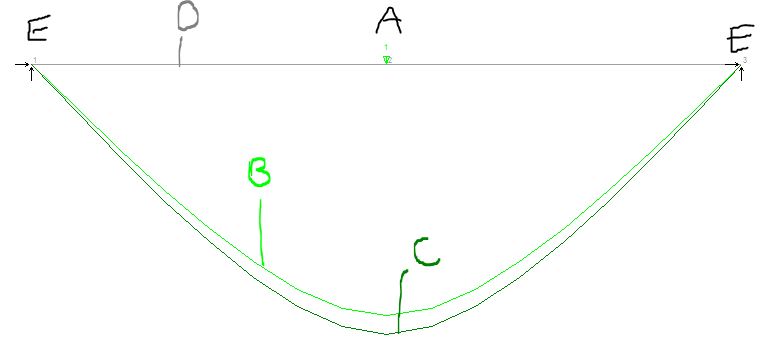

a) Point Load (1N)
b) Deflection using a non-linear (secondary) analysis (Light Green)
c) Deflection using a linear analysis (Dark Green)
d) Un-deformed structure
e) Pin supports

Linear analysis: The beam deflects, tension is formed in the beam
Non-Linear Analysis: The tension in the beam "pulls up" the beam so it deflects LESS.

So then, why does the curved beam deflect MORE due to secondary effects? I would assume that since the load creates compression in the members, the secondary analysis would reduce deflection and push the beam upward.

a) Deflection using a linear analysis (Dark Green)
b) Deflection using a non-linear (secondary) analysis (Light Green)
c) Un-deformed structure
d) Point Load (1N)
e) Pin supports

Answer 1

Play around with a simple version of this structure, made from a sheet of paper fixed in a slight curve, and see what happens when you apply a load to the mid point.

If the first example, any deflection of the beam will increase its length, which creates more stiffness that is included in the nonlinear analysis but not in the linear one.

In the second diagram, you can change the shape of the beam by making it more curved at the ends and flatter in the centre, without changing its length. In fact if you apply more load, the stiffness will become zero and the beam will suddenly deflect into a curved shape with the center below the end points, not above them. After that has happened, the stiffness will start to increase, but if you remove the load the beam won't return to its original shape.

That type of behaviour is often called "snap through buckling," and it is usually a bad thing if it happens to a real structure, for the obvious reasons. You often get this behaviour if the lid of a tin can is not quite flat. The lid has as two stable states, bent in opposite directions, and the sudden displacement of the air makes a loud noise if it flips from one state to the other.

Answer 2

The answer of alephzero is spot on. I just want to mention that the arch structure is particularly sensitive to 2nd order effects when it is shallow (say depth to span ratio ~0.1) . It's not acceptable to model it using 1st order theory. The figure below demostrates the type of behaviour expected (load vs vertical displacement curve) for an arch with pinned supports and pinned apex.

• The blue curve is the response when you apply displacement
• The orange curve is the response when you apply load

The small figures below the graph, indicate the deformed shape and applied load to the arch, at the respective curve points (of the blue curve).

As you can see, the stiffness of the structure decreases initially as the bars tend to become horizontal. When they become horizontal there is no way to carry the vertical load (except for bending, but it's not allowed due to the pinned apex), therefore stiffens becomes zero and the structure snaps under load control to an opposite geometry which is more stable.

On the other hand when performing linear analysis, no change in stiffness is considered. Therefore, since initial stiffness is the highest one, it is expected to find lower displacements.