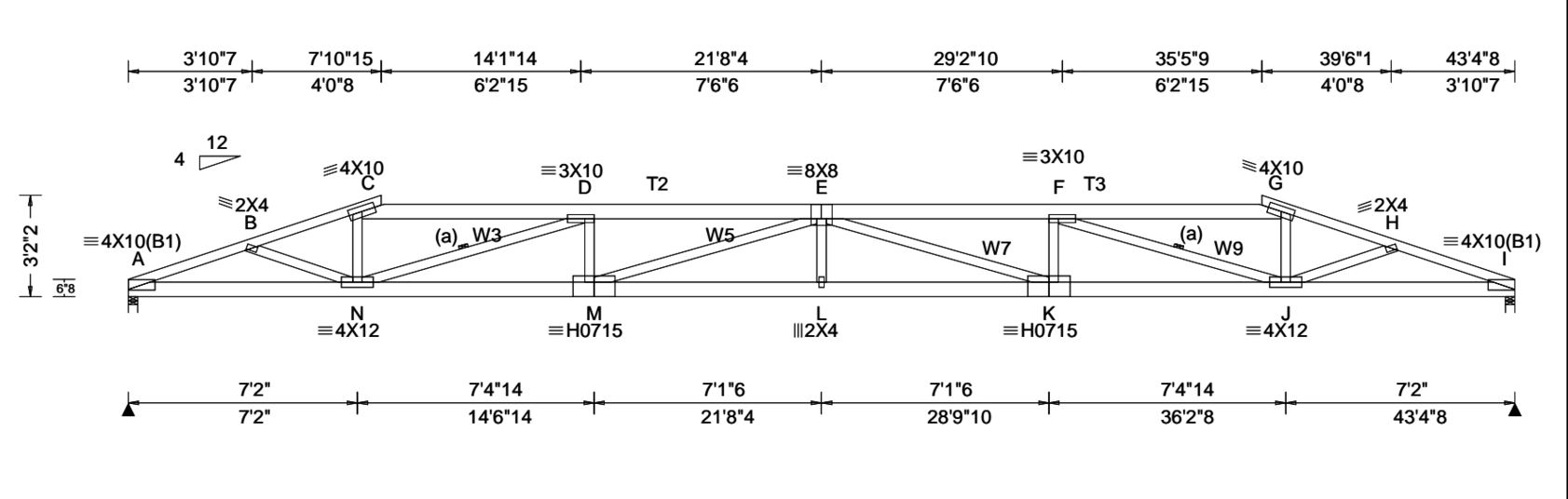
The most common way to model this structure is as follows (ignore the fact that the proportions are a bit off):
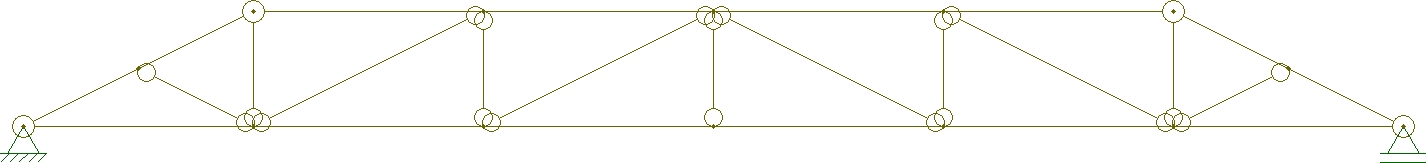
So, all of the diagonals are pinned-pinned. You'll notice in the schematic, however, that the chords (including the diagonals from the supports to the top chord) are not segmented at every point of intersection with the diagonals. Indeed, the chords are probably as long as possible, presenting splices at points E, J and M simply due to constructability demands (usually defined by the size of a standard truck). These splices between beams composing the chords, however, are usually designed so as to behave as a fixed joint, which is why I didn't put pins anywhere along the chords themselves.
Regarding the loads, you can just apply them as distributed loads. Since the individual member spans are quite small, almost no bending will occur and the vast majority of the internal forces will be axial regardless. Some analysis programs do allow you to apply distributed loads but set that they should be transformed into nodal forces, but there's really no need. And hell, the real truss will have that tiny bending, so you're more than welcome to include it in your analysis if you want.
The exception of course are the chords. Since they are not pinned at every joint, they will suffer significant bending, given that the diagonals will "deposit" their axial loads as concentrated nodal loads along the chords' effective spans.
And finally note that obviously the supports are different: one allows for horizontal displacement while the other doesn't.