1
$\begingroup$

I am analysing the forces within a certain frame, and for some reason there do not seem to be sufficient equations of equilibrium to solve for all the internal forces. Is there something about this structure than makes it unsolvable without more information?

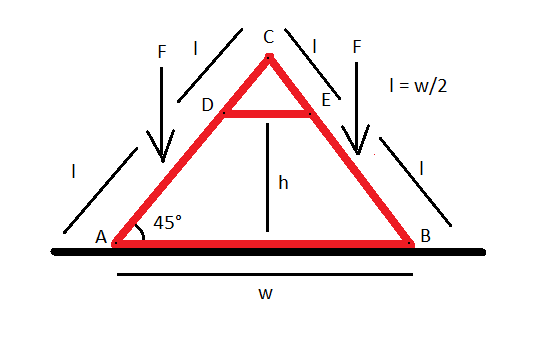
There is a frame, ABCDE, shown below. Two equal forces are applied to it (both shown as F). Assume we know F, w, and h (and from the illustration, l = w/2). Let's try to find the forces within member ADC.

Frame illustration

First, we can analyse the frame as a whole. It is straightforward to show that Ay = By = F.

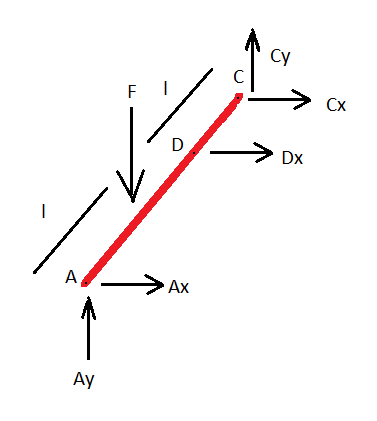
Next, we should be able to analyse member ADC by itself.

Member ADC

If we sum forces in the y-direction, we get:

Ay + Cy - F = 0

Since we already found that Ay = F when we analysed the whole frame, we know that Cy = 0

If we sum forces in the x-direction, we get:

Ax + Dx + Cx = 0

And if we sum moments around A, we get:

F*w/4 + Dx*h + Cx*w/2 = 0

Without going any further, we can see that we have two equations with three unknowns (Ax, Dx, and Cx).

If we look at the other members in the frame, we don't get any new information, I don't think... So what's missing? How can this simple frame be an unsolvable physical conundrum?

Improve this question
$\endgroup$
7
  • $\begingroup$ Ax + Dx + Cx = 0 is only true if all forces are 0, since all are pointing in the same direction. I believe you have to analyse the structure as a whole. $\endgroup$
    – Koo Zhengqun
    Commented Jul 27, 2017 at 1:58
  • $\begingroup$ Are you sure that the two members ADC and BEC are supposed to be connected to each other at point C? If they are not connected at point C it is intuitively apparent that this is a well described problem with a unique solution. The reason I'm suspicious about there being a connection at point C is that if you consider members ADC and BEC to be 'real' beams rather than ideal infinitely stiff beams then the force at point C (as well as points D and E) will depend very sensitively on the actual real stiffnesses of the ADC and BEC beams, and that normally isn't the case with these sorts of problems $\endgroup$
    – Barney Cowell
    Commented Jul 27, 2017 at 2:03
  • $\begingroup$ Is not Ax=0 due to DE? Imagine AB is a spring, it will neither compress or expand $\endgroup$
    – Willy Billy Williams
    Commented Jul 27, 2017 at 5:06
  • 2
    $\begingroup$ This is an example of a statically indeterminate system which can sometimes be solved by considering small displacements. ocw.mit.edu/courses/civil-and-environmental-engineering/… $\endgroup$
    – Farcher
    Commented Jul 27, 2017 at 5:48
  • 1
    $\begingroup$ @KooZhengqun If the forces are not in the direction indicated by the arrows, they will just be negative. No big deal. $\endgroup$
    – PProteus
    Commented Jul 27, 2017 at 17:32

1 Answer 1

Reset to default
2
$\begingroup$

It's statically indeterminate. It's far from unique - most real world structures are.

Trivially / intuitively, the frame 'works' if member DE is omitted (ie, you could take it out and the frame still stands up), so we can say immediately that there's a valid solution with member DE having zero axial force.

Also, we can see that if you put a tension into that member (for example, if it's slightly too short and you stretch it to fit it in place) the frame would still work. Thus, there's a valid solution with member DE having a non-zero axial force.

To analyse it, you need to know more. The normal assumption would be that the members are all unstressed if no loads are imposed (ie, if the members are weightless and F is removed, there's no member actions anywhere). Then, if you know the relative stiffness of the members you can work out to what degree the forces distribute. If DE is very axially compliant and AC and CB are very flexurally stiff, the frame would tend towards acting as if DE wasn't there - DE would have a very low force. If DE is very stiff, and the sides are relatively flexible, then DE would tend to prop the sides apart and pick up lots of load.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks! That's a great clarifying answer. I was hoping to be able to do the calculations without investing in software, but it seems like approaches to this sort of problem are basically just ways of doing numerical methods by hand. Do you know of a reasonable way to analyse these structures by hand, or alternately, is there a software program you could recommend? SAPY looks promising... $\endgroup$
    – PProteus
    Commented Jul 28, 2017 at 9:15
  • 1
    $\begingroup$ @PProteus - I have seen many people on this site use SkyCiv in their answers as it is free software. I have no experience with it myself. $\endgroup$
    – AndyT
    Commented Jul 28, 2017 at 9:20
  • $\begingroup$ I tried it out briefly, and the functionality of the free version seemed quite limited. $\endgroup$
    – PProteus
    Commented Jul 28, 2017 at 14:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.