0
$\begingroup$

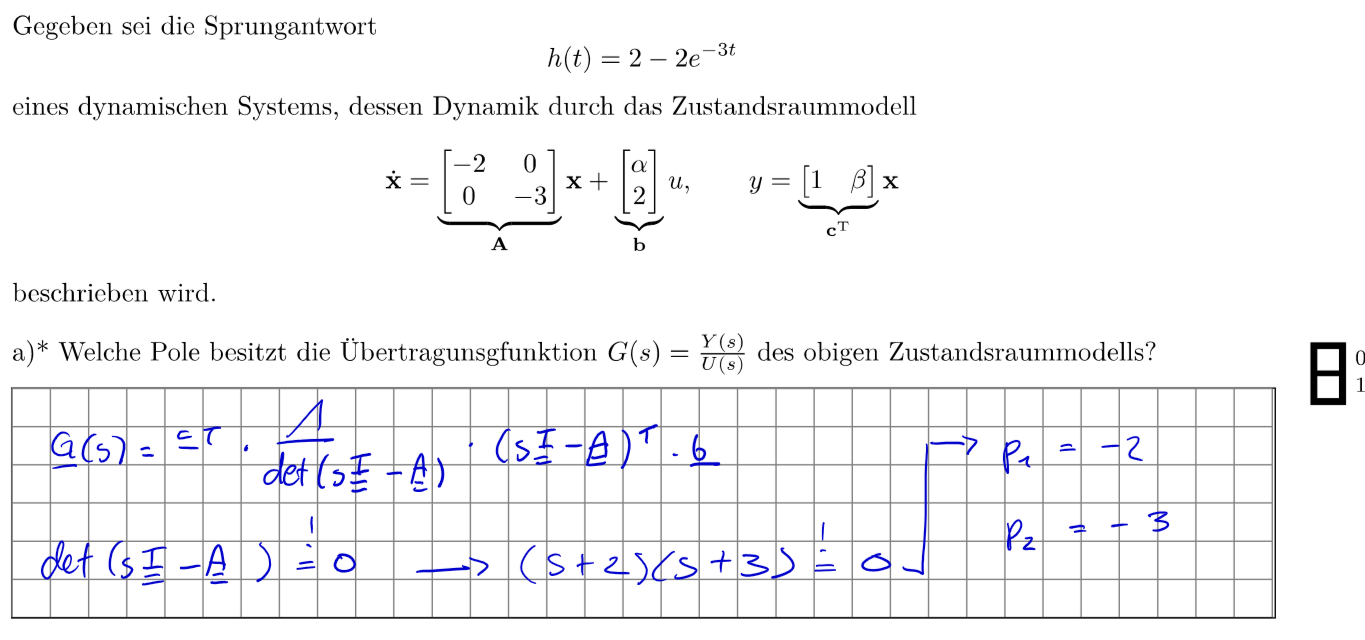
I have a question regarding the poles of a transfer function given a state space diagram. There is a formula for it. This is my answer:

enter image description here

Unfortunately, the answer is apparently long. The answer says that the pole of the transfer function G(s) is located at -3. So it doesn't mention the -2.

Anyone know where I went wrong?

Translation of question :

Given is the step response h(t) of a dynamic system whose dynamics are described by the state space model. Which poles does the transfer function G(s) = Y(s) / U(s) have?

Improve this question
$\endgroup$
3
  • $\begingroup$ Could you translate the question in the screenshot to English? There could be some property of $\alpha$ and $\beta$ which "cancel" the pole at -2 with a zero at -2. What is $h(t)$? if it is something like impulse or step response and it contains only $e^{-3t}$, I strongly suspect that there is a zero involved in the transfer function. $\endgroup$
    – AJN
    Commented Nov 25 at 15:34
  • $\begingroup$ is $G(s) = \Lambda / \det {(sI-A)}$? What is $\Lambda$? $\endgroup$
    – AJN
    Commented Nov 25 at 15:37
  • $\begingroup$ So this is the translation: Given is the step response h(t) of a dynamic system whose dynamics are described by the state space model. Which poles does the transfer function G(s) = Y(s) / U(s) have? $\endgroup$
    – CheesyBeqa
    Commented Nov 25 at 19:13

1 Answer 1

Reset to default
0
$\begingroup$

Let the system be $H(s)$. Laplace transform of the step response is $$ \begin{align} \frac{1}{s}\cdot H(s) &= \mathscr{L}\left(2-2e^{-3t}\right)\\ &= \frac{2}{s} - \frac{2}{s+3}\\ \implies H(s) &= \frac{6}{s+3} \end{align} $$ The above system has a pole at $s=3$.

Moreover, if you find the expression for the transfer function from the formula $C\cdot (sI-A)^{-1}\cdot B$. You will be able to note that for certain values of $\alpha$ and $\beta$, the pole at $s=2$ is cancelled by a zero at $s=2$. I am leaving that unsolved since this looks like a homework question.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Thank you so much for this answer. I realize as well that for some values of alpha, one of the poles ( in this case 2 ) will cancel out. $\endgroup$
    – CheesyBeqa
    Commented Nov 26 at 15:03

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.