I'm trying to calculate the amount of torque I need to put into my braking mechanism in order to hold up a weight. The weight is 18kg at the end of a 170mm arm. this should result in an approx. 30Nm torque on the arm. Now I have two break pads on either side of the arm (outer diameter 40mm, inner diameter 12mm) They are pressed against the arm via a M10 bolt. The coefficient of friction is 0.2. What torque do I have to put into the bolt for the brakes to hold up the weight? I cannot find a simple formula for this online so if you could provide this and explain the calculation it would be great. Thanks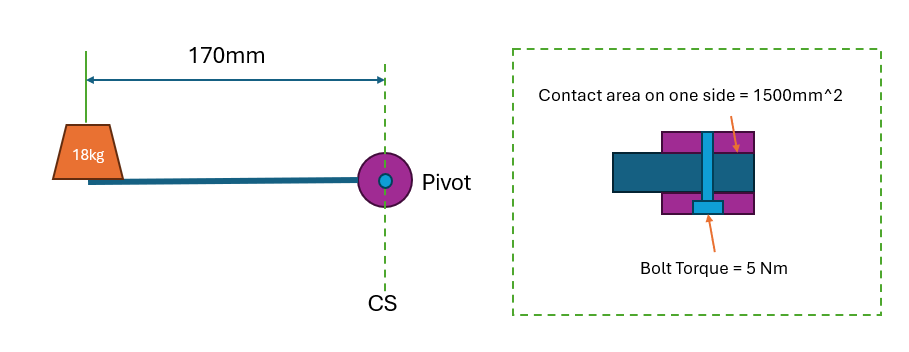
-
$\begingroup$ you need pads against the round thing, not the arm. Then you can balance the moments to find the friction force, then get that force via calcs that turn screw torque into holding force. $\endgroup$– Tiger GuyCommented Jul 8 at 14:40
1 Answer
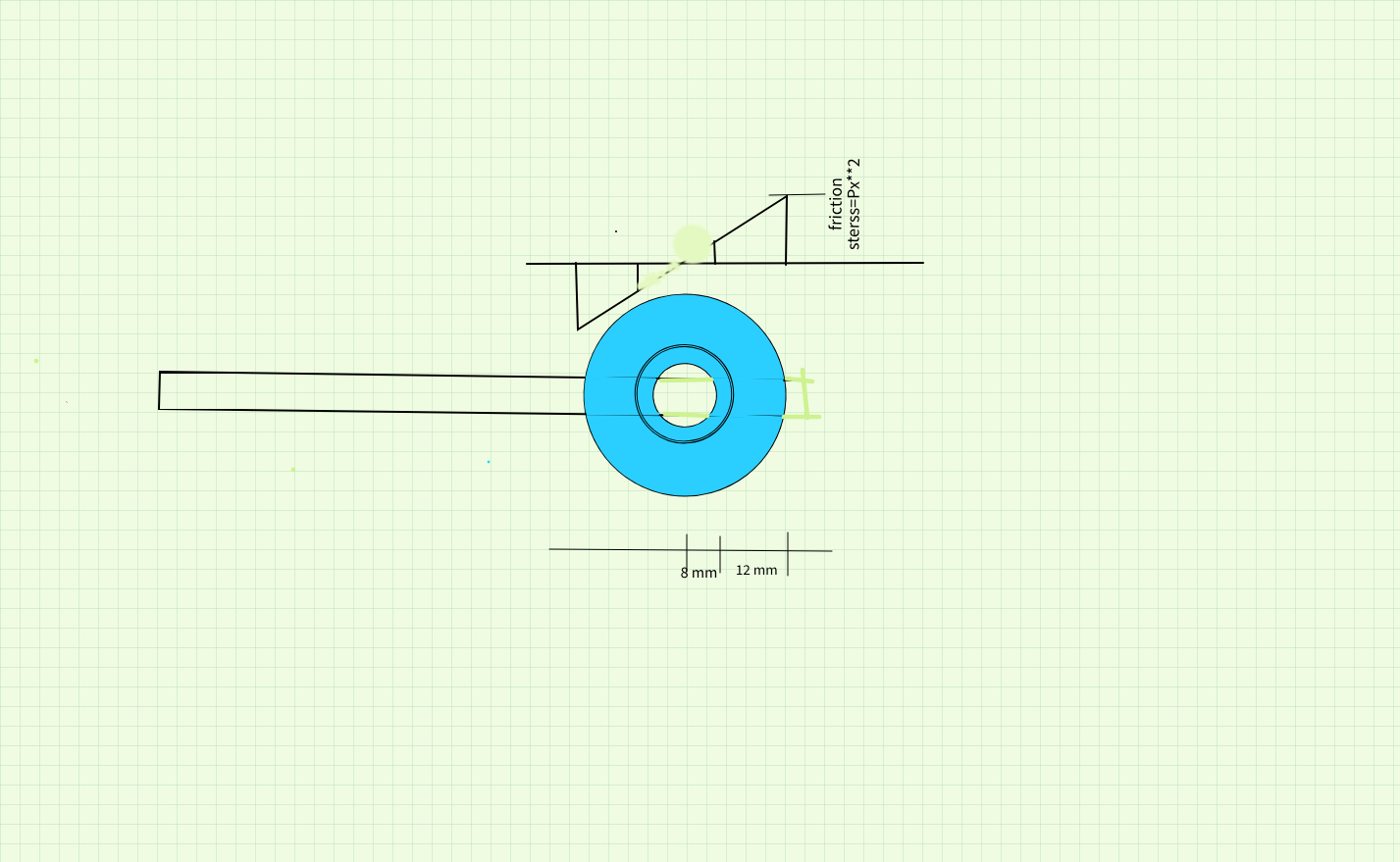
Assuming your arm is attached to a round disk sandwiched between the two friction pads $A=1500mm^2$ and assuming the brake pads are attached via a pair of washers to the $M10$ bolt, we calculate the friction on the one pad and then multiply by two. on my attached diagram.
We calculate the friction stress and friction force on a differential ring shown in the middle of the pad. Assuming the pads are rigid,
$$\sigma_{pad}= \frac{tension in the bolt*0.2}{area of contact}=P$$
Toque of the differential ring $d\tau \ $ with a width $dr$
$$d\tau_{differential- ring}= \int_{6}^{20} 2\pi rPr dr$$ Then we integrate to get the torque of one disk and multiply by two.
$$ \tau_{one-disk}=2/3P\pi r^3 \biggr\rvert _{6}^{20}$$
From the above, we can calculate P and the tension required in the M10 bolt.
From there, depending on the pitch and friction of the bolt, we can calculate its torque.
'