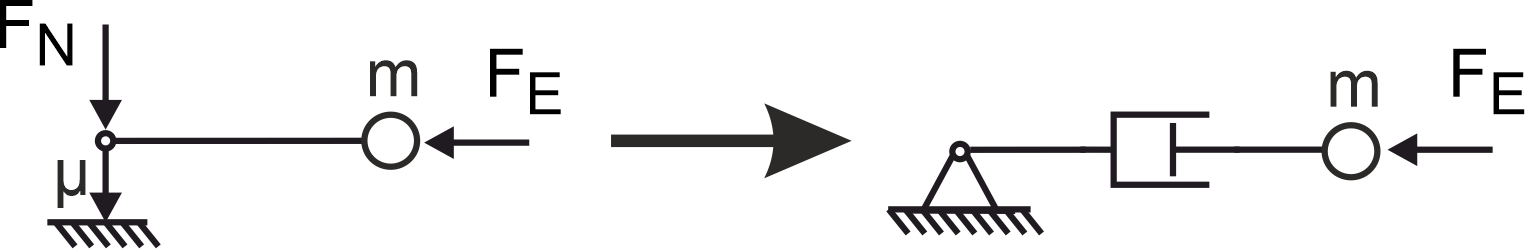
In general this is impossible, because for a given value of $F_e$, the friction damper dissipates a fixed amount of energy per cycle of vibration independent of the vibration frequency.
This is (nonlinear) hysteretic damping, not (nonlinear) viscous damping.
The only way to approximate this with a viscous damper would be to make $C$ a function of both the $F_e$ and the frequency $\omega$, which won't produce a useful equation of motion except in the special case where the machine only operates at one fixed frequency $\omega$.
Aside from that issue, a general way to make the approximation is to model one cycle of the stick-slip motion of the friction damper and find the energy dissipated during the cycle. Then choose $C$ to dissipate the same amount of energy.
For a simple slip-stick damper you can do this from first principles, though the details are messy, and you need the complete equation of motion of the system - you haven't specified how the mass and/or stiffness are connected to the damper.
A more general approach is to use the so-called Harmonic Balance Method to produce a numerical approximation. There are many variations on the basic idea (and many research papers describing them!) but one implementation is the NLVib function in Matlab.