Hi I read this response but it isn't exactly my situation I have a circle where the axis of rotation is on the c axis like this for a segment equal to the green.
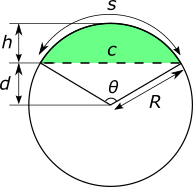
Specifically, I have a round steel plate on a concrete base attached with anchors. There a moment across the center (out of plane) I am trying to determine the resisting moment of the concrete surface.
I am very much not sure what the integration result would be.