1
$\begingroup$

My understanding so far is that a jump occurs when the Froude Number $\left(Fr=\frac{v}{\sqrt{2gh}}\right)$ of the radial flow falls with a decreasing velocity due to friction and viscosity; and the jump occurs at the critical point where $Fr=1$. However, I also learnt that the height of the jump is dependant on the difference in $Fr$ before and after the jump which contradicts the former theory where there is no difference.

Could somebody please explain and help correct my understanding?

Sources

http://thatsmaths.com/2014/01/09/white-holes-in-the-kitchen-sink/ https://en.wikipedia.org/wiki/Hydraulic_jump#Tabular_summary_of_the_analytic_conclusions

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ What 'former theory' says there is no difference in Froude number across a jump? $\endgroup$
    – Carlton
    Commented Sep 29, 2015 at 14:25
  • $\begingroup$ @Carlton The first link in the sources states that the hydraulic jump occurs at the critical point as it "slows to a critical speed where a circular hydraulic jump... forms" (I assume critical speed means Fr=1). From this, assume that the Froude number before the jump = 1. I thought a difference in Froude Number requires that the Fr immediately before a jump >1 as seen in the Wikipedia table. $\endgroup$
    – MadCommy
    Commented Sep 29, 2015 at 14:38
  • $\begingroup$ The way I interpreted it is that the Froude number is steadily decreasing as the water flows outward, due to the decrease in flow velocity. The velocity is at a maximum where the water impacts the sink, and only decreases from there on out. The decrease is partly due to friction, but also due to the increase in flow area. The jump occurs where the value of the Froude number crosses 1, i.e. where the speed of the surface wave is equal to the flow velocity. $\endgroup$
    – Carlton
    Commented Sep 29, 2015 at 14:46
  • $\begingroup$ @Carlton That was my initial understanding too, yes. But the Wikipedia table (linked) shows suggests that, with an upstream flow ≤ 1.0, no jump will occur; and the "Ratio of height after to height before jump" is proportional to the "prejump Froude Number". So I question how a hydraulic jump can occur with a prejump Fr of 1 (or slightly higher); or are you saying that the Froude number jumps down from 1 to something like 0.7? The wiki article seems to suggest that it jumps at a high Fr (eg. 1.5) down to a low Fr (e.g. 0.4) or something like that. $\endgroup$
    – MadCommy
    Commented Sep 29, 2015 at 14:54
  • $\begingroup$ Oh, yeah, the upstream Fr has to be >1 for a jump to occur, otherwise the surface wave will always outrun the flow and any buildup of fluid height would be immediately dissipated.. I think the Wikipedia table is trying to show this by saying the height after/height before ratio is 1.0: that is, no change in height. Also note the comment below the table about it being a "very rough" classification. $\endgroup$
    – Carlton
    Commented Sep 29, 2015 at 15:04

2 Answers 2

Reset to default
2
$\begingroup$

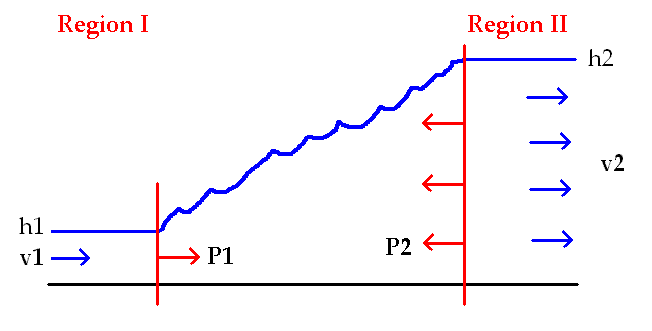
Below is a diagram of hydraulic jump that I found on Google Images that shows all the key elements. In "Region 1", $Fr > 1.0$ and in "Region 2", $Fr < 1.0$. In between these two regions, the Froude number is transitioning from one value to the other. If the upstream Froude number is increased, the ratio of fluid height before and after the jump will increase.

By the way, this diagram is for one-dimensional flow; The sink problem is a little different because it's two-dimensional; the cross-sectional area of the flow is increasing as the flow moves outward.

enter image description here

I also found this image, which shows another important feature: the flow leading up to the jump is steadily decreasing in velocity and increasing in height. These two events are what drive down the Froude number to the point that it falls below 1.0.

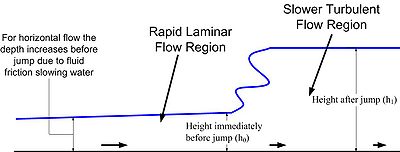
enter image description here

So, how does the jump start in the first place? Look at the second image; even before we get to the jump, the height of the fluid downstream is slightly higher than the fluid upstream. This causes the fluid to want to flow upstream, against the flow. More specifically, there is a surface wave trying to flow upstream. It can't flow upstream, however, because its velocity is slower than the fluid velocity. Eventually though, the fluid slows enough (due to friction) such that it approaches the speed of the surface wave. At this point the wave is not carried away by the flow any more, it stays put and pushes back against the flow. This causes a build-up of fluid until finally the kinetic energy of the incoming fluid balances the potential energy of the fluid after the jump. At this point an equilibrium is reached. We can use Bernoulli's equation to analyze this equilibrium:

$$\frac{v^2}{2} + gz + \frac{p}{\rho} = constant$$

This equation is a form of energy balance. The three terms in the equation represent kinetic, gravitational potential, and pressure (potential) energy (respectively). Ignoring losses due to friction, their sum is constant across the hydraulic jump. The flow entering the jump has a lot of kinetic energy, but not much potential energy. The flow leaving the jump however, has less kinetic energy but a lot more potential energy because its higher than the upstream flow. The pressure of the fluid probably doesn't change much, so we can just ignore the pressure term.

Equating the sums of energies of the inlet and outlet flows gives:

$$\frac{v_{in}^2}{2} + gz_{in} = \frac{v_{out}^2}{2} + gz_{out}$$

There is also a nice derivation of the momentum equation for this case in the Wikipedia article on hydraulic jump.

Improve this answer
$\endgroup$
7
  • $\begingroup$ You seem to suggest that the jump occurs at a Froude value just above one. What causes the jump in the first place? Furthermore, substituting a value like this (e.g. 1.1) into the Belanger equation $\frac{h_2}{h_1} = \frac{\sqrt{1+8Fr^2}-1}{2}$ gives ratio very close to 1 meaning the jump is very slight. But hydraulic jumps are typically much larger than this. How does this work? $\endgroup$
    – MadCommy
    Commented Sep 29, 2015 at 15:46
  • $\begingroup$ An upstream Froude number of 1.1 would produce a very small jump indeed. I have modified the answer to address your question as to how the jump starts in the first place. $\endgroup$
    – Carlton
    Commented Sep 29, 2015 at 16:08
  • $\begingroup$ It's starting to make sense to me now. Would you mind elaborating a bit on how an equilibrium is reached? Kinetic energy balances the potential energy - does this mean the jump moves further upstream into the supercritical region to balance the two? $\endgroup$
    – MadCommy
    Commented Sep 29, 2015 at 16:34
  • $\begingroup$ Sorry to be such a pest; but does this mean that the hydraulic jump must move upstream away from the initial critical point (where the critical speed originally was) into the supercritical region to achieve the equilibrium? $\endgroup$
    – MadCommy
    Commented Sep 29, 2015 at 17:13
  • $\begingroup$ If the steady-state flow was disturbed in some way (change in flow rate, velocity, etc.) then the jump will move accordingly to establish a new equilibrium point. It could move up- or down-stream, it just depends on where the new equilibrium point is, i.e. where the Froude number crosses 1.0 $\endgroup$
    – Carlton
    Commented Sep 29, 2015 at 18:32
0
$\begingroup$

I am researching on this topic actually. Hydraulic jump is not an easy matter as it seems to be. the location of the jump also depends on the thickness after the jump. if you increase the height after the jump(for example, put a bump). the location of the jump will move upstream. it depends on a lot of parameters, such as the upstream speed, the downstream configuraion, surface tension. etc. with all being said, the true reason behind this phenomenon is still unknown.

Improve this answer
$\endgroup$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.