Lateral stiffness
Assuming the plates stay parallel, you can use lateral deflection of beam end while keeping the 0 rotation there. Replacing one of the plates with a force-moment couple, $F_1$ and $M_1$, the bending moment along the beam of length $L$ will be following:
$$M(x) = F_1\cdot (L-x)-M_1$$
Since how much the beam bends locally is proportional to the bending moment, i.e. (Bernoulli beam):
$$\frac{d\varphi}{dx} = \frac{M(x)}{EI_y}$$
where:
- $E$ is Young's modulus
- $I_y$ is second moment of area with respect to $y$ axis, for thin tube $I_y = \pi\cdot r^3\cdot t$
you can get the function of rotation as a function of $x$:
$$\varphi(x) = \varphi_0 + \int\limits_0^x \frac{M(x)}{EI_y} dx$$
The rotation at the start of the beam is zero $\varphi_0 = 0$ and after integration, you get the following expression:
$$\varphi(x) = \frac{1}{EI_y} \cdot \left(F_1\cdot \left(L\cdot x-\frac{x^2}{2}\right)-M_1\cdot x\right)$$
Now it is important, that rotation at the end of the beam is also 0:
$$\varphi(x=L) = \frac{1}{EI_y} \cdot \left(F_1\cdot \left(L\cdot L-\frac{L^2}{2}\right)-M_1\cdot L\right) = 0$$
From this, you get relationship between force and moment:
$$M_1 = F_1\frac{L}{2}$$
Lateral deflection is proportional to local rotation:
$$\frac{dw}{dx} = \varphi(x)$$
So the end deflection can be obtained by integration of rotation function with the relationship between end force and moment:
$$w_L = w_0 + \int\limits_0^L \varphi(x) dx = 0+ \frac{1}{EI_y} \cdot \int\limits_0^L\left(F_1\cdot \left(L\cdot x-\frac{x^2}{2}\right)-F_1\frac{L}{2}\cdot x\right) dx$$
So at the end, the deflection due to lateral force will be:
$$w_L = \frac{L^3}{12EI_y}\cdot F_1$$
And lateral stiffness of one tube is:
$$k_F = \frac{12E}{L^3}I_y$$
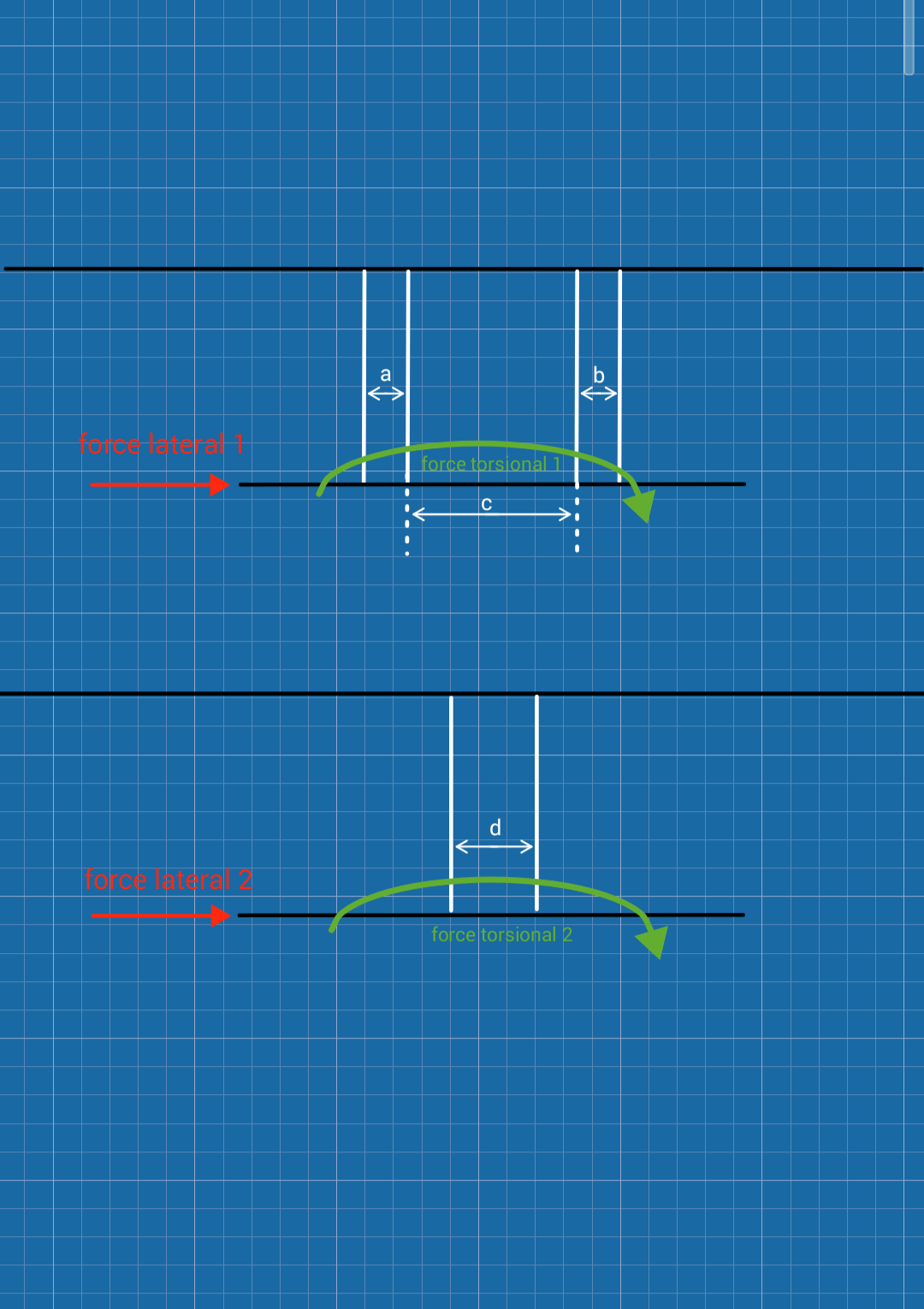
For your 2 cases and loading by force $F$ this means:
- one tube:
$$k_{F1} = \frac{12E}{L^3}\pi\cdot \frac{d^3}{8}\cdot t = \frac{3E}{2L^3}\pi\cdot d^3\cdot t$$
- two tubes:
$$k_{F2} = 2\frac{12E}{L^3}\pi\cdot \frac{a^3}{8}\cdot t = \frac{3E}{L^3}\pi\cdot a^3\cdot t$$
Torsional stiffness
Torsional stiffness $k_{T1}$ for single tube is trivial:
$$k_{T1} = \frac{GI_p}{L}$$
Since $G = \frac{E}{2(1+\nu)}$ and $I_p = 2I_y$ (just for tube), the stiffness will be:
$$k_{T1} = \frac{E\cdot \pi\cdot d^3\cdot t}{16(1+\nu)L}$$
In case of two tubes, you need to take into account their torsional stiffness, but also lateral stiffness, since twisting the couple by angle $\vartheta$ will twist individual section by the same angle, but also laterally displace ends of the beams by $\vartheta\cdot (c+a)/2$, which would be caused by force from moment $M = F\cdot (c+a)$. Using only this lateral deflection, we can write:
$$\frac{k_{F2}}{2} \cdot \frac{c+a}{2}\cdot \vartheta = \frac{M}{c+a}$$
$$\frac{(c+a)^2}{4} k_{F2} \cdot \vartheta = M$$
So the total torsional stiffness will be:
$$k_{T2} = \frac{E\cdot \pi\cdot a^3\cdot t}{8(1+\nu)L} + \frac{(c+a)^2}{4} k_{F2}$$
Stiffness to weight ratio
I am not sure if stiffness to weight ratio is meaningful for this case, but since the both cases have the same weight, relative stiffness difference will be the same. Using relation between $a$ and $d$; $d = 2a$ (from thin shell assumption), you can calculate how much is the second case stiffer compared to the first one:
- lateral sitffness:
$$\frac{k_{F2}}{k_{F1}} = \frac{1}{4}$$
- torsional stiffness:
$$\frac{k_{T2}}{k_{T1}} = \frac{1}{4}+\frac{3(c+a)^2}{2}\cdot\frac{1+\nu}{L^2}$$
In conclusion, lateral stiffness of single tube is $4\times$ greater than for two tubes. Torsional stiffness is more complicated, but if we focus only on tube twisting, the case of one tube would also be $4\times$ greater. However, the two tube case has additional stiffness resulting from lateral stiffness, which you can calculate for your particular set of parameters.