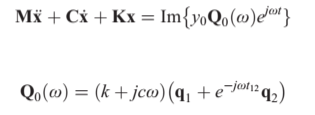Euler's formula with different coefficients and constants

Question

Hi and thank you in advance. I understand that one of Euler's formulas states:

cos(x) + isin(x) = e^ix 

I know that if you were to have the same coefficient attached to sin and cos the following would hold true:

 4*cos(x) + 4*isin(x) = 4*e^ix or z*cos(x) + z*isin(x) = z*e^ix

Additionally a constant could be applied inside the trig term and this would hold true:

cos(3x) + isin(3x) = e^3ix or cos(xt) + isin(xt) = e^ixt

I have trouble understanding how we can utilize this though say if any of the above coefficients were to not be uniform. Say:

4*cos(x) + 3*isin(x) = ?

If you were to say the above is equivalent to 7e^ix, this not true. How can you use eulers formula with different coefficients? Is it possible?

I ask this because a fellow student used the following:

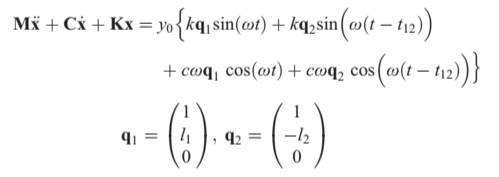
and somehow through Euler's converted it to the following:

Answer 1

$$sin(\omega t) = Im\{e^{j\omega t}\} $$

But also

$$cos(\omega t) = Im\{je^{j\omega t}\} $$

Since

$$ j\left(cos(\omega t) + jsin(\omega t)\right) = jcos(\omega t) - sin(\omega t) $$

So then you can do:

$$ k \pmb{q_1} sin(\omega t) + c\omega \pmb{q_1} cos(\omega t) $$

$$ k \pmb{q_1}Im\{e^{(j\omega t)}\} + c\omega \pmb{q_1} Im\{je^{(j \omega t)}\} $$

$$ Im\{(k \pmb{q_1} + jc\omega \pmb{q_1} )e^{(j\omega t)}\} $$

Answer 2

For your example: 4cos(x) + 3isin(x) = ? Plot a point at (4,3), find the distance from origin to point = $(x^2 + y^2)^½$

Then $4*cos(x) + 3*isin(x) = 5e^{i\theta}$

$\theta$ is the angle from the x axis.