TL;DR: ball bearings deform due to Hertzian pressure and therefore distribute the load
However, the reason balls (rolling elements) don't easily crack is the ductility of the material (and to some lesser extent the treatment of the material)
The Deformation of the bearing balls due to Hertzian pressure contributes to the distribution of the loads and only delays failure. However the mode of failure is not affected. So for a high enough pressure you could have a load that will lead to failure, but you wouldn't expect it to crack.
Distribution of loads due to Hertzian pressure deformation
Every material in deforms when a load is applied. The ball bearing although they are very stiff and seem non-deformable they experience small deformation, due to what its called Hertzian pressure. The deformation is what causes distribution of the loads to neighboring balls.
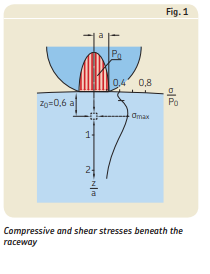
The contact surface between a ball bearing and a contact surface is:
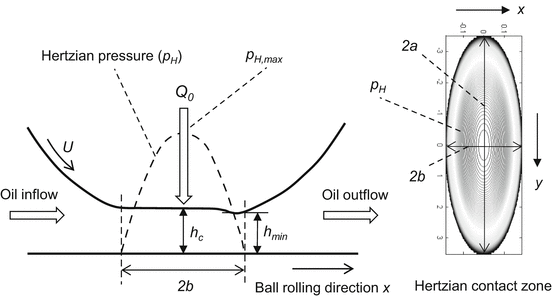
Figure: contact between a ball bearing and a raceway with a lubricant (source Springer)
The end result (exaggerated) is the following:
| 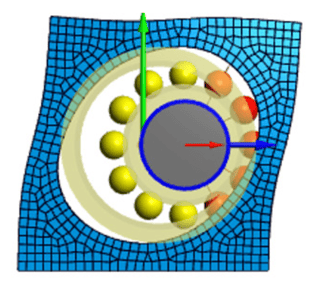 |
|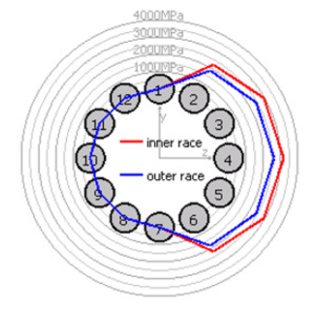 |
|
Figure: Exaggerated deformation of elastic bearing (left) and corresponding stresses (right) - (Source bearing news)
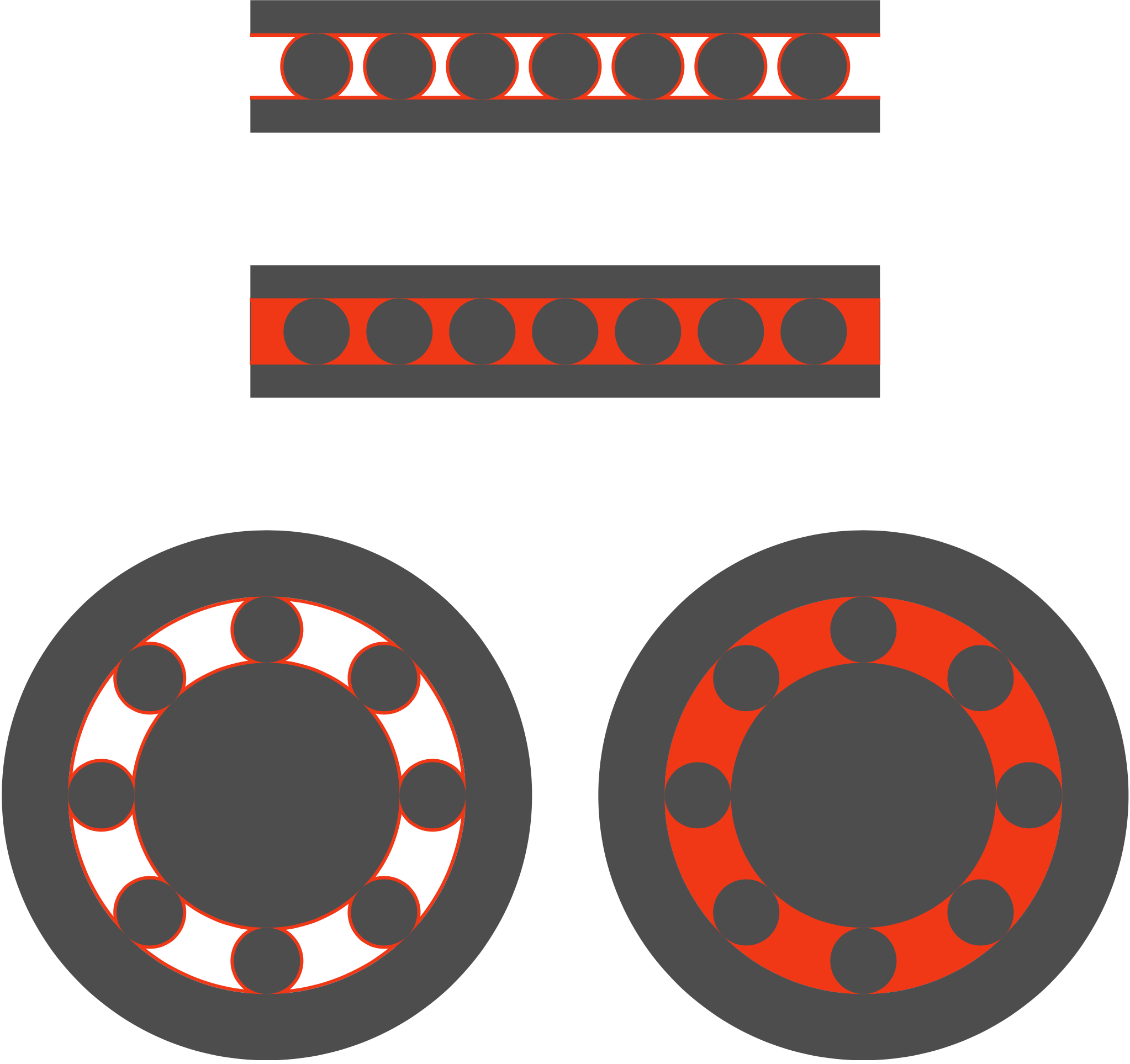
the red ball bearings are the one in contact. Obviously the central one carries the highest load compared to any other single ball. However, the load is distributed at different bearing (see image below)
Also, the load on the central bearing significantly reduces compared to others as the load increases (because deformation is not linear).
So there is a significant distribution of the loads (however that does not explain by itself why the balls don't crack because for a high enough load there could be a case where the loads are such that the bearing will fail)
Compressive failure of Ductile vs Brittle material.
When a material is compressed, depending on its ductility it will behave differently.
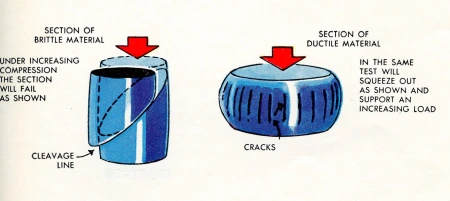
Figure: Compressive brittle and ductile failure (source:pmpaspeakingofprecision)
In the brittle failure there is a plane at 45 degrees (where the maximum shear strength is observed that a crack develops).
In the failure of ductile material under compression, there will be a lateral increase of the ball (like squashing a tennis ball), and eventually when the maximum shear stress of the material is reached cracks will appear radially (see above).
Steel is a ductile material and it is expected to fail in a ductile manner. However, bearing very rarely fail by cracking of the load. By the time the reach a more brittle mode failure, the shape is such that the bearing will not be able to rotate anymore and it will become stuck. This in turns causes additional stresses from torsional moments etc which are unpredictable.
Additionally ball bearings treated (heat and also mechanically sometimes) to create prestresses that create a compressive stress on the surface which tends to arrest cracks. Because there are those compressive stresses, they tend to arrest any cracks (which usually develop from the surface).
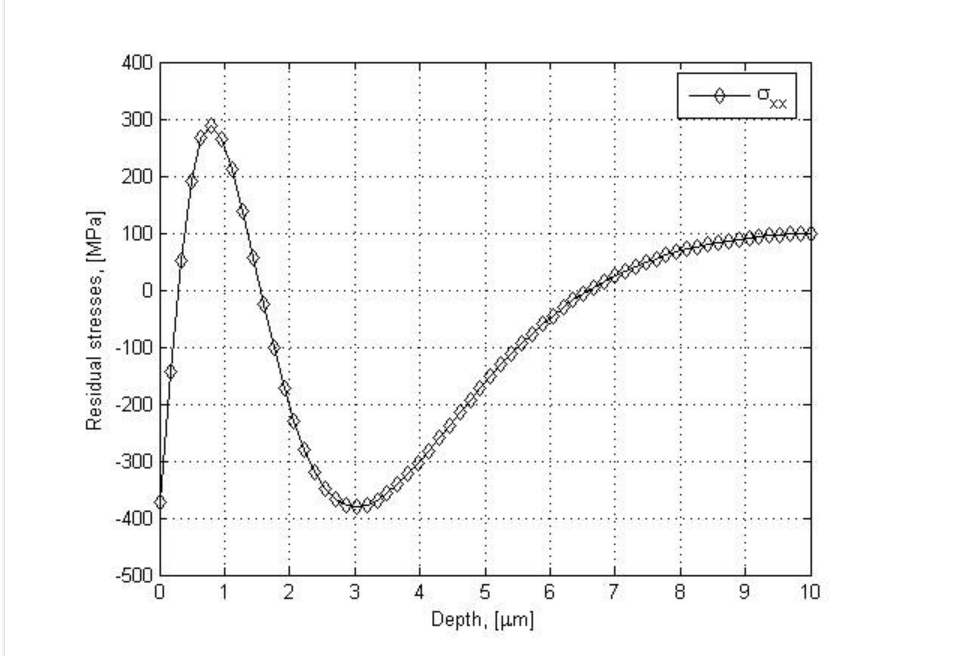
Figure : (source [Marcelin Benchea](Residual stress versus depth along the contact axis ))