9
$\begingroup$

As a layman, how can I know the shear strength of a particular size bolt?

For example, if I want to attach something to the internal wall of my van ( 1mm stainless steel ) using a rivnut and standard metric bolt and it should hold up to 50 kg, how would I figure out the right size and grade to use? Nothing specialty, just standard stuff a layman could get at a typical hardware store.

In my specific case I'm installing wooden cabinets on the internal walls of my Fiat Ducato van, but I'd like as general an answer as reasonable.

Improve this question
$\endgroup$
5
  • 10
    $\begingroup$ The shear strength of the bolt is likely not going to be the limiting factor. Pullout force of the rivnut or flex in the sheet metal are more relevant. Just use plenty of whatever size is most convenient to spread the load. $\endgroup$
    – Jonathan R Swift
    Commented Sep 12, 2021 at 18:07
  • 1
    $\begingroup$ Honestly, having fitted out a van myself, the limiting factor is likely to be vibration and loosening. Something, somewhere, will work loose, perhaps in the construction of the cabinets themselves. Differential thermal expansion between wood and steel isn't your friend either $\endgroup$
    – Chris H
    Commented Sep 13, 2021 at 13:36
  • 6
    $\begingroup$ Anybody can design a bridge that stands. But it takes an engineer to design a bridge that just barely stands. Don't be an engineer. Overdo it. $\endgroup$
    – dotancohen
    Commented Sep 13, 2021 at 15:46
  • 1
    $\begingroup$ @dotancohen You'd have to be an aerospace engineer for that to hold. Other engineers use safety factors that are noticeably higher than 1. $\endgroup$
    – TooTea
    Commented Sep 14, 2021 at 9:10
  • 1
    $\begingroup$ @TooTea sure, but the point is they don't use a safety factor of 50, because that would be prohibitively expensive and of marginal real safety benefit, but spending \$50 instead of \$5 on your van is less so $\endgroup$
    – llama
    Commented Sep 14, 2021 at 15:48

7 Answers 7

Reset to default
13
$\begingroup$

For a layman, you shouldn't use bolts stressed in shear. Bolts seldom hold shear forces. Bolts are used to hold two surfaces together so that the joined components take the shear stress. It is the friction of the two surfaces that support the load. This is the principle that holds wheels on automobile hubs. The friction of hub to the wheel takes the forces, not the shear strength of the studs.

For holding a cabinet up, just use appropriately sized bolts and you will be fine.

ETA: As @r13 comments, the engineering work of using friction of the joined pieces is to determine the required friction, the required bolt tension, number of bolts, the required bolt torque to get that tension, bolt specifications, etc. I consider this beyond the layman level.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ The friction type bolting is preferable over the bearing type as it provides additional safety factors against mishaps. However, the proper torque and resulting strength level usually remain a mystery for most non-technical people. $\endgroup$
    – r13
    Commented Sep 13, 2021 at 1:30
  • 2
    $\begingroup$ If your bolts are rated for the shear load, you don't have to worry about slip-critical joints and their complexity. Maybe you've got enough tension to keep the joint from slipping, maybe you don't. It's a lot easier to add up a dozen shear ratings off the spec sheet (then give yourself a safety margin since different bolts might take different loads) than to worry about friction levels. Since the correct answer shouldn't be "go slip-critical", you should probably explain what an appropriately-sized bolt is. $\endgroup$
    – MichaelS
    Commented Sep 13, 2021 at 21:09
15
$\begingroup$

For complete layman's terms, an m6 bolt can hold up an elephant. For 10 kg, the bolts won't be the weak link, it will be whatever you are bolting into. Use 3 or 4 and you will probably be fine

The fine print: I'm considering an average female Asian elephant (6000 lb or 2700 kg), grade 8 bolts (the expensive "top shelf" bolts) , double shear, and no additional safety factor.

Improve this answer
$\endgroup$
1
8
$\begingroup$

The first thing to know is the "grade" of the bolt is available to you. Then use the appropriate table (similar to the one below) from the standard code to determine the loading capacity of the bolt. Note that your application shouldn't involve "bending" and "twisting", for which the table is not appropriate for use. Also, you shall provide a safety factor to the listed loads, Applicable Shear (Tension) = List Value/Safety Factor.

enter image description here

enter image description here

enter image description here

Single Shear vs Double Shear

In general, double shear has twice the shear area compared to single shear.

enter image description here

Note: When you need the information on the bolt with a smaller size, either try to get the information from the appropriate code, or, most directly, read the instructions of the product, which usually shall indicate the safe working load.

ADD: Capacity of Screws (Imperial)

enter image description here

Conversion: 1 kgf = 2.2 lbs

Gauge #, mm:

#6 - 3.5mm, #8 - 4mm, #10 - 5mm, #12 - 5.5mm

Improve this answer
$\endgroup$
7
  • 1
    $\begingroup$ Good answer, although perhaps a table showing M4 thru M12 would be more appropriate for the task at hand! $\endgroup$
    – Jonathan R Swift
    Commented Sep 12, 2021 at 19:56
  • $\begingroup$ @JonathanRSwift This answer is meant to provide an example of how and where to get one's hand on the loading capacity of a fastener of a specific grade. Unfortunately, the data source only made the information of the structural grade bolts available. For smaller sizes (machine bolts), the OP needs to find the information through other venues. $\endgroup$
    – r13
    Commented Sep 12, 2021 at 21:20
  • $\begingroup$ You can’t find data on M4 to M8? $\endgroup$
    – Solar Mike
    Commented Sep 13, 2021 at 5:02
  • $\begingroup$ @SolarMike r13 presumably comes from either the USA or Liberia, judging by the choice of units in his/her posts. (But from his/her frequent unidiomatic use of "shall" I might have guessed Germany instead...) $\endgroup$
    – alephzero
    Commented Sep 14, 2021 at 19:50
  • $\begingroup$ @SolarMike No. Not for the structural bolt which the table covers. I guess a bolt smaller than M12 may not be permitted for structural use in the British, you can conveniently check it out. $\endgroup$
    – r13
    Commented Sep 14, 2021 at 20:56
4
$\begingroup$

I will start by highlighting the main points (some were also found in other answers). I will additionally provide a short calculation for the tensile strength (and shear strength) very quickly by knowing the type (e.g. M6) and grade (e.g 10.9). and below I will providing a layman's calculation for a metric bold.

In the numerical calculation I will use a very small, and very low grade bolt to show case that the bolts usually aren't the weak link in this case.

the important parts for a layman

  • (tiger guy's): Bolts shouldn't be used in shear (they can be used but in most scenarios are not suggested)
  • for 50 kg any metric bolt should be able to hold.
  • (Daniel K) In many cases, bolts are not the weak link in the assembly.

Calculation for bolt tensile strength

The type M6 is indicative of the diameter of the core. So the cross-sectional diameter (d)of the core is (close to) $A = \frac{\pi \cdot d^2}{4}=\frac{\pi\cdot 6^2}{4}$

The grade shows the material type. It consist of two numbers separated by a dot (X.Y). E.g. 8.8.

  • The first number multiplied by 100 is the UTS of the material MPa
  • The second number (with the dot) is the ratio of the yield stress to the UTS of the material.

So for an 5.6 bolt the UTS is $5\cdot 100 =500 MPa$, and the yield stress is $0.6\cdot \sigma_{UTS}=300 MPa$. The followonig table has some of the most common bolt grades.

UTS yield stress
4.6 400 MPa 240 MPa]
5.6 500 MPa 300 MPa
8.8 800 Mpa 640 MPa
10.9 1000 MPa 800 MPa
etc .. ..

So the tensile strength up to yield strength of an M4 4.6 is: $$ A \cdot \sigma_y = \frac{\pi\cdot (4 [mm])^2}{4} \cdot 240 \left[\frac{N}{mm^2}\right]= 3015 N$$

Or approximately 300 kg.

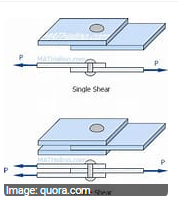
Calculation for bolt shear strength

For the shear strength, you can approximate the shear yield stress to 0.5 of the tensile yield stress (this is a very conservative approximation if you compare it to tables from Eurocode). So the shear load should be in the order of (at least) 150 kg.

Please note that I used in the example one of the smaller metric bolts M4 (its hard to find a wrench them), at the lowest grade, and it still was capable of holding (At least) 3 times the 50 kg load.

Improve this answer
$\endgroup$
1
  • $\begingroup$ The type indicates the outer diameter, i.e., including the thread, so the core is a bit less, around 3.1mm for a standard coarse pitch M4. Conservatively basing a strength calculation on the core only, which seems to be normal practice, would reduce the tensile strength in your example from around 310 kgf to around 180 kgf. $\endgroup$
    – Alex Selby
    Commented Jun 17, 2023 at 16:31
3
$\begingroup$

Source, Eurocodeapplide.com

Shear strength of bolts

The shear resistance of the bolt per shear plane Fv,Rd is provided in EN1993-1-8 Table 3.4:

Fv,Rd = αv ⋅ fub ⋅ A / γM2

where:

αv is a coefficient that takes values αv = 0.6 for bolt classes 4.6, 5.6, 8.8 or αv = 0.5 for bolt classes 4.8, 5.8, 6.8 and 10.9. When the shear plane passes through the unthreaded part of the bolt αv = 0.6.

  • fub is the ultimate tensile strength of the bolt depending on the bolt class (see table above)

  • A is the appropriate area for shear resistance. When the shear plane passes through the threaded part of the bolt A is equal to the tensile stress area of the bolt As. When the shear plane passes through the unthreaded part of the bolt A is equal to the gross cross-sectional area of the bolt Ag.

  • γM2 is the partial safety factor for the resistance of bolts in accordance with EN1993-1-8 §2.2(2) Table 2.1 and the National Annex. The recommended value in EN1993-1-8 is γM2 = 1.25.

For small DIY work i would recommend using 0.3 Ag multiplied by fu of the bolt you use. but you have to mind if bolt is too strong it can tear or warp the material.

Improve this answer
$\endgroup$
1
$\begingroup$

I would go with M6 or M8 and use several.

A variety of values are possible for each size as there are grades of bolts.

The material that the rivnut is fitted into may be the limiting factor - body panels are not that strong which is why they have ridges in them especially the supporting ribs.

I have often just pried rivnuts out when the bolts have been seized.

Improve this answer
$\endgroup$
0
$\begingroup$

I would use a boat washer behind the steel panels to spread the loads, as well as an l angle underneath to support the weight and lock nuts too. Just some common sense .

Improve this answer
$\endgroup$
1
  • $\begingroup$ As it’s currently written, your answer is unclear. Please edit to add additional details that will help others understand how this addresses the question asked. You can find more information on how to write good answers in the help center. $\endgroup$
    – Community Bot
    Commented May 18 at 20:19

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.