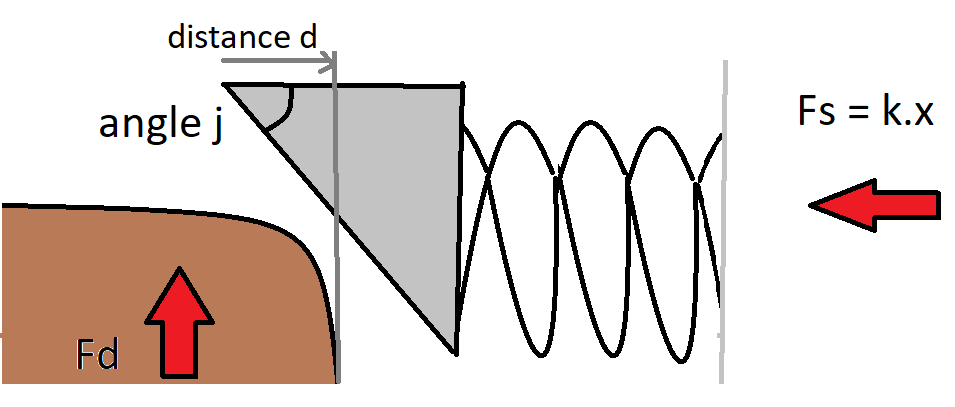
I want to make a sprung latch mechanism which keeps a door in place until a certain force is applied to the door. In the diagram attached, the force the door must release at is Fd, and the latch spring force necessary to hold it until Fd is reached is Fs.
My questions are these: 1. How do I work out what force spring I need? 2. Assuming the contact is frictionless, and that distance d stays the same, does it make a difference what the angle j is?
Many many thanks for any help!
The force of resistance of the latch varies. at the time the door touches the latch it is zero and when it is pushed fully back it is k*d.
Edit
why F*tan j is the horizontal component? after OP's comment.
If we draw the perpendicular, $F_d*cos j,$ and $F_d* Sin j \ , $ components of Fd on the surface of latch, the hypotenuse of the triangle is the tan of that angle and is the horizontal component factor of the Fd.
We have ignored the round corner of the door on the diagram and assumed it's a sharp point.
End of edit. $$ F_d*tanj=k*d \\F_d=\frac{k*d}{tanj }$$
The steeper the angle J the less force is needed to push the door open.
