1
$\begingroup$

I need to find the response of my FTIR spectrometer. I do have a calibrated black-body source (area-emitter) which I measured at various temperatures.

The system operates in inverse cm, cm-1. My approach so far was the following:

  • 1) Measure spectra at temperatures $T_i$

  • 2) Scale the spectra i.e.

    • the x-axis $x_{in~um} = 1e4/x_{in~cm-1}$
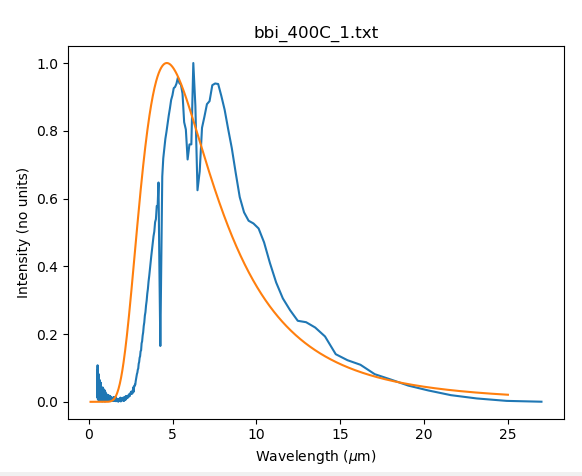
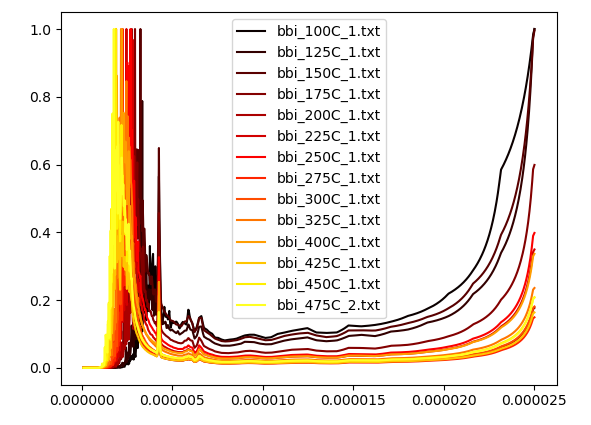
    • the y-axis, $y_{in~um} = y_{in~cm-1}/x_{in~cm}^2$ to account for the dispersion relation between measured energy spacing and desired wavelength spectrum. At this point an example for scaled measurement (blue) and theoretical blackbody (orange) at the same nominal temperature looks like this (CO2 and H2O lines are at the correct positions): enter image description here

  • 3) calculate the response function $R=I_{BB}(T_i)/y(T_i)$ (I think, here in a first approach I assume that the background radiation does not contribute significantly. I would have expected similar response functions for the different temperatures; however what I get looks like:

enter image description here

The "response function" drifts with temperature. I am unsure now if I have a misconception in the defintion of the response function, or if I picked up an artefact. I hope someone with more experience can help me out?

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ How have you calibrated the transmission function of the spectrometer system itself? Is it constant with temperature? A reference to a comparable approach is in this publication $\endgroup$
    – Jeffrey J Weimer
    Commented Nov 23, 2019 at 21:29

1 Answer 1

Reset to default
1
$\begingroup$

For anyone coming later:

I ended up using a procedure described here:

Kevin C. Gross, Phenomenological model for IR emission from high explosive detonation fireballs

In one sentence: I ended up measuring the blackbody spectrum at increasing temperatures. Then fitting a linear relation between measured and theoretical value for emission intensity (per wavelength) to obtain system gain and offset. So far, this leads to consistent results when measuring validation samples. Thanks @ Jeffrey J Weimer for the tip.

Improve this answer
$\endgroup$
1
  • $\begingroup$ Glad to hear that you found a method to do what you needed. $\endgroup$
    – Jeffrey J Weimer
    Commented Feb 11, 2020 at 14:25

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.