LED Lumen Calculations

Question

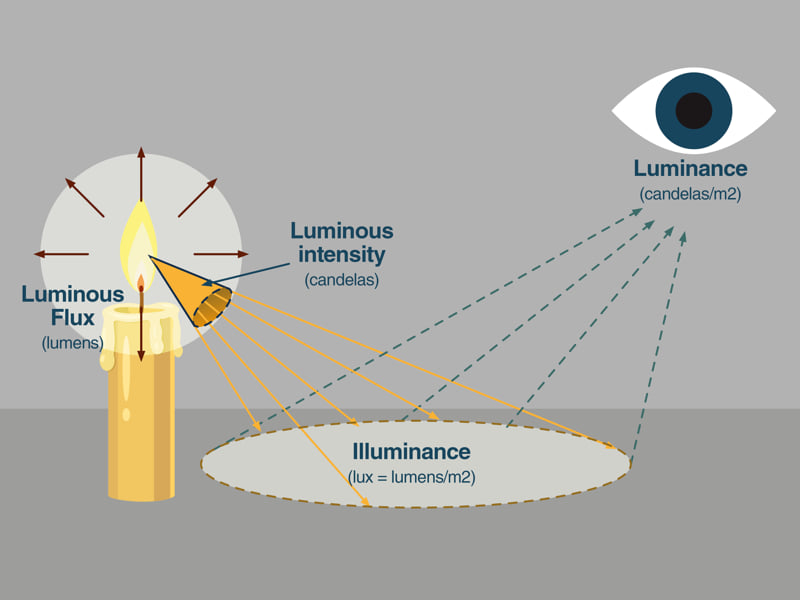
I am using an LED as a point source for a school project. I have two questions in regards to the LED(s).

I know that light follows the inverse square law when measuring perceived brightness, but all sources I can find show examples of a round bulb light. Is there an altered version of the formula for LED’s that incorporates factors such as the fan angles?

Also, I will most likely be adding more LED’s to my project to increase the total lumens/lux. I’ve seen many people ask the question whether lumens will add in such a scenario. In theory they would but it wouldn’t be perceived that way. My question is; is there a formula/equation/calculation that can help me predict the lumens perceived by x number of LED’s, d inches apart from one another, measured at y feet away, with regard to joining and isolated parts of the fan angles of each LED.

Answer 1

Lumen (luminous flux) is irrelevant to the distance where the LED's being measured from, you can take lumen as a total light energy measured around that LED (captured by an all-rounded measuring sphere).

While Lux (illuminance) on the other hand is related to how far you're measuring the light from the LED.

The formula simply is as below: Lux = Lumen / Area of interest

Page below might be useful for you: https://www.compuphase.com/electronics/candela_lumen.htm

Answer 2

If you want to look at an area that light is illuminating then you are probably best working with Illuminance which is measured in lux. The inverse square law looks how the area reflecting the light increases as you get further away, but if you can calculate the area reflecting the light from a known angle, distance and luminous flux (lumens).

With regards to your second question it would appear there is a roughly square relationship between the amount of light and what the eye perceives so...

Perceived light ≈ √Actual light

Answer 3

This problem is more complicated than you first think.

Say a bulb at $x$ watts increases to $2x$ watts instead (same size). In theory the perceived light will be $\sqrt{2}x$.

If we now go to LEDs, placing two beside each other, we have two different light sources, with different incident angles to the target, on close proximity we can probably assume the angle, if negligible, the real distance for each of the two LEDs would be $\sqrt{( \frac{x}{2})^2 + d^2 }$, where $x$ is the distance between the diodes and $d$ is the distance to the retina (approx 22 mm inside the eye).

If the angle is less than 0.025 degrees ($2\ arctan(\frac{x}{2}d$) (I may remember incorrectly, or there will be more accurate medical data available) the light will hit a single cone/rod in the eye. If the angle if larger it will hit several, due to lateral inhibition in the eye the light hitting at an angle less than 0.025 deg will be perceived to be more intense than actual light, about 80% of each source or 160% vs 141% if the angle was greater.