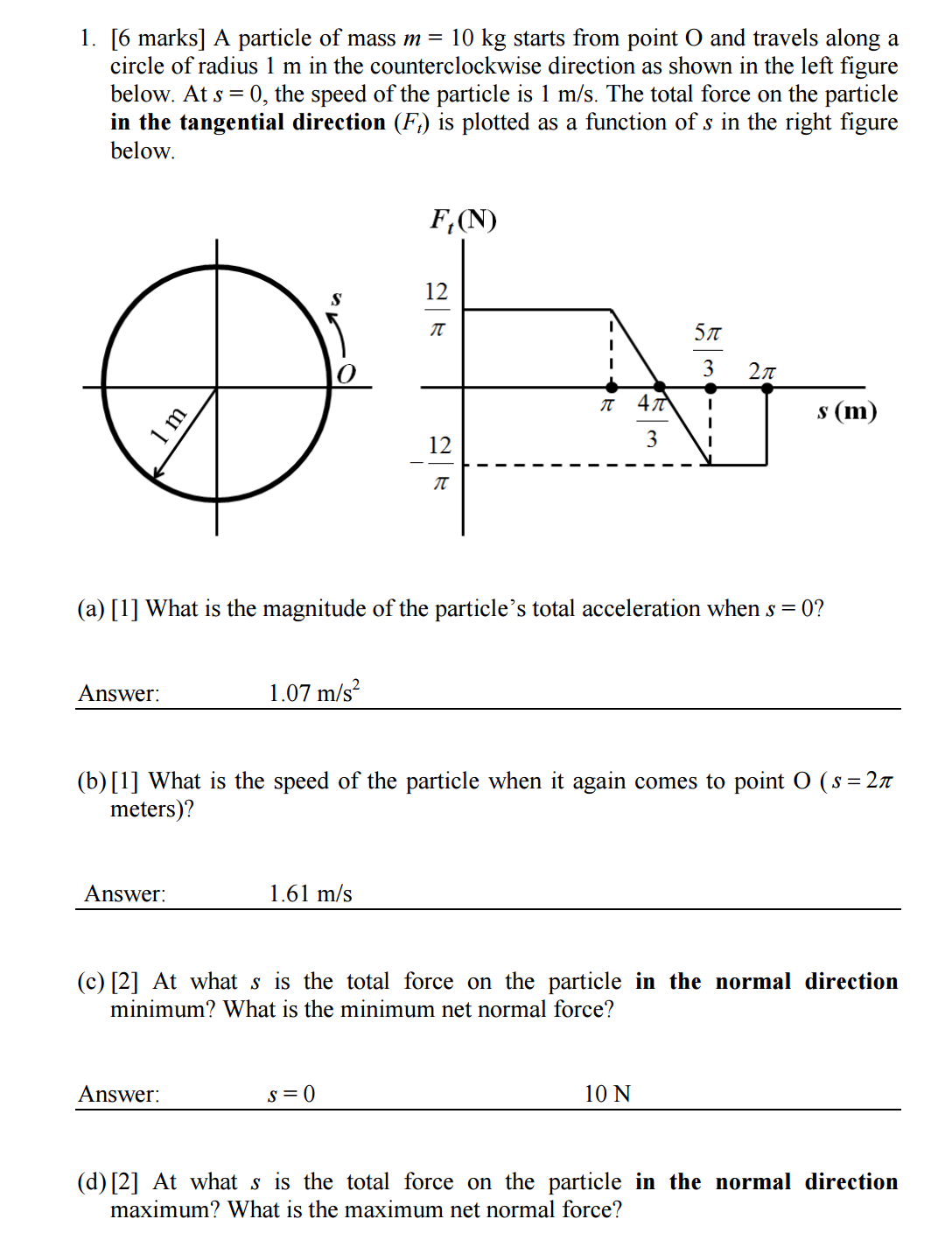
b) I dont understand how to take the integration like its integral of ft from 0 to 2 pi = integral of velocity, and the integral of the first is the area under the graph= 8j and then I dont know how to get the answer fron there cause dont get what to plug in ? Any help is greatly appresiated.
For the first question, you can split up the equations of motion into normal and tangential direction:
$$ma_n=F_n$$ $$ma_t=F_t.$$
The tangential force $F_t=12/\pi \text{ N}$. Hence, $a_t=\frac{12}{10\pi} \frac{\text{m}}{\text{s}^2}$. For the normal force (centripetal force) you have to remember that $F_n=m\frac{v^2}{r}$. Hence, $a_n=\frac{v^2}{r}=1\frac{\text{m}}{\text{s}^2}$. The total acceleration is given by $a=\sqrt{a_t^2+a_n^2}=1.07\frac{\text{m}}{\text{s}^2}$.
So, now it's up to you. Try the other questions and tell us what you have tried or where you got stuck.
Edit: For b) we note that $$ma_t=F_t\implies \frac{F_t}{m}=a_t=\dfrac{dv}{dt}.$$ This can be rewritten by the chain rule (Note, that $ds/dt=v$) as: $$\frac{F_t}{m}=\dfrac{dv}{ds}\dfrac{ds}{dt}=v\dfrac{dv}{ds}\implies \int_{s=0}^{2\pi}F_t ds=m\int_{v_0}^{v_{2\pi}}vdv.$$
The integral of on the right-hand side is just the area in your $s-F_t$-diagram. It turns out to be $8 \text{ J}$. Can you continue from here?
