At what volume would air bubbles become static in a fluid with viscosity of 7000cps

Question

I would appreciate any help with this as its been a few years since my fluid dynamics courses at University!

Basically I am trying to determine the cause of voids that we are seeing in a set resin. There are a multitude of other influences at play including venting and the geometry of the cavity that is being filled, but this made me curious about the movement of air through this resin during application and if under normal atmospheric application (not under vacuum) if this would always be inherent to the process.

As stated, the cps value of the resin is 7000, would someone be able to point me in the direction of a formula which I can use in excel to specify the volume of the air bubbles to predict their velocity in this fluid?

Thanks

Answer 1

TL; DR: this is actually complicated and I don't know if your question can really be answered.

The movement of (practically) spheres in a fluid, you can use Stoke's law for very low Reynolds numbers, as we would expect in a thick resin (without doing the actual math, that part is up to you). Just find the equilibrium between bouyuancy and stokes' friction for the rising speed of the bubble. This tells you that in a newtonian fluid with a viscostiy of 7000 cps air bubbles will be very slow indeed, the smaller the slower, but they will always rise.

However, you wanted to know at what volume bubbles become static in resin - so you need to know if resin is indeed a newtonian fluid. This has been investigated, find this paper. I think the interesting information is this figure:

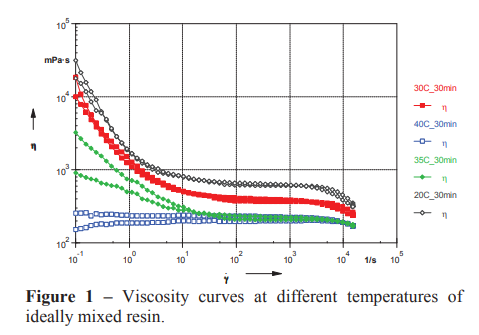
The graph shows viscosity vs. shear rate and shows that between $\gamma = 100s^{-1}$ to $1000 s^{-1}$ viscosity is constant (the fluid is newtonian), outside this range the fluid is shear thinning.

The problem is that viscosity depends on shear rate, not stress - so we can't make a straightforward prediction of the actual viscosity based on the buoyant force.

There is some discussion on wether yield-stress fluids or bingham plastics exist, or if these are in fact only shear thinning fluids with very high viscosities. In either case, the invvestigation of epoxy resin I linked doesn indicate yield stress behaviour for resins - if this were the case, we could calculate the yield stress below which the resin is plastic, and from there the bubble size. I've had a similar idea a few years ago.