Thermodynamics question

Question

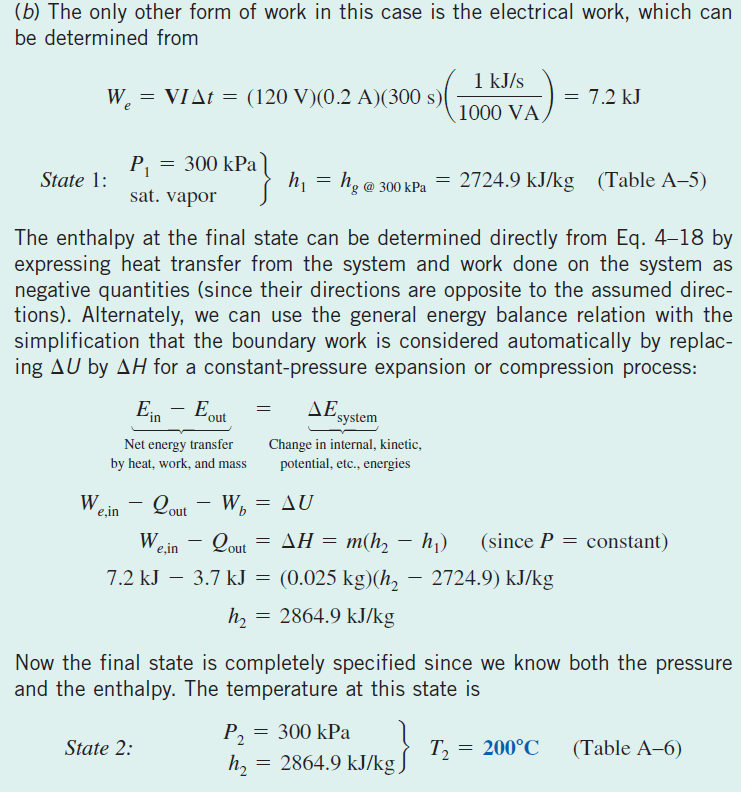
I am looking for help with the following question please.

A piston cylinder device contains 28g of saturated water vapor that is maintained at a constant pressure of 300 kPa. A resistance heater within the cylinder heats the saturated water by added 8 kJ of heat. At the same time, a heat loss of 3.5 kJ occurs.

(i) Show that for a closed system the boundary work Wb and change in internal energy $\Delta U$ in the first law relation can be combined into one term, $\Delta H$, for a constant

(ii) Determine the final temperature of the steam.

So far i have this for (i):

For a constant pressure expansion or compression process:

$$\delta u + Wb = \delta H$$

which is a quasi equilibrium process

I am quite stuck on this. I would like some pointers to start through this please. I'm looking to understand the problem better.

(Edit)

For (ii) i have found how to complete the question. Now could i get some help to complete the question please? How do i calculate State 1 to get 2724.9 kJ/Kg??

Once i keno this then i can place my information in and calculate the final temperature of the steam.

Answer 1

You have the cylinder held at constant pressure $p = \text{const}$, some heat is added $8 \ \text{kJ}$ and some heat is lost $3.6 \ \text{kJ}$, making the net heat added to the system $Q_{in} - Q_{out} = 8-3.5 = 4.5 \ \text{kJ}$

We are keeping the cylinder held at constant pressure, meaning that we're allowing the piston to move freely as a reaction to the current state of the system. So, we add heat, the saturated water vapor's internal energy will increase, this will result in an increase of the volume, water vapor exerts work against the piston, so the piston moves up.

We can show that (assuming no change in kinetic or potential energy): $$\Delta E_{sys} = W_{in} + Q_{in} - W_{out} - Q_{out} = \Delta U$$

(i) Show that for a closed system the boundary work $W_b$ and change in internal energy $\Delta u$ in the first law relation can be combined into one term, $\Delta H$, for a constant.

For a closed system at constant pressure, this can be achieved by adding the boundary work term $W_{out}$ to the right hand side, since The enthalpy $H$ of a thermodynamic system is defined as the sum of its internal energy $U$ and the work required to achieve its pressure and volume, since pressure is constant we can write: $$Q_{in} - Q_{out} = \Delta U+W_{out}= \Delta H=4.5 \ \text{kJ} = \text{const}$$

Determine the final temperature of the steam. You know that water vapor state 1 is at $p = 300 \ \text{kPa}$ and it's saturated, so quality factor $x = 1$, so you can easily get internal energy of such state using your steam table.

For state 2, you apply the first law formulation above, calculate the internal energy of the second state, so now for state 2 you know 2 properties about it, pressure and internal energy, again you can open your steam table and interpolate for superheated region at $300 \ \text{kPa}$ and the internal energy value you have to obtain the temperature.