1
$\begingroup$

I want to create a Pneumatic cylinder, The purpose is, Inside it will will contain a Nylon piston, and one end will be filled with clay. Cylinder will be closed from either side using end caps, which also has Push-in connectors(1/4" BSP). Compressed Air from a cylinder(Max. pressure of Cylinder 10 Bar) will be given to Piston side through a Non return valve and a Regulator. Which will push the Clay out through other end.

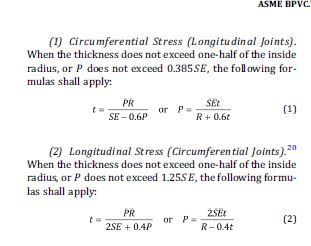
  1. What should be the Wall thickness of Cylinder? It should safely handle Maximum pressure that might arise.

  2. I have already provided a Safety valve calibrated at 6 Bar in my prototype that I made from a GI water Pipe, which has thickness of 3.85 mm. Is there any way the pressure inside the tank could reach a pressure higher than the maximum pressure Compressor can deliver (Compressor is rated for 10 Bar, with cutoff at 8 Bar)

  3. I'm thinking about making the cylinder using a 100 x 5.75 mm Aluminium Pipe and End caps from Aluminum Rods. Would this be enough?

  4. Should I give more thickness for End caps than cylinder?

  5. How to find the maximum pressure rating of the cylinder if material, Wall thickness and diameter are known?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
0
$\begingroup$

I recommend you to use one of the codes/standards in order to be on the safe side. For example you can calculate cylinder wall thickness according to ASME, the following formulas are taken from the pressure vessels, but they are good enough to give you a point: for flat cover and cylinder respectively For flat covers

For cylinder

"E" is a joint efficiency , if you use seamless part consider it 1, if not than 0.7 for circumferential stress. For longitudinal stress in your case ,I think, you should use 0.7

"S" is a permissible material stress= UTS/3.5

"P" is a pressure

"t" is a wall thickness

"C" is a factor of attachment of a flat cover, usually between 0.1 to 0.3

"d" inner diameter

"D" and "R" are outer diameter and radius

be consistent with proper units(SI,imperial etc.)!

Improve this answer
$\endgroup$
3
  • $\begingroup$ Can you give me units of each parameter? And what is meant by thickness does not exceed one-half of inside radius? $\endgroup$
    – Athul
    Commented Nov 26, 2018 at 5:13
  • $\begingroup$ The reason that the $t<r_{in}$ is that otherwise the assumptions that lead to the formula no longer apply. Basically, the formula applies for a thin-walled cylinder. $\endgroup$
    – NMech
    Commented Aug 15, 2020 at 13:31
  • $\begingroup$ The stresses in the cylinder wall are proportional to both the pressure and the radius. A pressure vessel 8ft in diameter might need ¾” thick steel walls, while a tube connected, say, to a pressure gauge might only need 1/16” brass for the same pressure. This is also the reason that the thickness of flat end caps has to be calculated in a different manner - they have infinite radius of curvature, (and is why most end caps will be slightly curved.) $\endgroup$
    – Rich
    Commented Mar 27 at 21:58

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.