2
$\begingroup$

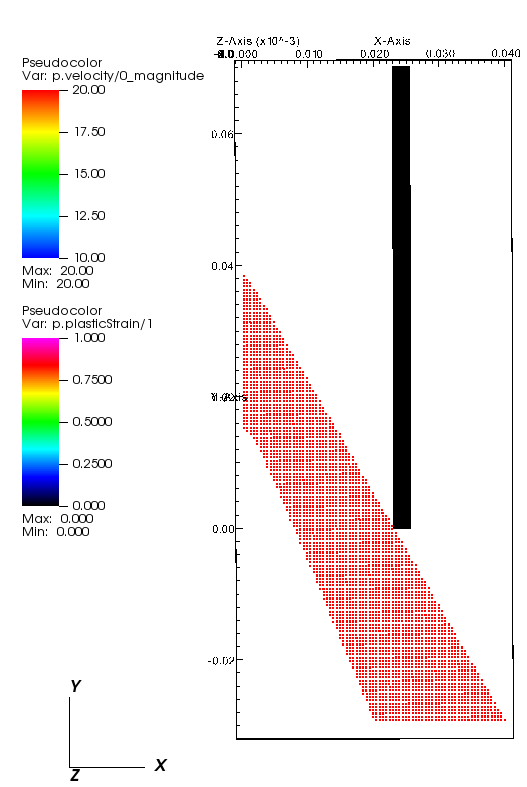
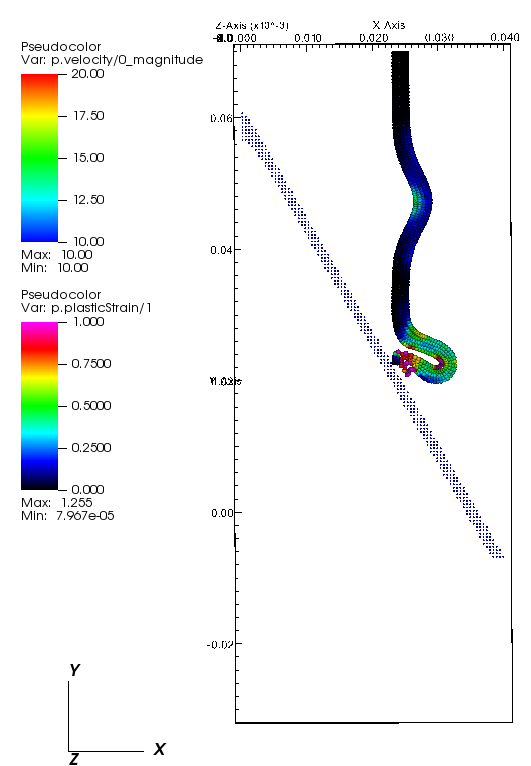
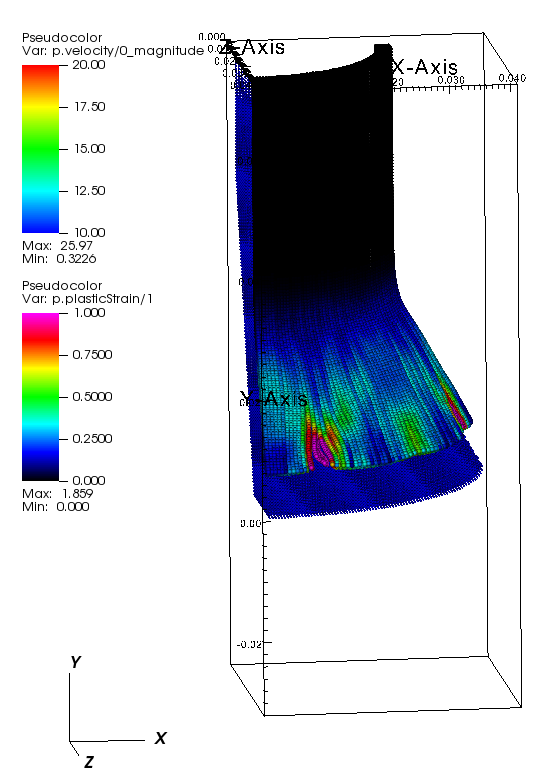
Can anyone point me to a calculation or formula that would tell me what percentage an 6061 T6 aluminum tube OD should be able to be flared to, using any or all of the following: OD size, Wall size, yield strength, ultimate tensile strength and elongation?

I have a 3" long sample that has a 2" outside diameter with a wall size of 0.090". The method of flaring is utilizing an Instron tensile testing machine. The part is being forced down onto a 60 degree cone with a flat surface at the top. There is not a predetermined speed or force that the cross-head must move. The T, Y & E properties fall into the middle of the tolerance for the alloy and temper.

I realize the calculation couldn't be precise, but I'm wanting a ballpark figure to work with as my customers include a minimum flaring percentage that the part must make and I don't know where they're getting their data from. They are coming from steel so I want to make sure they are not just bringing that measurement straight across.

The typical part is anywhere from 2" to 3.5" OD with a wall in a range from .075" to 0.100".. The customer's spec for minimum flare percentage is from 10% to 15% depending on the part.

Improve this question
$\endgroup$
8
  • 1
    $\begingroup$ It depends on more than just that... What temperature/environment are you doing this in? Expanded how gradually, i.e. how extreme is the taper? $\endgroup$
    – Jonathan R Swift
    Commented Sep 20, 2018 at 18:16
  • $\begingroup$ Just to check if we are in the same page, you mean if you pressurise the tube then what would be the ratio of the end OD to the original OD ? $\endgroup$
    – user14407
    Commented Sep 20, 2018 at 18:16
  • $\begingroup$ There are brake pipe flaring tools designed for copper, but only for the end to make a joint - are you talking about the whole length or just the end? $\endgroup$
    – Solar Mike
    Commented Sep 20, 2018 at 19:02
  • $\begingroup$ Hi: you may find much more useful answers if you describe what the end result is you are looking for , rather than how to get there. For example, there might be better ways to produce the "expanded" tubing, or it might be that material other than aluminum is far preferable to meet your system needs. $\endgroup$
    – Carl Witthoft
    Commented Sep 20, 2018 at 19:39
  • $\begingroup$ Add this information to your original question, because it is labelled as to broad and it's at the verge of closing, but it is a good question. $\endgroup$
    – user14407
    Commented Sep 21, 2018 at 7:43

1 Answer 1

Reset to default
1
$\begingroup$

Though there are numerous rules of thumb used by manufacturers, a rigorous approach to finding an optimal amount of flare for a given geometry requires numerical solutions.

The simplest of these solutions will typical use von Mises plasticity (J2-plasticity) in conjunction with a damage model such as Johnson-Cook model.

An axisymmetric model (2D) will give you the plastic strains in the part but will not be able to predict tensile failures around the circumference. A random distribution of initial material parameters is needed to generate strain localization prior to failure.

Often it is easier for the manufacturer to just test a few samples rather than do numerical simulations for which there may not be in-house expertise.

Below, I've attached a few images of what a simulation might produce. Consider the axisymmetric case first. The initial condition is shown below.

If there is a lot of friction between the punch and the cylinder, you may get a buckled part (see below)

If friction is low, you will see flaring. Typically, a plastic strain of 0.25 - 0.5 is enough to cause tensile failure in 6061-T6 Al.

A better estimate can be found by doing a 3D simulation. See the figure below for a feel of what you will see.

There are many studies in the literature on the subject. A few recent ones are:

1) "A theoretical study of the expansion metal tubes" by Liu and Qiu, International Journal of Mechanical Sciences 114 (2016) 157–165.

2) "Towards the characterization of fracture in thin-walled tube forming" by Centeno et al., International Journal of Mechanical Sciences 119 (2016) 12–22.

3) "Numerical and experimental study of axial splitting of circular tubular structures" by Rouzegar and Karimi, Thin-Walled Structures 105 (2016) 57–70.

4) "Expansion and reduction of thin-walled tubes using a die:Experimental and theoretical investigation" by Almeida et al., International Journal of Machine Tools & Manufacture 46 (2006) 1643–1652.

You will get a good idea about the state of the art in this field in these papers.

Improve this answer
$\endgroup$
3
  • $\begingroup$ Thanks Biswajit, I will definitely look up the references you've posted. In my business, we haven't had the extrusion die built before receiving the quote, as dies are $1,000s of dollars, for a part we may not get. So, a challenge we face is that we can't do testing in house prior to needing to decide whether or not to take the business on. This particular customer was one of the first in the US to switch from steel to aluminum. Its been a struggle convincing folks of the limits of this alloy and temper. $\endgroup$
    – Jimmy V
    Commented Sep 24, 2018 at 12:55
  • $\begingroup$ Added to the previous, the customer and the customer's customer will consider the entire lot of material non-conforming if only one sample is found to have a split. We may send thousands of feet of tube, but just one 2" sample split can cause major headaches and returns as they don't deal with a ppm, but a zero-defect policy. $\endgroup$
    – Jimmy V
    Commented Sep 24, 2018 at 13:13
  • $\begingroup$ In that case I'd recommend doing simulations. 6061-T6 is well characterized and you'll be able to find data quite easily. Tensile splits are caused by tiny imperfections in the shape and small variations in material properties. These cannot be avoided if you're working close to the limiting strength of the material. The solution is to work well below that strength, use a slow process (for low strain rates), and make sure that the tube extrusion process does not introduce too much texture in the tubes. $\endgroup$
    – Biswajit Banerjee
    Commented Sep 24, 2018 at 20:53

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.