1
$\begingroup$

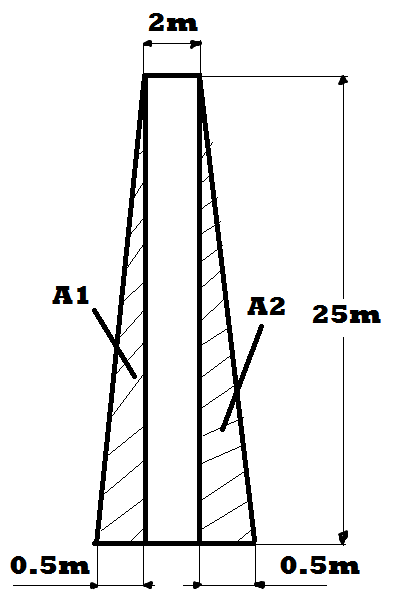
I am trying to solve the following problem:

A masonry chimney having the shape of a conical frustrum is 25 m high. The external diameter at the top and internal diameter at the bottom is 2 m. The chimney is 0.5 m thick at its base. If the weight of the chimney is 1800 kN, find the uniform horizontal wind pressure that may act on the chimney per unit projected area of the chimney in order for tension at the base to be just avoided.

I am using the equation:

$$\mathrm{Centroid} (\bar{y}) = \dfrac {A_{1}y_{1} + A_2y_{2}} {A_{1} + A_{2}}$$

where $A1$ and $A2$ are the shaded areas in the figure below. Observe that the object is a chimney, so it is hollow in the centre portion; we are not adding up the area of that.

Cross-sectional diagram of the chimney with the area on the left marked A1 and the area on the right marked A2

The centroid becomes:

$$ \bar{y} = \dfrac{ (\dfrac{1}2 *0.5 *25) * \dfrac{25}3 + (\dfrac{1}2 *0.5 *25) * \dfrac{25}3}{(\dfrac{1}2 *0.5 *25) +(\dfrac{1}2 *0.5 *25) }$$

$$\bar{y} = 8.333$$

However, I created a 3D model of the solid chimney in CAD and found the centroid to be 8.035, which does not match my calculated centroid. It is close to 8.333 but not exactly the same as my $\bar{y}$.

When I calculate the total wind pressure, which centroid should I use? The one I calculated by hand from the 2D cross-section, or the one I generated in CAD from the 3D model?

The CAD generated centroid is for a 3D solid and $\bar{y}$ calculated is for that central section.

Improve this question
$\endgroup$

1 Answer 1

Reset to default
2
$\begingroup$

I believe that you are confusing yourself by introducing the 3D aspect when building your CAD model, and yet trying to determine the answer by hand using a 2D calculation. Due to the volume being different to an area, this will skew your answer.

I hope this helps nudge you in the right direction

Improve this answer
$\endgroup$
3
  • $\begingroup$ i know that the only difference is due to consideration of 2d shape / 3d solid. My question is in this context which centroid must be considered? my prof. just told me the wind force is acting on projected area and not curved surface area. but the doubt still remains $\endgroup$
    – Fennekin
    Commented Oct 22, 2016 at 15:40
  • $\begingroup$ @Fennekin - Your prof is right. Consider a T section with wind acting perpendicularly to the flange, and on the flange side. What does it matter how big the web is, or whether the web is central or not, in terms of the wind force acting on the section? (As a further note: it's the area project by the external face that is important. I assume the external face doesn't have a hole in the middle? So why does your picture in the question have a hole in the middle?) $\endgroup$
    – AndyT
    Commented Nov 22, 2016 at 10:45
  • $\begingroup$ because I was showing section at middle $\endgroup$
    – Fennekin
    Commented Nov 22, 2016 at 16:04

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.