I'm confused by this. There is a moment of inertia for a shape, let's say circle:
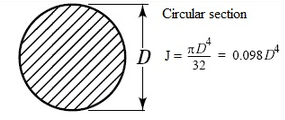
But if you make it 3D, you have a new moment of inertia:
Is there any connection between these two?
$\begingroup$
$\endgroup$
5
-
$\begingroup$ Look up first mpment of innertia and second moment. TBH english is a bit stupid in this regard $\endgroup$– joojaaCommented Feb 16, 2020 at 19:29
-
$\begingroup$ @joojaa other names for those two are area moment of inertia and mass moment of inertia (those can help a little to differentiate the two). $\endgroup$– fibonaticCommented Feb 16, 2020 at 19:54
-
$\begingroup$ @fibonatic yeah but still both called inertia. Theres no need to do that you know. Not all languages do. $\endgroup$– joojaaCommented Feb 16, 2020 at 19:59
-
$\begingroup$ Please get in the habit of calling the first type the second moment of area. The sanity you save may be your own. And have an upvote to mitigate the driveby downvote. I reckon I've seen tables labeled "inertia" that had ten different dimensional units attached. It's crazy. But each industry seems to have had its own canonical way of cookbooking inertial properties. Just make sure you know exactly how any tabulated inertial values are intended to be used. $\endgroup$– Phil SweetCommented Feb 17, 2020 at 2:04
-
1$\begingroup$ The connection is that in the second case M is = density * L * pi * r^2. So inertia = 1/2 * density * L * pi * r^4, or 1/2 * density * L * pi * d^4 / 16. They have different dimensional units but they scale similarly with r. $\endgroup$– Phil SweetCommented Feb 17, 2020 at 2:18
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
The general idea in both cases is similar.
In the first case, we are basically summing the differential areas multiplied by the square of their distance from the axis of rotation, neutral axis. This is tightly related to the concept of the linear relationship of stress and strain in the flexural properties of beams and Young's modulus. This is usually called the second area moment or second-moment of inertia.
$$ I_x=\int y^2dA $$
In the second case, as shown in your second diagram, We are summing the differential mass elements of a solid (doesn't have to be a solid, liquid, gas or plasma is fine) again multiplied by the square of their distances from the axis of rotation, to get the inertia for angular momentum and the concepts of rotational energy, work, and preservation of angular momentum and the entire spectrum of related physics. $$ I=\int r^2dm$$
