First of all a bit of background, the Poisson ratio is calculated from
$$v=-\frac{\epsilon_y}{\epsilon_x}$$
To use the above equation, you need to use a uniaxial loading (axial or compressive). Because at different loads you might have different Poisson ratio usually what happens is that in the elastic region you create a plot of $-\epsilon_y$ vs. $\epsilon_x$ and you can use regression analysis to estimate the coefficient.
Regarding the example you present, I presume that one case of a hollow box with a changing thickness has the following shape.

In this example there are two things that are important:
- The Poisson Ratio in the literature can be found as a material or structure property.
The former case (material property), is the most traditional and is measured with the above mentioned formula as $-\frac{\epsilon_y}{\epsilon_x}$ for a uniaxial stress state. In that case, you are getting the averaged property of an infinitesimal cube of the material. (Because each piece of the material is assumed to be in the same state is should exhibit similar behaviour, thus averaging). Essentially, its the same as stacking 1000 pieces of A4 paper in order to estimate the thickness of one sheet of A4 paper with a hand held measure).
The latter case (structure property) is most commonly used for auxetic structures. Sometimes these are referenced as auxetic materials. Again what you are measuring is an averaged property of the structure (the material behaviour is a totally different thing). As such you should have a repeated pattern across the structure.
Obviously, the stresses and strains on a small cube of material at different points of the structure differ significantly, and only in a macroscopic and (very loose) sense can be viewed as stresses and strains. In that sense the Poisson ratio is applied to this case of "materials".
- Loading axially a structure with changing cross-section (exception is if its symmetric), introduces shear stresses.
Imagine cutting image 1, in for pieces (resulting in a trapezoidal-ish shape). If you apply a force on that geometry, then the material will also shear.
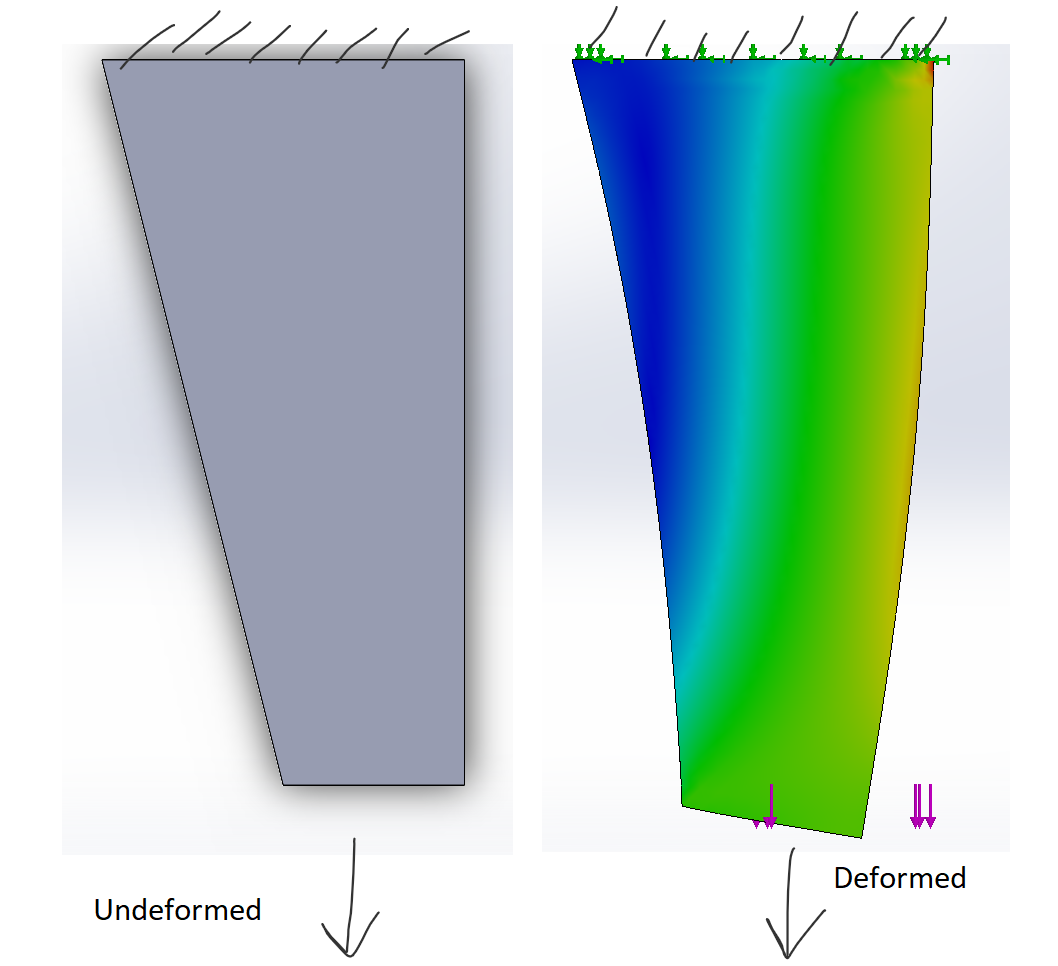
As a result, you can't use the Poisson formula for this scenario. Not unless you have a repeating structure (like a honeycomb or a auxetic structure).

