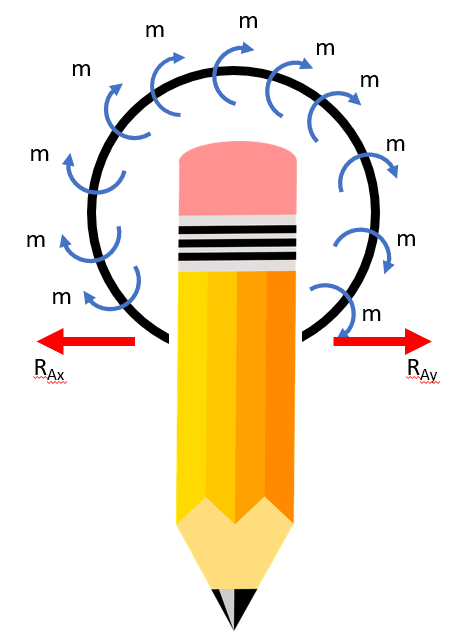
I have some Shape Memory Alloy wire programmed to curl from a straight beam into a circle. The force this generates is approximated as a uniform distributed bending moment along the length of the wire. As the ends of the wire approach to close the circle, I put a pencil in between so that the bending wire "pinches" and grips the pencil. What equations could I use to predict the "pinching (gripping)" force assuming I know the magnitude of the distributed moment? I'm imagining starting from a two-hinged arch and approximating the distributed moment as a concentrated moment at the center of the beam, but am struggling to find example equations that would fit, and I'm really rusty on my free body diagrams.
I did find a similar approach for a three-hinged arch, but I'm not sure those equations apply.
I'm also aware that the bending moment shrinks as the wire approaches its maximum (no load) curled deflection, but I'm OK assuming it's constant at all deflections for now.
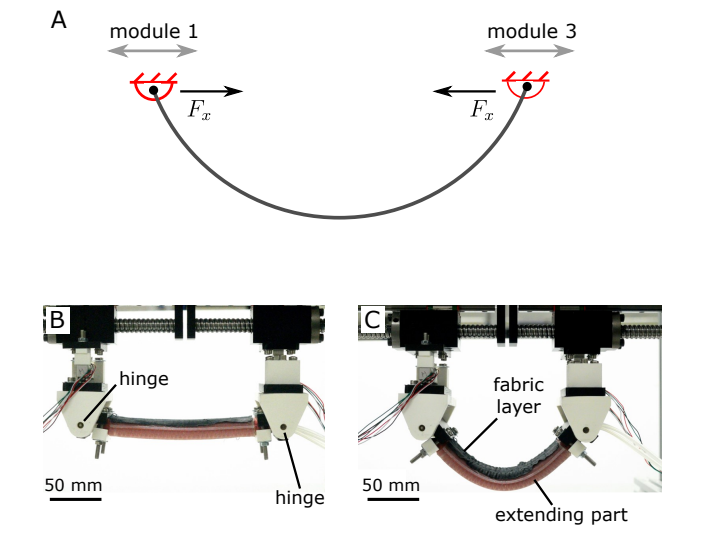
Here's an image of an actuator I found in a paper that is doing the same type of motion by inflating a balloon which causes this beam to bend. They don't provide any equations though; just raw force measurements on some lab equipment.
EDIT: Here's my attempt at a FBD, but I know I'm missing things I'm not sure on.: